
Question Number 151495 by Tawa11 last updated on 21/Aug/21
Commented by Tawa11 last updated on 21/Aug/21

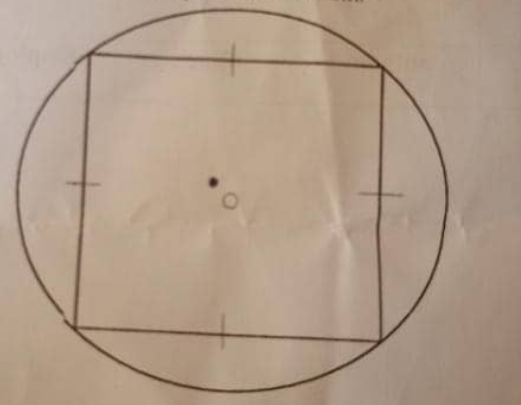
$$\mathrm{If}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{square}\:\mathrm{is}\:\:\:\mathrm{144m}^{\mathrm{2}} .\:\:\mathrm{Find}\:\mathrm{the}\:\mathrm{radius}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circle}. \\ $$$$\mathrm{I}\:\mathrm{got}\:\:\:\:\:\mathrm{6}\sqrt{\mathrm{2}}\:,\:\:\:\:\mathrm{is}\:\mathrm{it}\:\mathrm{correct}? \\ $$
Commented by talminator2856791 last updated on 21/Aug/21

$$\:\mathrm{6}\sqrt{\mathrm{2}} \\ $$
Commented by puissant last updated on 21/Aug/21
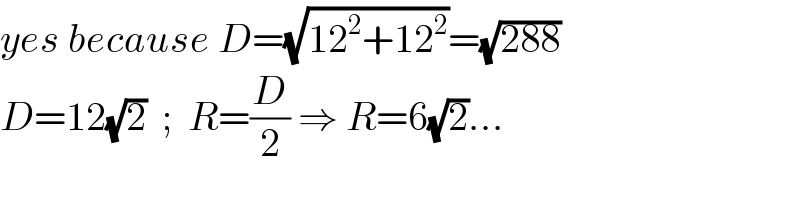
$${yes}\:{because}\:{D}=\sqrt{\mathrm{12}^{\mathrm{2}} +\mathrm{12}^{\mathrm{2}} }=\sqrt{\mathrm{288}} \\ $$$${D}=\mathrm{12}\sqrt{\mathrm{2}}\:\:;\:\:{R}=\frac{{D}}{\mathrm{2}}\:\Rightarrow\:{R}=\mathrm{6}\sqrt{\mathrm{2}}... \\ $$
Commented by Tawa11 last updated on 21/Aug/21
$$\mathrm{Thanks}\:\mathrm{sir}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}. \\ $$
