Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 147768 by aliibrahim1 last updated on 23/Jul/21

Answered by liberty last updated on 23/Jul/21

Let BC = x ⇒tan 44°=((CD)/x) ⇒CD=x tan 44° Let AC =y⇒tan 48°=((CD)/y) ⇒CD=y tan 48° where y=(√(30^2 −x^2 )) =(√(900−x^2 )) (•)CD=CD ⇒x tan 44°=(√(900−x^2 )) tan 48° ⇒x^2 tan^2 44°=(900−x^2 )tan^2 48° ⇒x^2 (tan^2 44°+tan^2 48°)=900×tan^2 48° ⇒x = (√((900×tan^2 48°)/(tan^2 44°+tan^2 48°))) ≈22.64 ∴ CD=22.64×tan 44°≈ 21.87

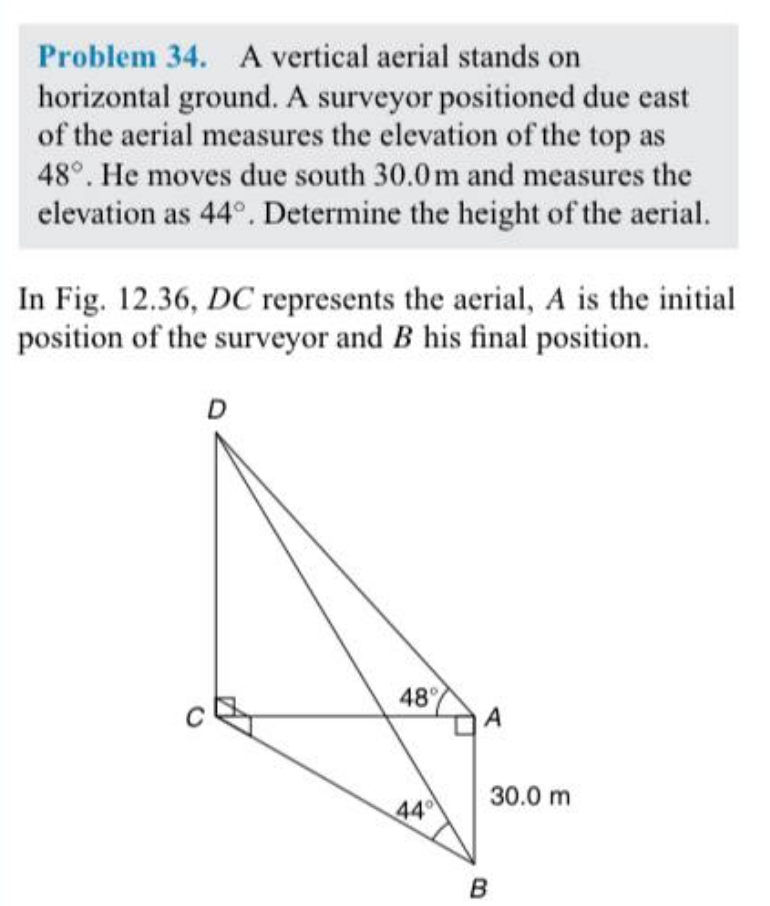
$${Let}\:{BC}\:=\:{x}\:\Rightarrow\mathrm{tan}\:\mathrm{44}°=\frac{{CD}}{{x}} \\ $$$$\Rightarrow{CD}={x}\:\mathrm{tan}\:\mathrm{44}° \\ $$$$\mathrm{L}{et}\:{AC}\:={y}\Rightarrow\mathrm{tan}\:\mathrm{48}°=\frac{{CD}}{{y}} \\ $$$$\Rightarrow{CD}={y}\:\mathrm{tan}\:\mathrm{48}° \\ $$$${where}\:{y}=\sqrt{\mathrm{30}^{\mathrm{2}} −{x}^{\mathrm{2}} }\:=\sqrt{\mathrm{900}−{x}^{\mathrm{2}} } \\ $$$$\left(\bullet\right){CD}={CD} \\ $$$$\Rightarrow{x}\:\mathrm{tan}\:\mathrm{44}°=\sqrt{\mathrm{900}−{x}^{\mathrm{2}} }\:\mathrm{tan}\:\mathrm{48}° \\ $$$$\Rightarrow{x}^{\mathrm{2}} \:\mathrm{tan}\:^{\mathrm{2}} \mathrm{44}°=\left(\mathrm{900}−{x}^{\mathrm{2}} \right)\mathrm{tan}\:^{\mathrm{2}} \mathrm{48}° \\ $$$$\Rightarrow{x}^{\mathrm{2}} \left(\mathrm{tan}\:^{\mathrm{2}} \mathrm{44}°+\mathrm{tan}^{\mathrm{2}} \:\mathrm{48}°\right)=\mathrm{900}×\mathrm{tan}\:^{\mathrm{2}} \mathrm{48}° \\ $$$$\Rightarrow{x}\:=\:\sqrt{\frac{\mathrm{900}×\mathrm{tan}^{\mathrm{2}} \:\mathrm{48}°}{\mathrm{tan}\:^{\mathrm{2}} \mathrm{44}°+\mathrm{tan}\:^{\mathrm{2}} \mathrm{48}°}}\:\approx\mathrm{22}.\mathrm{64} \\ $$$$\therefore\:{CD}=\mathrm{22}.\mathrm{64}×\mathrm{tan}\:\mathrm{44}°\approx\:\mathrm{21}.\mathrm{87} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com