
Question Number 131199 by aurpeyz last updated on 02/Feb/21

Answered by physicstutes last updated on 02/Feb/21
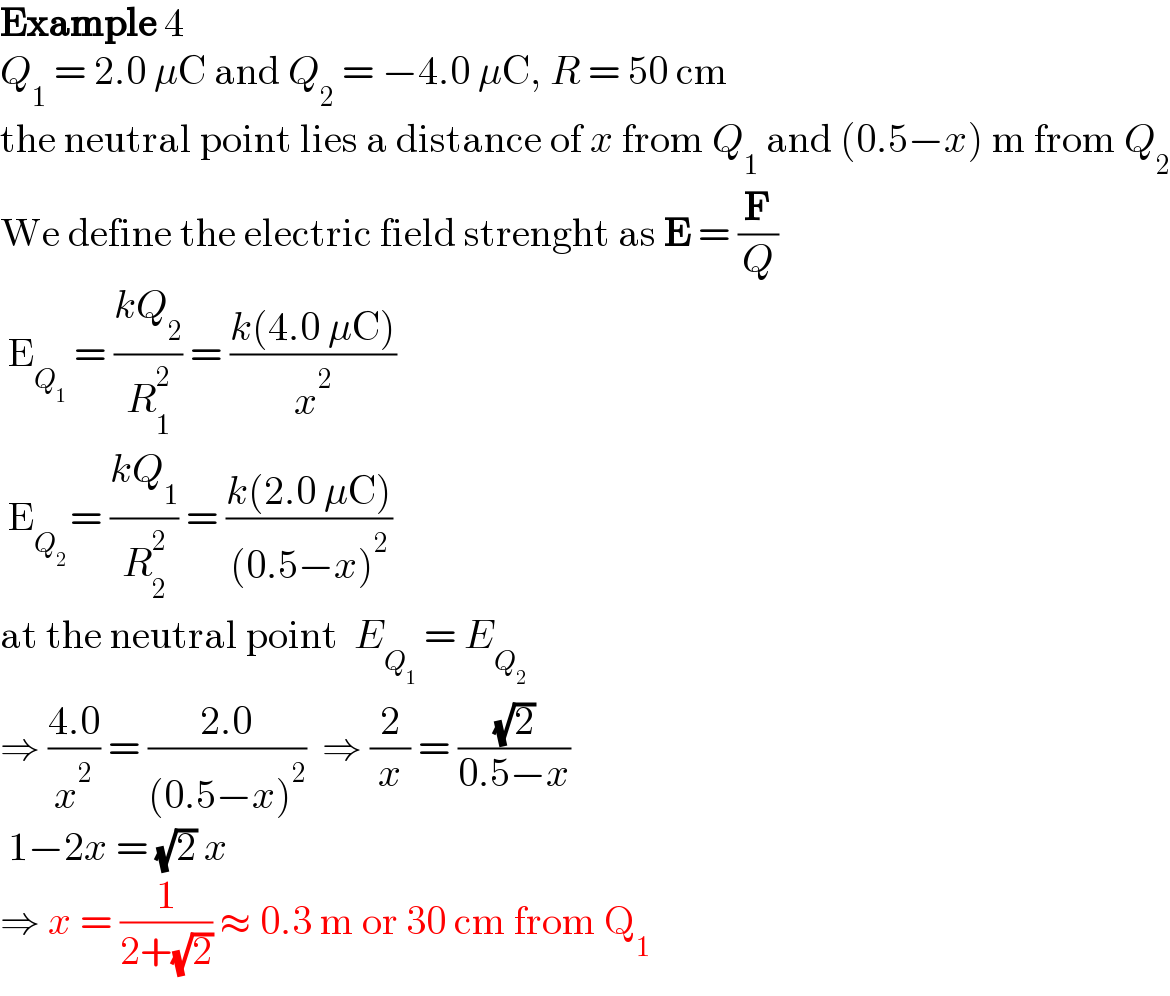
$$\boldsymbol{\mathrm{Example}}\:\mathrm{4} \\ $$$${Q}_{\mathrm{1}} \:=\:\mathrm{2}.\mathrm{0}\:\mu\mathrm{C}\:\mathrm{and}\:{Q}_{\mathrm{2}} \:=\:−\mathrm{4}.\mathrm{0}\:\mu\mathrm{C},\:{R}\:=\:\mathrm{50}\:\mathrm{cm} \\ $$$$\mathrm{the}\:\mathrm{neutral}\:\mathrm{point}\:\mathrm{lies}\:\mathrm{a}\:\mathrm{distance}\:\mathrm{of}\:{x}\:\mathrm{from}\:{Q}_{\mathrm{1}} \:\mathrm{and}\:\left(\mathrm{0}.\mathrm{5}−{x}\right)\:\mathrm{m}\:\mathrm{from}\:{Q}_{\mathrm{2}} \\ $$$$\mathrm{We}\:\mathrm{define}\:\mathrm{the}\:\mathrm{electric}\:\mathrm{field}\:\mathrm{strenght}\:\mathrm{as}\:\boldsymbol{\mathrm{E}}\:=\:\frac{\boldsymbol{\mathrm{F}}}{{Q}} \\ $$$$\:\mathrm{E}_{{Q}_{\mathrm{1}} } \:=\:\frac{{kQ}_{\mathrm{2}} }{{R}_{\mathrm{1}} ^{\mathrm{2}} }\:=\:\frac{{k}\left(\mathrm{4}.\mathrm{0}\:\mu\mathrm{C}\right)}{{x}^{\mathrm{2}} } \\ $$$$\:\mathrm{E}_{{Q}_{\mathrm{2}} \:} =\:\frac{{kQ}_{\mathrm{1}} }{{R}_{\mathrm{2}} ^{\mathrm{2}} }\:=\:\frac{{k}\left(\mathrm{2}.\mathrm{0}\:\mu\mathrm{C}\right)}{\left(\mathrm{0}.\mathrm{5}−{x}\right)^{\mathrm{2}} } \\ $$$$\mathrm{at}\:\mathrm{the}\:\mathrm{neutral}\:\mathrm{point}\:\:{E}_{{Q}_{\mathrm{1}} } \:=\:{E}_{{Q}_{\mathrm{2}} } \\ $$$$\Rightarrow\:\frac{\mathrm{4}.\mathrm{0}}{{x}^{\mathrm{2}} }\:=\:\frac{\mathrm{2}.\mathrm{0}}{\left(\mathrm{0}.\mathrm{5}−{x}\right)^{\mathrm{2}} }\:\:\Rightarrow\:\frac{\mathrm{2}}{{x}}\:=\:\frac{\sqrt{\mathrm{2}}}{\mathrm{0}.\mathrm{5}−{x}} \\ $$$$\:\mathrm{1}−\mathrm{2}{x}\:=\:\sqrt{\mathrm{2}}\:{x} \\ $$$$\Rightarrow\:{x}\:=\:\frac{\mathrm{1}}{\mathrm{2}+\sqrt{\mathrm{2}}}\:\approx\:\mathrm{0}.\mathrm{3}\:\mathrm{m}\:\mathrm{or}\:\mathrm{30}\:\mathrm{cm}\:\mathrm{from}\:\mathrm{Q}_{\mathrm{1}} \\ $$
Commented by aurpeyz last updated on 06/Feb/21
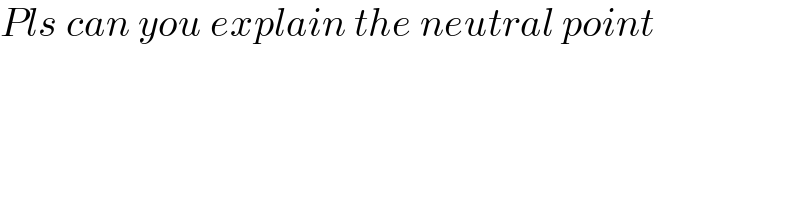
$${Pls}\:{can}\:{you}\:{explain}\:{the}\:{neutral}\:{point} \\ $$$$ \\ $$
Commented by physicstutes last updated on 06/Feb/21

$$\mathrm{point}\:\mathrm{were}\:{E}_{{net}} \:=\:\mathrm{0} \\ $$
