Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 129951 by Algoritm last updated on 21/Jan/21

Commented by MJS_new last updated on 21/Jan/21

2^(x/3) −2^x =2 t=2^(x/3) t−t^3 =2 t≈−1.52∨t≈.761±.858i 2^(2x/3) +2^(4x/3) +4^x =5 t=2^(x/3) t^6 +t^4 +t^2 =5 t≈±1.13∨t≈±(.648±1.25i) 2^x −8^x =10 (?) t=2^(x/3) t^3 −t^9 =10 t≈−1.32∨t≈−.960±.841∨t≈−.249±1.25∨t≈.661±1.14 ⇒ no solution at all

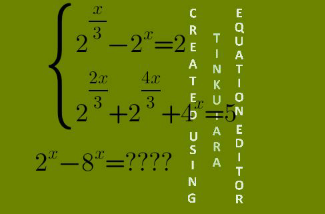
$$\mathrm{2}^{{x}/\mathrm{3}} −\mathrm{2}^{{x}} =\mathrm{2} \\ $$$${t}=\mathrm{2}^{{x}/\mathrm{3}} \\ $$$${t}−{t}^{\mathrm{3}} =\mathrm{2} \\ $$$${t}\approx−\mathrm{1}.\mathrm{52}\vee{t}\approx.\mathrm{761}\pm.\mathrm{858i} \\ $$$$ \\ $$$$\mathrm{2}^{\mathrm{2}{x}/\mathrm{3}} +\mathrm{2}^{\mathrm{4}{x}/\mathrm{3}} +\mathrm{4}^{{x}} =\mathrm{5} \\ $$$${t}=\mathrm{2}^{{x}/\mathrm{3}} \\ $$$${t}^{\mathrm{6}} +{t}^{\mathrm{4}} +{t}^{\mathrm{2}} =\mathrm{5} \\ $$$${t}\approx\pm\mathrm{1}.\mathrm{13}\vee{t}\approx\pm\left(.\mathrm{648}\pm\mathrm{1}.\mathrm{25i}\right) \\ $$$$ \\ $$$$\mathrm{2}^{{x}} −\mathrm{8}^{{x}} =\mathrm{10}\:\left(?\right) \\ $$$${t}=\mathrm{2}^{{x}/\mathrm{3}} \\ $$$${t}^{\mathrm{3}} −{t}^{\mathrm{9}} =\mathrm{10} \\ $$$${t}\approx−\mathrm{1}.\mathrm{32}\vee{t}\approx−.\mathrm{960}\pm.\mathrm{841}\vee{t}\approx−.\mathrm{249}\pm\mathrm{1}.\mathrm{25}\vee{t}\approx.\mathrm{661}\pm\mathrm{1}.\mathrm{14} \\ $$$$ \\ $$$$\Rightarrow\:\mathrm{no}\:\mathrm{solution}\:\mathrm{at}\:\mathrm{all} \\ $$

Answered by SEKRET last updated on 21/Jan/21

10

$$\mathrm{10} \\ $$

Answered by SEKRET last updated on 21/Jan/21

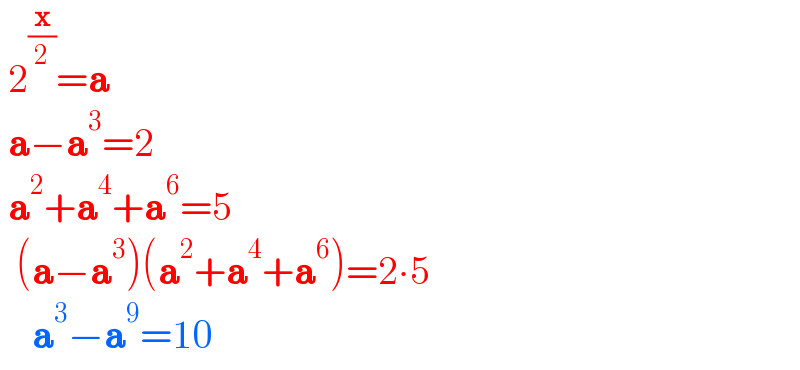
2^(x/2) =a a−a^3 =2 a^2 +a^4 +a^6 =5 (a−a^3 )(a^2 +a^4 +a^6 )=2∙5 a^3 −a^9 =10

$$\:\mathrm{2}^{\frac{\boldsymbol{\mathrm{x}}}{\mathrm{2}}} =\boldsymbol{\mathrm{a}} \\ $$$$\:\boldsymbol{\mathrm{a}}−\boldsymbol{\mathrm{a}}^{\mathrm{3}} =\mathrm{2} \\ $$$$\:\boldsymbol{\mathrm{a}}^{\mathrm{2}} +\boldsymbol{\mathrm{a}}^{\mathrm{4}} +\boldsymbol{\mathrm{a}}^{\mathrm{6}} =\mathrm{5} \\ $$$$\:\:\left(\boldsymbol{\mathrm{a}}−\boldsymbol{\mathrm{a}}^{\mathrm{3}} \right)\left(\boldsymbol{\mathrm{a}}^{\mathrm{2}} +\boldsymbol{\mathrm{a}}^{\mathrm{4}} +\boldsymbol{\mathrm{a}}^{\mathrm{6}} \right)=\mathrm{2}\centerdot\mathrm{5} \\ $$$$\:\:\:\:\boldsymbol{\mathrm{a}}^{\mathrm{3}} −\boldsymbol{\mathrm{a}}^{\mathrm{9}} =\mathrm{10} \\ $$

Commented by MJS_new last updated on 21/Jan/21

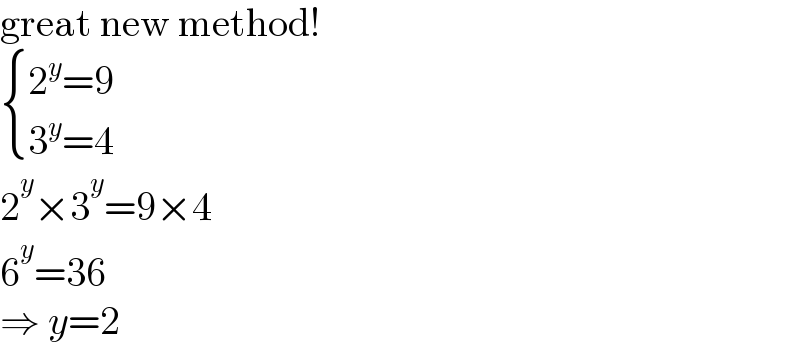
great new method! { ((2^y =9)),((3^y =4)) :} 2^y ×3^y =9×4 6^y =36 ⇒ y=2

$$\mathrm{great}\:\mathrm{new}\:\mathrm{method}! \\ $$$$\begin{cases}{\mathrm{2}^{{y}} =\mathrm{9}}\\{\mathrm{3}^{{y}} =\mathrm{4}}\end{cases} \\ $$$$\mathrm{2}^{{y}} ×\mathrm{3}^{{y}} =\mathrm{9}×\mathrm{4} \\ $$$$\mathrm{6}^{{y}} =\mathrm{36} \\ $$$$\Rightarrow\:{y}=\mathrm{2} \\ $$

Answered by bemath last updated on 21/Jan/21

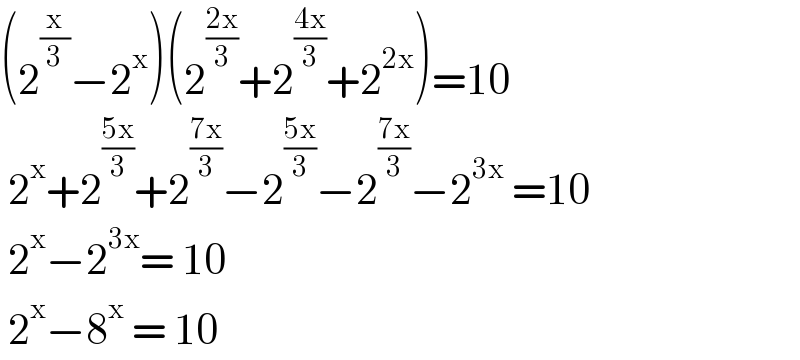
(2^(x/3) −2^x )(2^((2x)/3) +2^((4x)/3) +2^(2x) )=10 2^x +2^((5x)/3) +2^((7x)/3) −2^((5x)/3) −2^((7x)/3) −2^(3x) =10 2^x −2^(3x) = 10 2^x −8^x = 10

$$\left(\mathrm{2}^{\frac{\mathrm{x}}{\mathrm{3}}} −\mathrm{2}^{\mathrm{x}} \right)\left(\mathrm{2}^{\frac{\mathrm{2x}}{\mathrm{3}}} +\mathrm{2}^{\frac{\mathrm{4x}}{\mathrm{3}}} +\mathrm{2}^{\mathrm{2x}} \right)=\mathrm{10} \\ $$$$\:\mathrm{2}^{\mathrm{x}} +\mathrm{2}^{\frac{\mathrm{5x}}{\mathrm{3}}} +\mathrm{2}^{\frac{\mathrm{7x}}{\mathrm{3}}} −\mathrm{2}^{\frac{\mathrm{5x}}{\mathrm{3}}} −\mathrm{2}^{\frac{\mathrm{7x}}{\mathrm{3}}} −\mathrm{2}^{\mathrm{3x}} \:=\mathrm{10} \\ $$$$\:\mathrm{2}^{\mathrm{x}} −\mathrm{2}^{\mathrm{3x}} =\:\mathrm{10} \\ $$$$\:\mathrm{2}^{\mathrm{x}} −\mathrm{8}^{\mathrm{x}} \:=\:\mathrm{10} \\ $$

Commented by MJS_new last updated on 21/Jan/21

people, this is wrong! there′s no x solving both equations as I showed. multiplying two equations is not allowed in case the variables are not the same { ((x=5)),((x=3)) :} ⇒^? x^2 =15

$$\mathrm{people},\:\mathrm{this}\:\mathrm{is}\:\mathrm{wrong}!\:\mathrm{there}'\mathrm{s}\:\mathrm{no}\:{x}\:\mathrm{solving} \\ $$$$\mathrm{both}\:\mathrm{equations}\:\mathrm{as}\:\mathrm{I}\:\mathrm{showed}.\:\mathrm{multiplying} \\ $$$$\mathrm{two}\:\mathrm{equations}\:\mathrm{is}\:\mathrm{not}\:\mathrm{allowed}\:\mathrm{in}\:\mathrm{case}\:\mathrm{the} \\ $$$$\mathrm{variables}\:\mathrm{are}\:\mathrm{not}\:\mathrm{the}\:\mathrm{same} \\ $$$$\begin{cases}{{x}=\mathrm{5}}\\{{x}=\mathrm{3}}\end{cases} \\ $$$$\overset{?} {\Rightarrow}\:{x}^{\mathrm{2}} =\mathrm{15} \\ $$

Commented by liberty last updated on 21/Jan/21

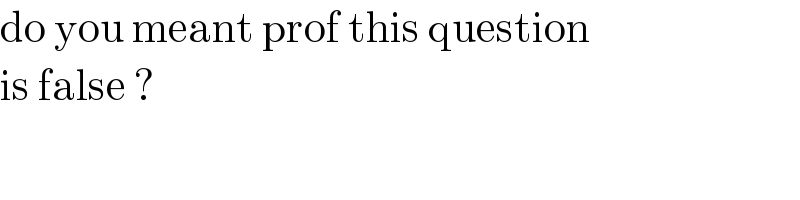
do you meant prof this question is false ?

$$\mathrm{do}\:\mathrm{you}\:\mathrm{meant}\:\mathrm{prof}\:\mathrm{this}\:\mathrm{question} \\ $$$$\mathrm{is}\:\mathrm{false}\:? \\ $$

Commented by MJS_new last updated on 21/Jan/21

obviously

$$\mathrm{obviously} \\ $$

Commented by liberty last updated on 21/Jan/21

yes. i agree

$$\mathrm{yes}.\:\mathrm{i}\:\mathrm{agree} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com