Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 127460 by ajfour last updated on 30/Dec/20

Commented by ajfour last updated on 30/Dec/20

Q.127127 (Revisit) Given p,q ; find R.

$${Q}.\mathrm{127127}\:\:\:\left({Revisit}\right) \\ $$$${Given}\:\:{p},{q}\:\:\:;\:\:{find}\:{R}. \\ $$

Answered by ajfour last updated on 30/Dec/20

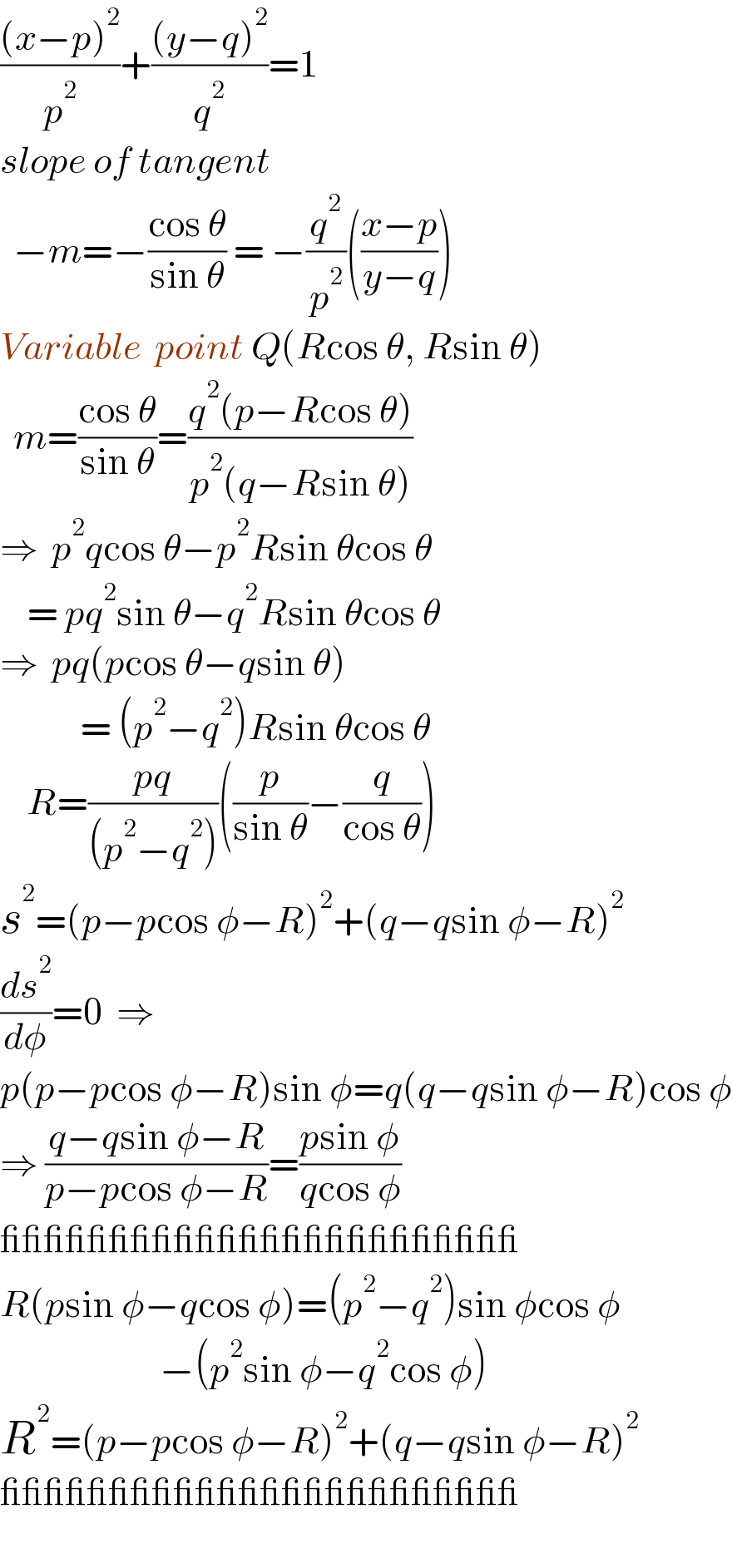
(((x−p)^2 )/p^2 )+(((y−q)^2 )/q^2 )=1 slope of tangent −m=−((cos θ)/(sin θ)) = −(q^2 /p^2 )(((x−p)/(y−q))) Variable point Q(Rcos θ, Rsin θ) m=((cos θ)/(sin θ))=((q^2 (p−Rcos θ))/(p^2 (q−Rsin θ))) ⇒ p^2 qcos θ−p^2 Rsin θcos θ = pq^2 sin θ−q^2 Rsin θcos θ ⇒ pq(pcos θ−qsin θ) = (p^2 −q^2 )Rsin θcos θ R=((pq)/((p^2 −q^2 )))((p/(sin θ))−(q/(cos θ))) s^2 =(p−pcos φ−R)^2 +(q−qsin φ−R)^2 (ds^2 /dφ)=0 ⇒ p(p−pcos φ−R)sin φ=q(q−qsin φ−R)cos φ ⇒ ((q−qsin φ−R)/(p−pcos φ−R))=((psin φ)/(qcos φ)) ________________________ R(psin φ−qcos φ)=(p^2 −q^2 )sin φcos φ −(p^2 sin φ−q^2 cos φ) R^2 =(p−pcos φ−R)^2 +(q−qsin φ−R)^2 ________________________

$$\frac{\left({x}−{p}\right)^{\mathrm{2}} }{{p}^{\mathrm{2}} }+\frac{\left({y}−{q}\right)^{\mathrm{2}} }{{q}^{\mathrm{2}} }=\mathrm{1} \\ $$$${slope}\:{of}\:{tangent} \\ $$$$\:\:−{m}=−\frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}\:=\:−\frac{{q}^{\mathrm{2}} }{{p}^{\mathrm{2}} }\left(\frac{{x}−{p}}{{y}−{q}}\right) \\ $$$${Variable}\:\:{point}\:{Q}\left({R}\mathrm{cos}\:\theta,\:{R}\mathrm{sin}\:\theta\right) \\ $$$$\:\:{m}=\frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}=\frac{{q}^{\mathrm{2}} \left({p}−{R}\mathrm{cos}\:\theta\right)}{{p}^{\mathrm{2}} \left({q}−{R}\mathrm{sin}\:\theta\right)} \\ $$$$\Rightarrow\:\:{p}^{\mathrm{2}} {q}\mathrm{cos}\:\theta−{p}^{\mathrm{2}} {R}\mathrm{sin}\:\theta\mathrm{cos}\:\theta \\ $$$$\:\:\:\:=\:{pq}^{\mathrm{2}} \mathrm{sin}\:\theta−{q}^{\mathrm{2}} {R}\mathrm{sin}\:\theta\mathrm{cos}\:\theta \\ $$$$\Rightarrow\:\:{pq}\left({p}\mathrm{cos}\:\theta−{q}\mathrm{sin}\:\theta\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:=\:\left({p}^{\mathrm{2}} −{q}^{\mathrm{2}} \right){R}\mathrm{sin}\:\theta\mathrm{cos}\:\theta \\ $$$$\:\:\:\:{R}=\frac{{pq}}{\left({p}^{\mathrm{2}} −{q}^{\mathrm{2}} \right)}\left(\frac{{p}}{\mathrm{sin}\:\theta}−\frac{{q}}{\mathrm{cos}\:\theta}\right) \\ $$$${s}^{\mathrm{2}} =\left({p}−{p}\mathrm{cos}\:\phi−{R}\right)^{\mathrm{2}} +\left({q}−{q}\mathrm{sin}\:\phi−{R}\right)^{\mathrm{2}} \\ $$$$\frac{{ds}^{\mathrm{2}} }{{d}\phi}=\mathrm{0}\:\:\Rightarrow \\ $$$${p}\left({p}−{p}\mathrm{cos}\:\phi−{R}\right)\mathrm{sin}\:\phi={q}\left({q}−{q}\mathrm{sin}\:\phi−{R}\right)\mathrm{cos}\:\phi \\ $$$$\Rightarrow\:\frac{{q}−{q}\mathrm{sin}\:\phi−{R}}{{p}−{p}\mathrm{cos}\:\phi−{R}}=\frac{{p}\mathrm{sin}\:\phi}{{q}\mathrm{cos}\:\phi} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$${R}\left({p}\mathrm{sin}\:\phi−{q}\mathrm{cos}\:\phi\right)=\left({p}^{\mathrm{2}} −{q}^{\mathrm{2}} \right)\mathrm{sin}\:\phi\mathrm{cos}\:\phi \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\left({p}^{\mathrm{2}} \mathrm{sin}\:\phi−{q}^{\mathrm{2}} \mathrm{cos}\:\phi\right) \\ $$$${R}^{\mathrm{2}} =\left({p}−{p}\mathrm{cos}\:\phi−{R}\right)^{\mathrm{2}} +\left({q}−{q}\mathrm{sin}\:\phi−{R}\right)^{\mathrm{2}} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$$ \\ $$

Answered by mr W last updated on 30/Dec/20

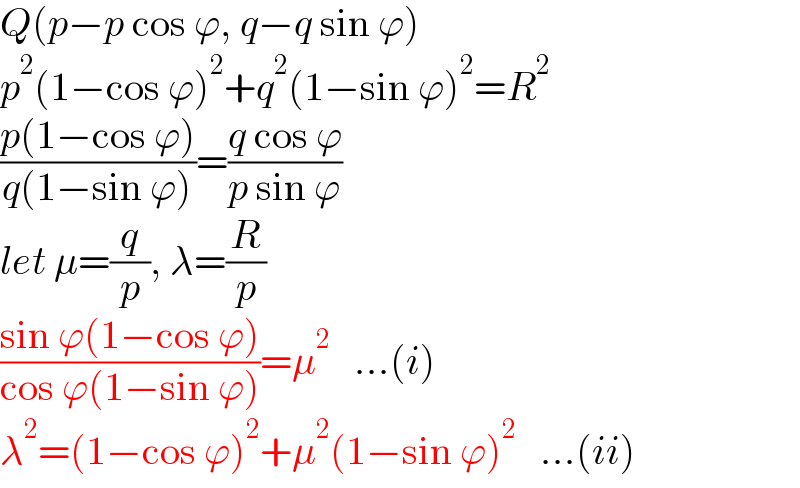
Q(p−p cos ϕ, q−q sin ϕ) p^2 (1−cos ϕ)^2 +q^2 (1−sin ϕ)^2 =R^2 ((p(1−cos ϕ))/(q(1−sin ϕ)))=((q cos ϕ)/(p sin ϕ)) let μ=(q/p), λ=(R/p) ((sin ϕ(1−cos ϕ))/(cos ϕ(1−sin ϕ)))=μ^2 ...(i) λ^2 =(1−cos ϕ)^2 +μ^2 (1−sin ϕ)^2 ...(ii)

$${Q}\left({p}−{p}\:\mathrm{cos}\:\varphi,\:{q}−{q}\:\mathrm{sin}\:\varphi\right) \\ $$$${p}^{\mathrm{2}} \left(\mathrm{1}−\mathrm{cos}\:\varphi\right)^{\mathrm{2}} +{q}^{\mathrm{2}} \left(\mathrm{1}−\mathrm{sin}\:\varphi\right)^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$$\frac{{p}\left(\mathrm{1}−\mathrm{cos}\:\varphi\right)}{{q}\left(\mathrm{1}−\mathrm{sin}\:\varphi\right)}=\frac{{q}\:\mathrm{cos}\:\varphi}{{p}\:\mathrm{sin}\:\varphi} \\ $$$${let}\:\mu=\frac{{q}}{{p}},\:\lambda=\frac{{R}}{{p}} \\ $$$$\frac{\mathrm{sin}\:\varphi\left(\mathrm{1}−\mathrm{cos}\:\varphi\right)}{\mathrm{cos}\:\varphi\left(\mathrm{1}−\mathrm{sin}\:\varphi\right)}=\mu^{\mathrm{2}} \:\:\:...\left({i}\right) \\ $$$$\lambda^{\mathrm{2}} =\left(\mathrm{1}−\mathrm{cos}\:\varphi\right)^{\mathrm{2}} +\mu^{\mathrm{2}} \left(\mathrm{1}−\mathrm{sin}\:\varphi\right)^{\mathrm{2}} \:\:\:...\left({ii}\right) \\ $$

Commented by mr W last updated on 30/Dec/20

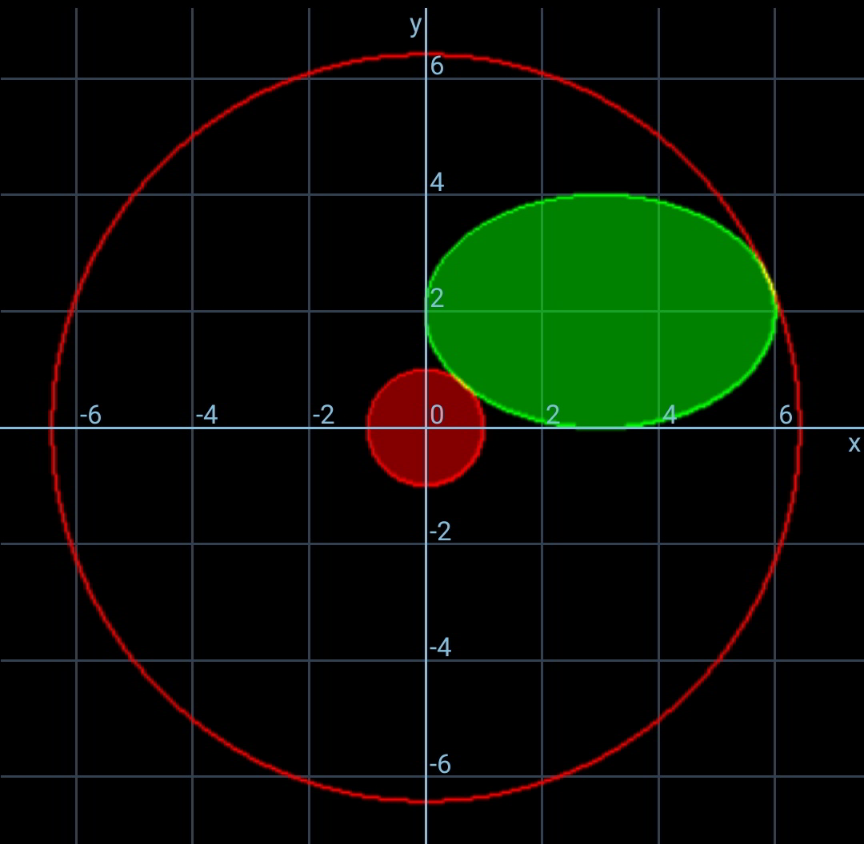
Commented by mr W last updated on 30/Dec/20

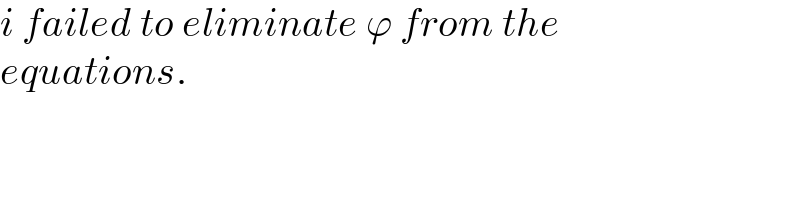
i failed to eliminate ϕ from the equations.

$${i}\:{failed}\:{to}\:{eliminate}\:\varphi\:{from}\:{the} \\ $$$${equations}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com