
Question Number 124953 by Algoritm last updated on 07/Dec/20
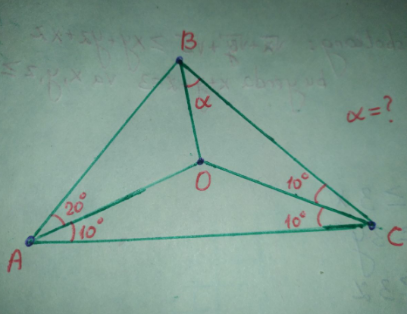
Answered by mr W last updated on 07/Dec/20

$${say}\:{AC}=\mathrm{1} \\ $$$$\frac{{BC}}{\mathrm{sin}\:\mathrm{30}}=\frac{{AC}}{\mathrm{sin}\:\mathrm{50}} \\ $$$$\Rightarrow{BC}=\frac{\mathrm{sin}\:\mathrm{30}}{\mathrm{sin}\:\mathrm{50}} \\ $$$${similarly} \\ $$$${OC}=\frac{\mathrm{sin}\:\mathrm{10}}{\mathrm{sin}\:\mathrm{20}} \\ $$$$\frac{\mathrm{sin}\:\left(\alpha+\mathrm{10}\right)}{\mathrm{sin}\:\alpha}=\frac{{BC}}{{OC}}=\frac{\mathrm{sin}\:\mathrm{30}×\mathrm{sin}\:\mathrm{20}}{\mathrm{sin}\:\mathrm{50}×\mathrm{sin}\:\mathrm{10}} \\ $$$$\mathrm{cos}\:\mathrm{10}+\frac{\mathrm{sin}\:\mathrm{10}}{\mathrm{tan}\:\alpha}=\frac{\mathrm{sin}\:\mathrm{30}×\mathrm{sin}\:\mathrm{20}}{\mathrm{sin}\:\mathrm{50}×\mathrm{sin}\:\mathrm{10}} \\ $$$$\mathrm{tan}\:\alpha=\frac{\mathrm{sin}\:\mathrm{10}}{\frac{\mathrm{sin}\:\mathrm{30}×\mathrm{sin}\:\mathrm{20}}{\mathrm{sin}\:\mathrm{50}×\mathrm{sin}\:\mathrm{10}}−\mathrm{cos}\:\mathrm{10}}=\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}} \\ $$$$\Rightarrow\alpha=\mathrm{30}° \\ $$
