
Question Number 123412 by bemath last updated on 25/Nov/20

Answered by liberty last updated on 25/Nov/20
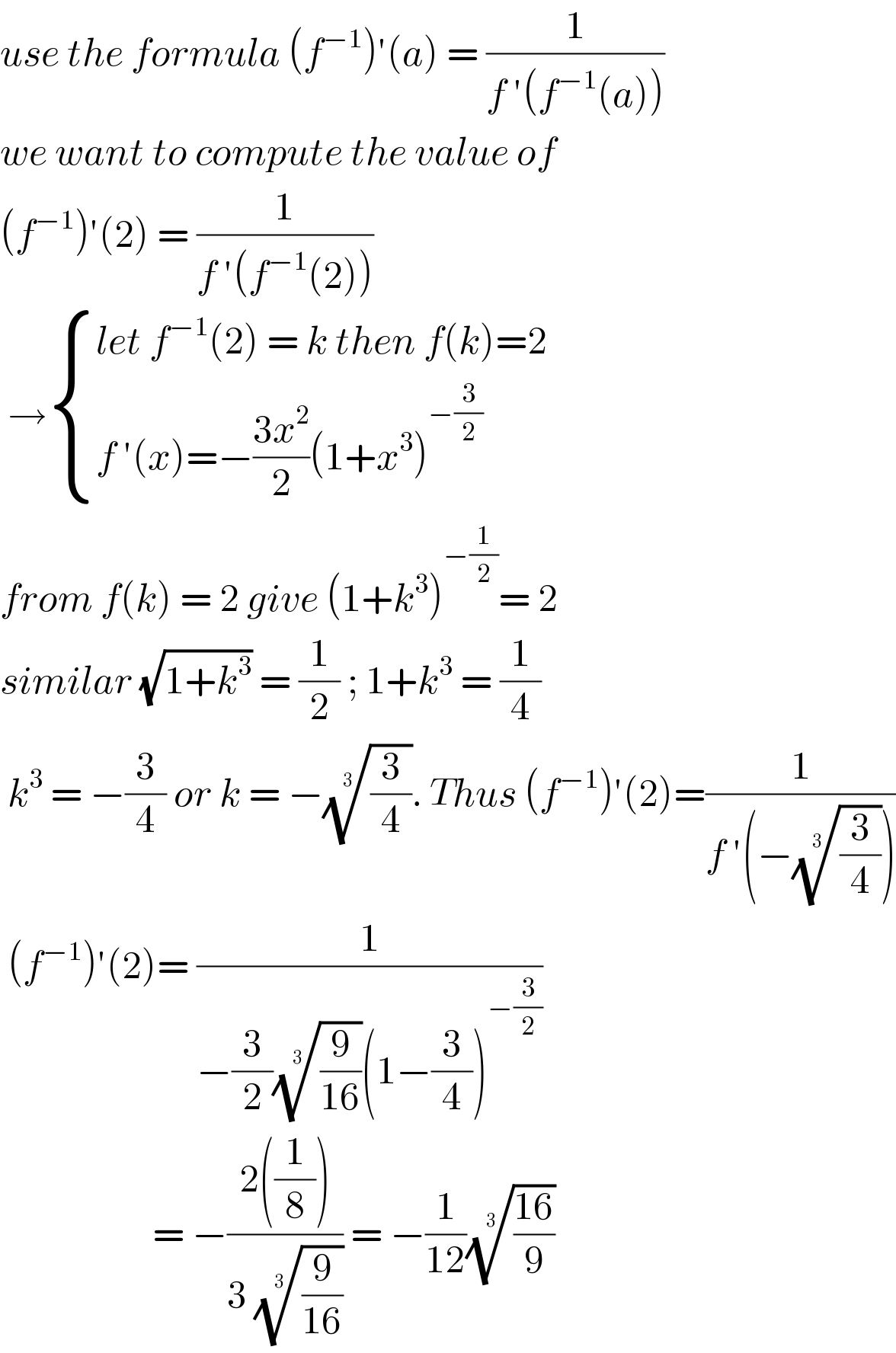
$${use}\:{the}\:{formula}\:\left({f}^{−\mathrm{1}} \right)'\left({a}\right)\:=\:\frac{\mathrm{1}}{{f}\:'\left({f}^{−\mathrm{1}} \left({a}\right)\right)} \\ $$$${we}\:{want}\:{to}\:{compute}\:{the}\:{value}\:{of}\: \\ $$$$\left({f}^{−\mathrm{1}} \right)'\left(\mathrm{2}\right)\:=\:\frac{\mathrm{1}}{{f}\:'\left({f}^{−\mathrm{1}} \left(\mathrm{2}\right)\right)} \\ $$$$\:\rightarrow\begin{cases}{{let}\:{f}^{−\mathrm{1}} \left(\mathrm{2}\right)\:=\:{k}\:{then}\:{f}\left({k}\right)=\mathrm{2}}\\{{f}\:'\left({x}\right)=−\frac{\mathrm{3}{x}^{\mathrm{2}} }{\mathrm{2}}\left(\mathrm{1}+{x}^{\mathrm{3}} \right)^{−\frac{\mathrm{3}}{\mathrm{2}}} }\end{cases} \\ $$$${from}\:{f}\left({k}\right)\:=\:\mathrm{2}\:{give}\:\left(\mathrm{1}+{k}^{\mathrm{3}} \right)^{−\frac{\mathrm{1}}{\mathrm{2}}} =\:\mathrm{2} \\ $$$${similar}\:\sqrt{\mathrm{1}+{k}^{\mathrm{3}} }\:=\:\frac{\mathrm{1}}{\mathrm{2}}\:;\:\mathrm{1}+{k}^{\mathrm{3}} \:=\:\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\:{k}^{\mathrm{3}} \:=\:−\frac{\mathrm{3}}{\mathrm{4}}\:{or}\:{k}\:=\:−\sqrt[{\mathrm{3}}]{\frac{\mathrm{3}}{\mathrm{4}}}.\:{Thus}\:\left({f}^{−\mathrm{1}} \right)'\left(\mathrm{2}\right)=\frac{\mathrm{1}}{{f}\:'\left(−\sqrt[{\mathrm{3}}]{\frac{\mathrm{3}}{\mathrm{4}}}\right)} \\ $$$$\:\left({f}^{−\mathrm{1}} \right)'\left(\mathrm{2}\right)=\:\frac{\mathrm{1}}{−\frac{\mathrm{3}}{\mathrm{2}}\sqrt[{\mathrm{3}}]{\frac{\mathrm{9}}{\mathrm{16}}}\left(\mathrm{1}−\frac{\mathrm{3}}{\mathrm{4}}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} } \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:−\frac{\mathrm{2}\left(\frac{\mathrm{1}}{\mathrm{8}}\right)}{\mathrm{3}\:\sqrt[{\mathrm{3}}]{\frac{\mathrm{9}}{\mathrm{16}}}}\:=\:−\frac{\mathrm{1}}{\mathrm{12}}\sqrt[{\mathrm{3}}]{\frac{\mathrm{16}}{\mathrm{9}}} \\ $$
Commented by Bird last updated on 25/Nov/20

$${correct}! \\ $$
Answered by TANMAY PANACEA last updated on 25/Nov/20
=(1/(f^′ (a))) here b=2 2=(1+a^3 )^((−1)/2) 2=(1/((1+a^3 )^(1/2) )) 4=(1/(1+a^3 ))→4+4a^3 =1 4a^3 =−3 a=(((−3)/4))^(1/3) f(x)=(1+x^3 )^((−1)/2) f^′ (x)=((−1)/2)×(1+x^3 )^((−3)/2) ×3x^2 required answdr =(1/((((−3x^2 (1+x^3 )^((−3)/2) )/2))_(x=a=(((−3)/4))^(1/3) ) )) Pls calvulatd](Q123445.png)
$${y}={f}\left({x}\right)\:\:{and}\:\left({a},{b}\right)\:{lies}\:{on}\:{y}={f}\left({x}\right) \\ $$$${b}={f}\left({a}\right) \\ $$$${formula}\:\:\left[{f}^{−\mathrm{1}} \right]\left({b}\right)=\frac{\mathrm{1}}{{f}^{'} \left({a}\right)} \\ $$$${here}\:{b}=\mathrm{2}\:\: \\ $$$$\mathrm{2}=\left(\mathrm{1}+{a}^{\mathrm{3}} \right)^{\frac{−\mathrm{1}}{\mathrm{2}}} \\ $$$$\mathrm{2}=\frac{\mathrm{1}}{\left(\mathrm{1}+{a}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{2}}} } \\ $$$$\mathrm{4}=\frac{\mathrm{1}}{\mathrm{1}+{a}^{\mathrm{3}} }\rightarrow\mathrm{4}+\mathrm{4}{a}^{\mathrm{3}} =\mathrm{1} \\ $$$$\mathrm{4}{a}^{\mathrm{3}} =−\mathrm{3} \\ $$$${a}=\left(\frac{−\mathrm{3}}{\mathrm{4}}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \\ $$$${f}\left({x}\right)=\left(\mathrm{1}+{x}^{\mathrm{3}} \right)^{\frac{−\mathrm{1}}{\mathrm{2}}} \\ $$$${f}^{'} \left({x}\right)=\frac{−\mathrm{1}}{\mathrm{2}}×\left(\mathrm{1}+{x}^{\mathrm{3}} \right)^{\frac{−\mathrm{3}}{\mathrm{2}}} ×\mathrm{3}{x}^{\mathrm{2}} \\ $$$${required}\:{answdr} \\ $$$$=\frac{\mathrm{1}}{\left(\frac{−\mathrm{3}{x}^{\mathrm{2}} \left(\mathrm{1}+{x}^{\mathrm{3}} \right)^{\frac{−\mathrm{3}}{\mathrm{2}}} }{\mathrm{2}}\right)_{{x}={a}=\left(\frac{−\mathrm{3}}{\mathrm{4}}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} } } \\ $$$$ \\ $$$$ \\ $$$${Pls}\:{calvulatd} \\ $$$$ \\ $$
Answered by Bird last updated on 25/Nov/20
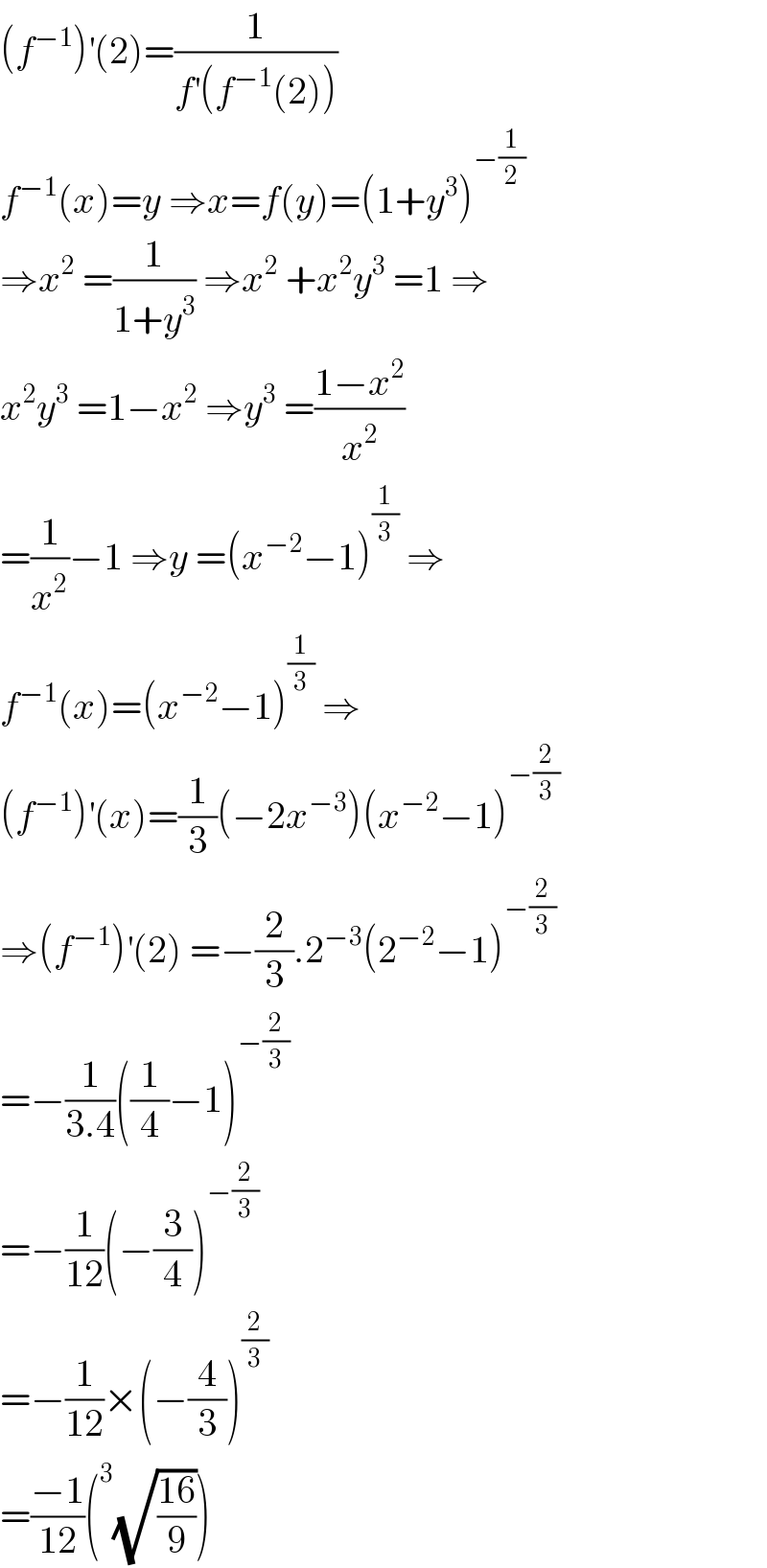
$$\left({f}^{−\mathrm{1}} \right)^{'} \left(\mathrm{2}\right)=\frac{\mathrm{1}}{{f}^{'} \left({f}^{−\mathrm{1}} \left(\mathrm{2}\right)\right)} \\ $$$${f}^{−\mathrm{1}} \left({x}\right)={y}\:\Rightarrow{x}={f}\left({y}\right)=\left(\mathrm{1}+{y}^{\mathrm{3}} \right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$\Rightarrow{x}^{\mathrm{2}} \:=\frac{\mathrm{1}}{\mathrm{1}+{y}^{\mathrm{3}} }\:\Rightarrow{x}^{\mathrm{2}} \:+{x}^{\mathrm{2}} {y}^{\mathrm{3}} \:=\mathrm{1}\:\Rightarrow \\ $$$${x}^{\mathrm{2}} {y}^{\mathrm{3}} \:=\mathrm{1}−{x}^{\mathrm{2}} \:\Rightarrow{y}^{\mathrm{3}} \:=\frac{\mathrm{1}−{x}^{\mathrm{2}} }{{x}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{{x}^{\mathrm{2}} }−\mathrm{1}\:\Rightarrow{y}\:=\left({x}^{−\mathrm{2}} −\mathrm{1}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:\Rightarrow \\ $$$${f}^{−\mathrm{1}} \left({x}\right)=\left({x}^{−\mathrm{2}} −\mathrm{1}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:\Rightarrow \\ $$$$\left({f}^{−\mathrm{1}} \right)^{'} \left({x}\right)=\frac{\mathrm{1}}{\mathrm{3}}\left(−\mathrm{2}{x}^{−\mathrm{3}} \right)\left({x}^{−\mathrm{2}} −\mathrm{1}\right)^{−\frac{\mathrm{2}}{\mathrm{3}}} \\ $$$$\Rightarrow\left({f}^{−\mathrm{1}} \right)^{'} \left(\mathrm{2}\right)\:=−\frac{\mathrm{2}}{\mathrm{3}}.\mathrm{2}^{−\mathrm{3}} \left(\mathrm{2}^{−\mathrm{2}} −\mathrm{1}\right)^{−\frac{\mathrm{2}}{\mathrm{3}}} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{3}.\mathrm{4}}\left(\frac{\mathrm{1}}{\mathrm{4}}−\mathrm{1}\right)^{−\frac{\mathrm{2}}{\mathrm{3}}} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{12}}\left(−\frac{\mathrm{3}}{\mathrm{4}}\right)^{−\frac{\mathrm{2}}{\mathrm{3}}} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{12}}×\left(−\frac{\mathrm{4}}{\mathrm{3}}\right)^{\frac{\mathrm{2}}{\mathrm{3}}} \\ $$$$=\frac{−\mathrm{1}}{\mathrm{12}}\left(^{\mathrm{3}} \sqrt{\frac{\mathrm{16}}{\mathrm{9}}}\right) \\ $$
