
Question Number 122111 by ajfour last updated on 14/Nov/20

Commented by ajfour last updated on 14/Nov/20
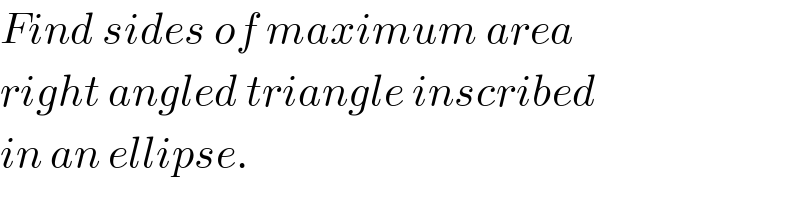
$${Find}\:{sides}\:{of}\:{maximum}\:{area} \\ $$$${right}\:{angled}\:{triangle}\:{inscribed} \\ $$$${in}\:{an}\:{ellipse}. \\ $$
Commented by MJS_new last updated on 14/Nov/20

$$\mathrm{it}'\mathrm{s}\:\mathrm{part}\:\mathrm{of}\:\mathrm{the}\:\mathrm{problem}\:\mathrm{to}\:\mathrm{find}\:\mathrm{the}\:\mathrm{general} \\ $$$$\mathrm{triangle}\:\mathrm{with}\:\mathrm{maximum}\:\mathrm{area}.\:\mathrm{I}\:\mathrm{solved}\:\mathrm{it}, \\ $$$$\mathrm{cannot}\:\mathrm{find}\:\mathrm{the}\:\mathrm{old}\:\mathrm{question}. \\ $$$$\mathrm{all}\:\mathrm{triangles}\:\mathrm{with}\:\mathrm{centroid}\:\mathrm{in}\:\mathrm{the}\:\mathrm{center}\:\mathrm{of} \\ $$$$\mathrm{the}\:\mathrm{ellipse}\:\mathrm{have}\:\mathrm{equal}\:\mathrm{area}.\:\mathrm{we}\:\mathrm{have}\:\mathrm{to}\:\mathrm{find} \\ $$$$\mathrm{the}\:\mathrm{rectangular}\:\mathrm{triangles}\:\mathrm{out}\:\mathrm{of}\:\mathrm{these} \\ $$
Commented by MJS_new last updated on 14/Nov/20

$$\mathrm{I}\:\mathrm{only}\:\mathrm{have}\:\mathrm{the}\:\mathrm{formulas}\:\mathrm{for}\:\mathrm{the}\:\mathrm{axes}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{smallest}\:\mathrm{ellipse}\:\mathrm{around}\:\mathrm{a}\:\mathrm{given}\:\mathrm{triangle}\:\mathrm{with} \\ $$$$\mathrm{sides}\:{a},\:{b},\:{c}\:\mathrm{which}\:\mathrm{is} \\ $$$$\frac{\mathrm{1}}{\mathrm{3}}\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} \pm\mathrm{2}\sqrt{{a}^{\mathrm{2}} {b}^{\mathrm{2}} +{a}^{\mathrm{2}} {c}^{\mathrm{2}} +{b}^{\mathrm{2}} {c}^{\mathrm{2}} −\delta^{\mathrm{2}} }} \\ $$$$\mathrm{where}\:\delta=\sqrt{\left({a}+{b}+{c}\right)\left({a}+{b}−{c}\right)\left({a}+{c}−{b}\right)\left({b}+{c}−{a}\right)} \\ $$$$\mathrm{if}\:\mathrm{we}\:\mathrm{let}\:{c}=\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:\mathrm{we}\:\mathrm{get} \\ $$$$\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} \pm\sqrt{{a}^{\mathrm{4}} −{a}^{\mathrm{2}} {b}^{\mathrm{2}} +{b}^{\mathrm{4}} }} \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{this}\:\mathrm{for}\:{a},\:{b}\:\mathrm{with}\:\mathrm{given} \\ $$$$\mathrm{axes}\:\alpha,\:\beta: \\ $$$$\begin{cases}{\alpha=\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +\sqrt{{a}^{\mathrm{4}} −{a}^{\mathrm{2}} {b}^{\mathrm{2}} +{b}^{\mathrm{4}} }}}\\{\beta=\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} −\sqrt{{a}^{\mathrm{4}} −{a}^{\mathrm{2}} {b}^{\mathrm{2}} +{b}^{\mathrm{4}} }}}\end{cases} \\ $$$$\mathrm{sorry}\:\mathrm{I}\:\mathrm{have}\:\mathrm{got}\:\mathrm{no}\:\mathrm{time}\:\mathrm{right}\:\mathrm{now} \\ $$
Commented by MJS_new last updated on 14/Nov/20
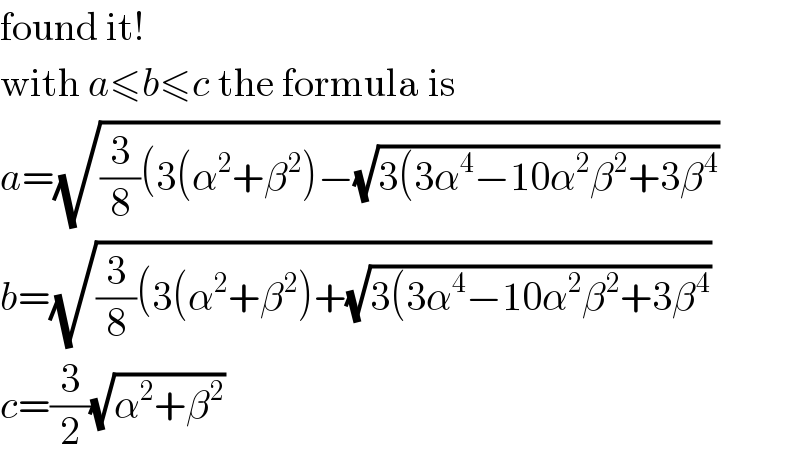
$$\mathrm{found}\:\mathrm{it}! \\ $$$$\mathrm{with}\:{a}\leqslant{b}\leqslant{c}\:\mathrm{the}\:\mathrm{formula}\:\mathrm{is} \\ $$$${a}=\sqrt{\frac{\mathrm{3}}{\mathrm{8}}\left(\mathrm{3}\left(\alpha^{\mathrm{2}} +\beta^{\mathrm{2}} \right)−\sqrt{\mathrm{3}\left(\mathrm{3}\alpha^{\mathrm{4}} −\mathrm{10}\alpha^{\mathrm{2}} \beta^{\mathrm{2}} +\mathrm{3}\beta^{\mathrm{4}} \right.}\right.} \\ $$$${b}=\sqrt{\frac{\mathrm{3}}{\mathrm{8}}\left(\mathrm{3}\left(\alpha^{\mathrm{2}} +\beta^{\mathrm{2}} \right)+\sqrt{\mathrm{3}\left(\mathrm{3}\alpha^{\mathrm{4}} −\mathrm{10}\alpha^{\mathrm{2}} \beta^{\mathrm{2}} +\mathrm{3}\beta^{\mathrm{4}} \right.}\right.} \\ $$$${c}=\frac{\mathrm{3}}{\mathrm{2}}\sqrt{\alpha^{\mathrm{2}} +\beta^{\mathrm{2}} } \\ $$
Commented by ajfour last updated on 14/Nov/20
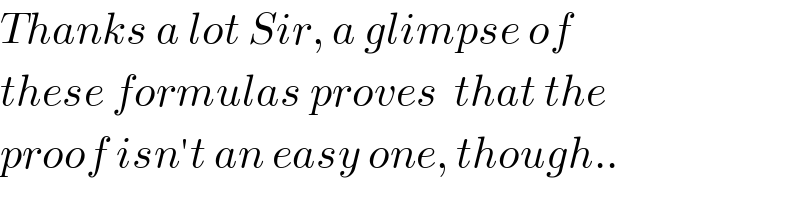
$${Thanks}\:{a}\:{lot}\:{Sir},\:{a}\:{glimpse}\:{of} \\ $$$${these}\:{formulas}\:{proves}\:\:{that}\:{the} \\ $$$${proof}\:{isn}'{t}\:{an}\:{easy}\:{one},\:{though}.. \\ $$
Commented by MJS_new last updated on 14/Nov/20

$$\mathrm{my}\:\mathrm{idea}\:\mathrm{had}\:\mathrm{been}\:\mathrm{to}\:\mathrm{start}\:\mathrm{with}\:\mathrm{a}\:\mathrm{unit}\:\mathrm{circle} \\ $$$$\mathrm{and}\:\mathrm{an}\:\mathrm{inscribed}\:\mathrm{equilateral}\:\mathrm{triangle} \\ $$$$\mathrm{then}\:\mathrm{first}\:\mathrm{rotate}\:\mathrm{the}\:\mathrm{triangle}\:\mathrm{by}\:\varphi\:\mathrm{and}\:\mathrm{second} \\ $$$$\mathrm{compress}\:\mathrm{the}\:\mathrm{whole}\:\mathrm{thing}\:\mathrm{to}\:\mathrm{get}\:\mathrm{the}\:\mathrm{desired} \\ $$$$\mathrm{ellipse}.\:\mathrm{this}\:\mathrm{way}\:\mathrm{it}'\mathrm{s}\:\mathrm{obvious}\:\mathrm{that}\:\mathrm{the}\:\mathrm{centroid} \\ $$$$\mathrm{stays}\:\mathrm{in}\:\mathrm{the}\:\mathrm{center}\:\mathrm{and}\:\mathrm{all}\:\mathrm{triangles}\:\mathrm{you}\:\mathrm{get} \\ $$$$\mathrm{have}\:\mathrm{the}\:\mathrm{same}\:\mathrm{area}\:\mathrm{and}\:\mathrm{there}'\mathrm{s}\:\mathrm{no}\:\mathrm{triangle} \\ $$$$\mathrm{with}\:\mathrm{a}\:\mathrm{greater}\:\mathrm{area}. \\ $$$$\mathrm{I}\:\mathrm{wanted}\:\mathrm{to}\:\mathrm{find}\:\mathrm{the}\:\mathrm{smallest}\:\mathrm{ellipse}\:\mathrm{around} \\ $$$$\mathrm{a}\:\mathrm{given}\:\mathrm{triangle}.\:\mathrm{I}\:\mathrm{kept}\:\mathrm{the}\:\mathrm{formulas}\:\mathrm{but}\:\mathrm{not} \\ $$$$\mathrm{the}\:\mathrm{path}.\:\mathrm{will}\:\mathrm{send}\:\mathrm{them}\:\mathrm{again}\:\mathrm{but}\:\mathrm{not}\:\mathrm{now} \\ $$
Commented by MJS_new last updated on 14/Nov/20

$$\mathrm{there}'\mathrm{s}\:\mathrm{no}\:\mathrm{rectangular}\:\mathrm{triangle}\:\mathrm{for}\:\mathrm{ellipses} \\ $$$$\mathrm{with}\:\alpha\geqslant\beta\:\wedge\:\beta>\frac{\alpha}{\:\sqrt{\mathrm{3}}}.\:\mathrm{for}\:\beta=\frac{\alpha}{\:\sqrt{\mathrm{3}}}\:\mathrm{the}\:\mathrm{triangle} \\ $$$$\mathrm{is}\:\mathrm{isosceles} \\ $$$$\mathrm{plus}\:\mathrm{I}\:\mathrm{corrected}\:\mathrm{a}\:\mathrm{typo}\:\mathrm{in}\:\mathrm{the}\:\mathrm{formulas}\:\mathrm{I}\:\mathrm{gave} \\ $$$$\mathrm{before}.\:−\mathrm{10}{a}^{\mathrm{2}} {b}^{\mathrm{2}} \:\mathrm{not}\:−\mathrm{20}{a}^{\mathrm{2}} {b}^{\mathrm{2}} \\ $$
Answered by ajfour last updated on 14/Nov/20
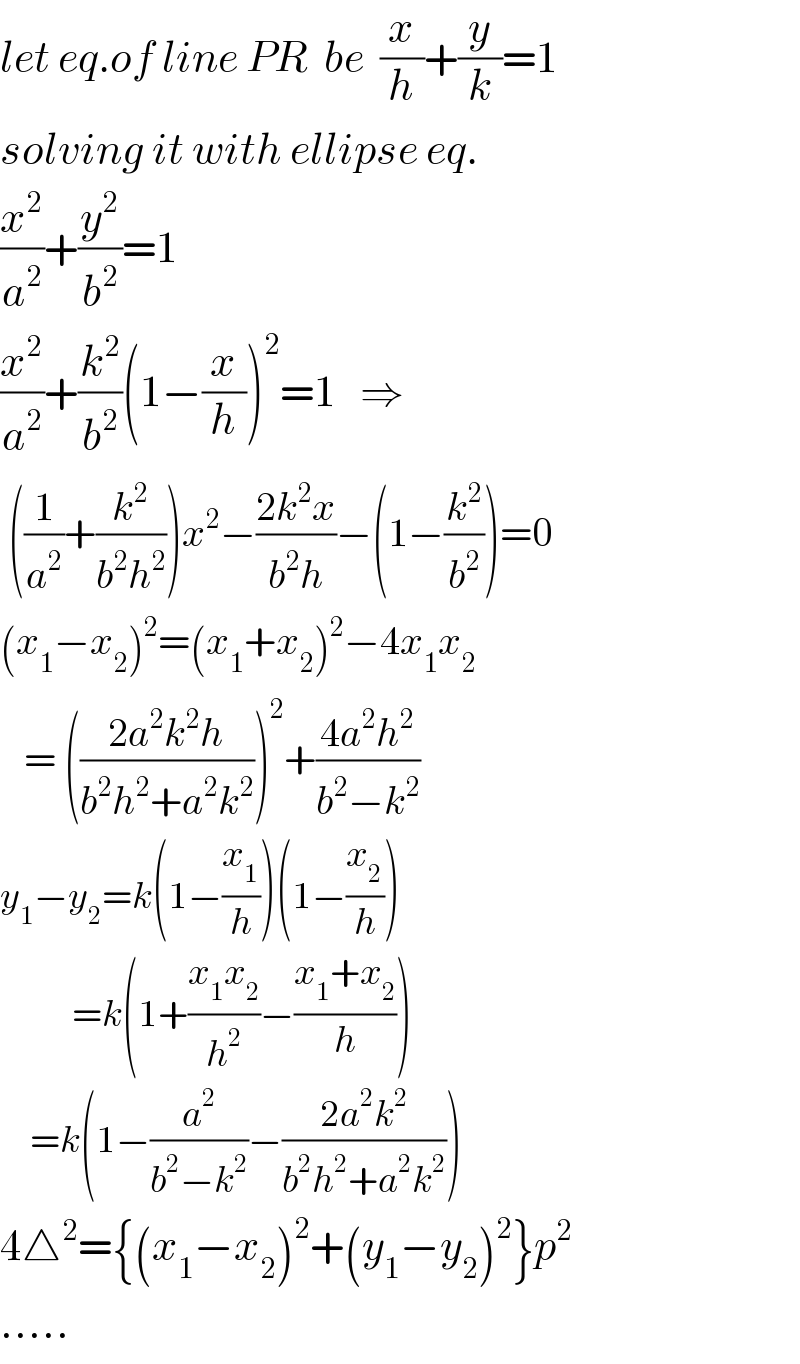
$${let}\:{eq}.{of}\:{line}\:{PR}\:\:{be}\:\:\frac{{x}}{{h}}+\frac{{y}}{{k}}=\mathrm{1} \\ $$$${solving}\:{it}\:{with}\:{ellipse}\:{eq}. \\ $$$$\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }=\mathrm{1} \\ $$$$\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }+\frac{{k}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\left(\mathrm{1}−\frac{{x}}{{h}}\right)^{\mathrm{2}} =\mathrm{1}\:\:\:\Rightarrow \\ $$$$\:\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{{k}^{\mathrm{2}} }{{b}^{\mathrm{2}} {h}^{\mathrm{2}} }\right){x}^{\mathrm{2}} −\frac{\mathrm{2}{k}^{\mathrm{2}} {x}}{{b}^{\mathrm{2}} {h}}−\left(\mathrm{1}−\frac{{k}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\right)=\mathrm{0} \\ $$$$\left({x}_{\mathrm{1}} −{x}_{\mathrm{2}} \right)^{\mathrm{2}} =\left({x}_{\mathrm{1}} +{x}_{\mathrm{2}} \right)^{\mathrm{2}} −\mathrm{4}{x}_{\mathrm{1}} {x}_{\mathrm{2}} \\ $$$$\:\:\:=\:\left(\frac{\mathrm{2}{a}^{\mathrm{2}} {k}^{\mathrm{2}} {h}}{{b}^{\mathrm{2}} {h}^{\mathrm{2}} +{a}^{\mathrm{2}} {k}^{\mathrm{2}} }\right)^{\mathrm{2}} +\frac{\mathrm{4}{a}^{\mathrm{2}} {h}^{\mathrm{2}} }{{b}^{\mathrm{2}} −{k}^{\mathrm{2}} } \\ $$$${y}_{\mathrm{1}} −{y}_{\mathrm{2}} ={k}\left(\mathrm{1}−\frac{{x}_{\mathrm{1}} }{{h}}\right)\left(\mathrm{1}−\frac{{x}_{\mathrm{2}} }{{h}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:={k}\left(\mathrm{1}+\frac{{x}_{\mathrm{1}} {x}_{\mathrm{2}} }{{h}^{\mathrm{2}} }−\frac{{x}_{\mathrm{1}} +{x}_{\mathrm{2}} }{{h}}\right) \\ $$$$\:\:\:\:\:={k}\left(\mathrm{1}−\frac{{a}^{\mathrm{2}} }{{b}^{\mathrm{2}} −{k}^{\mathrm{2}} }−\frac{\mathrm{2}{a}^{\mathrm{2}} {k}^{\mathrm{2}} }{{b}^{\mathrm{2}} {h}^{\mathrm{2}} +{a}^{\mathrm{2}} {k}^{\mathrm{2}} }\right) \\ $$$$\mathrm{4}\bigtriangleup^{\mathrm{2}} =\left\{\left({x}_{\mathrm{1}} −{x}_{\mathrm{2}} \right)^{\mathrm{2}} +\left({y}_{\mathrm{1}} −{y}_{\mathrm{2}} \right)^{\mathrm{2}} \right\}{p}^{\mathrm{2}} \\ $$$$..... \\ $$
