Question and Answers Forum

All Questions      Topic List

Logarithms Questions

Previous in All Question      Next in All Question      

Previous in Logarithms      Next in Logarithms      

Question Number 121138 by Ar Brandon last updated on 05/Nov/20

Answered by TANMAY PANACEA last updated on 05/Nov/20

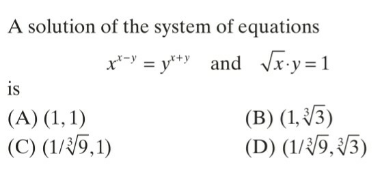
x^(x−x^(−(1/2)) ) =(x^(−(1/2)) )^(x+x^((−1)/2) ) x−x^(−(1/2)) =((−x)/2)−(1/2)x^(−(1/2)) ((3x)/2)=(1/2)x^((−1)/2) 3x=x^((−1)/2) 3x^(3/2) =1 x^(3/2) =(1/3)→x=((1/3))^(2/3) =(1/( (9)^(1/3) )) y=(1/( (√x)))=(1/( ((1/3))^((2/3)×(1/2)) ))=(1/(1/( (3)^(1/3) )))=(3)^(1/)) option D((1/( (9)^(1/3) )),(3)^(1/3) )

$${x}^{{x}−{x}^{−\frac{\mathrm{1}}{\mathrm{2}}} } =\left({x}^{−\frac{\mathrm{1}}{\mathrm{2}}} \right)^{{x}+{x}^{\frac{−\mathrm{1}}{\mathrm{2}}} } \\ $$$${x}−{x}^{−\frac{\mathrm{1}}{\mathrm{2}}} =\frac{−{x}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}{x}^{−\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$\frac{\mathrm{3}{x}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}}{x}^{\frac{−\mathrm{1}}{\mathrm{2}}} \\ $$$$\mathrm{3}{x}={x}^{\frac{−\mathrm{1}}{\mathrm{2}}} \\ $$$$\mathrm{3}{x}^{\frac{\mathrm{3}}{\mathrm{2}}} =\mathrm{1} \\ $$$${x}^{\frac{\mathrm{3}}{\mathrm{2}}} =\frac{\mathrm{1}}{\mathrm{3}}\rightarrow{x}=\left(\frac{\mathrm{1}}{\mathrm{3}}\right)^{\frac{\mathrm{2}}{\mathrm{3}}} =\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\mathrm{9}}} \\ $$$${y}=\frac{\mathrm{1}}{\:\sqrt{{x}}}=\frac{\mathrm{1}}{\:\left(\frac{\mathrm{1}}{\mathrm{3}}\right)^{\frac{\mathrm{2}}{\mathrm{3}}×\frac{\mathrm{1}}{\mathrm{2}}} }=\frac{\mathrm{1}}{\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\mathrm{3}}}}=\sqrt[{\left.\right)}]{\mathrm{3}}\: \\ $$$${option}\:{D}\left(\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\mathrm{9}}},\sqrt[{\mathrm{3}}]{\mathrm{3}}\:\right) \\ $$

Commented by Ar Brandon last updated on 05/Nov/20

Thanks Sir

Commented by TANMAY PANACEA last updated on 05/Nov/20

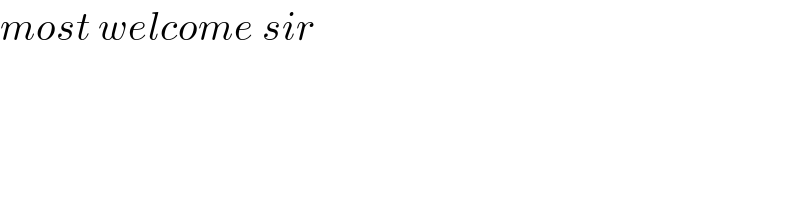
most welcome sir

$${most}\:{welcome}\:{sir} \\ $$

Answered by Dwaipayan Shikari last updated on 05/Nov/20

x^(x−y) =y^(x+y) ⇒x^(x+y) =y^(x+y) x^(2y) ⇒((x/y))^(x+y) =x^(2y) ⇒(x^(3/2) )^(x+(1/( (√x)))) =x^(2/( (√x))) ⇒x= 1 3(x+(1/( (√x))))=(4/( (√x))) ⇒3x=(1/( (√x)))⇒x=(1/( (9)^(1/3) )) y=(3)^(1/3) So x=1,y=1 x=(1/( (9)^(1/3) )) y=(3)^(1/3)

$${x}^{{x}−{y}} ={y}^{{x}+{y}} \\ $$$$\Rightarrow{x}^{{x}+{y}} ={y}^{{x}+{y}} {x}^{\mathrm{2}{y}} \\ $$$$\Rightarrow\left(\frac{{x}}{{y}}\right)^{{x}+{y}} ={x}^{\mathrm{2}{y}} \\ $$$$\Rightarrow\left({x}^{\frac{\mathrm{3}}{\mathrm{2}}} \right)^{{x}+\frac{\mathrm{1}}{\:\sqrt{{x}}}} ={x}^{\frac{\mathrm{2}}{\:\sqrt{{x}}}} \\ $$$$\Rightarrow{x}=\:\mathrm{1} \\ $$$$\mathrm{3}\left({x}+\frac{\mathrm{1}}{\:\sqrt{{x}}}\right)=\frac{\mathrm{4}}{\:\sqrt{{x}}} \\ $$$$\Rightarrow\mathrm{3}{x}=\frac{\mathrm{1}}{\:\sqrt{{x}}}\Rightarrow{x}=\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\mathrm{9}}} \\ $$$${y}=\sqrt[{\mathrm{3}}]{\mathrm{3}} \\ $$$${So}\:\:{x}=\mathrm{1},{y}=\mathrm{1}\:\:{x}=\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\mathrm{9}}}\:{y}=\sqrt[{\mathrm{3}}]{\mathrm{3}} \\ $$

Commented by Ar Brandon last updated on 05/Nov/20

Brilliant ! Thanks Mr Dwaipayan����

Commented by Ar Brandon last updated on 05/Nov/20

��You'll have to get used-to it bro��

Terms of Service

Privacy Policy

Contact: info@tinkutara.com