Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 119173 by help last updated on 22/Oct/20

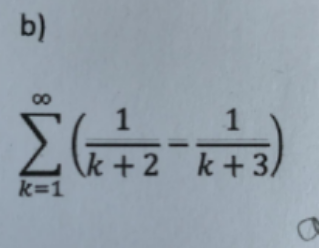
Answered by Olaf last updated on 22/Oct/20

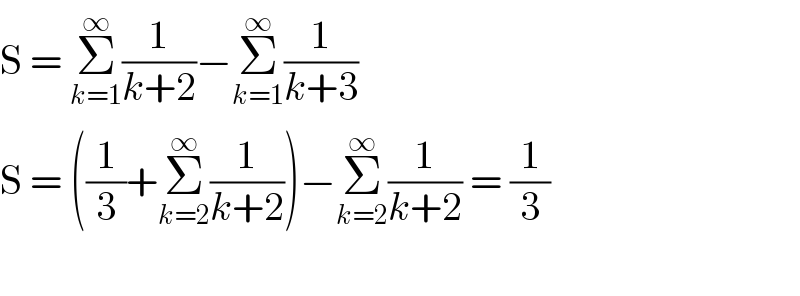
S = Σ_(k=1) ^∞ (1/(k+2))−Σ_(k=1) ^∞ (1/(k+3)) S = ((1/3)+Σ_(k=2) ^∞ (1/(k+2)))−Σ_(k=2) ^∞ (1/(k+2)) = (1/3)

$$\mathrm{S}\:=\:\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{k}+\mathrm{2}}−\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{k}+\mathrm{3}} \\ $$$$\mathrm{S}\:=\:\left(\frac{\mathrm{1}}{\mathrm{3}}+\underset{{k}=\mathrm{2}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{k}+\mathrm{2}}\right)−\underset{{k}=\mathrm{2}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{k}+\mathrm{2}}\:=\:\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$ \\ $$

Answered by JDamian last updated on 22/Oct/20

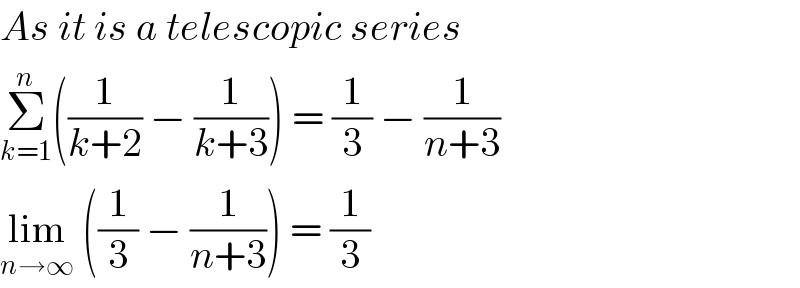
As it is a telescopic series Σ_(k=1) ^n ((1/(k+2)) − (1/(k+3))) = (1/3) − (1/(n+3)) lim_(n→∞) ((1/3) − (1/(n+3))) = (1/3)

$${As}\:{it}\:{is}\:{a}\:{telescopic}\:{series} \\ $$$$\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\left(\frac{\mathrm{1}}{{k}+\mathrm{2}}\:−\:\frac{\mathrm{1}}{{k}+\mathrm{3}}\right)\:=\:\frac{\mathrm{1}}{\mathrm{3}}\:−\:\frac{\mathrm{1}}{{n}+\mathrm{3}} \\ $$$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\:\left(\frac{\mathrm{1}}{\mathrm{3}}\:−\:\frac{\mathrm{1}}{{n}+\mathrm{3}}\right)\:=\:\frac{\mathrm{1}}{\mathrm{3}} \\ $$

Answered by mathdave last updated on 22/Oct/20

solution Σ_(k=1) ^∞ (1/(k+2))−Σ_(k=1) ^∞ (1/(k+3))=I=(Σ_(k=1) ^∞ (1/k)−1−(1/2))−(Σ_(k=1) ^∞ (1/k)−1−(1/2)−(1/3)) I=−(3/2)+((11)/6)=((−9+11)/6)=(2/6)=(1/3) ∵Σ_(k=1) ^∞ ((1/(k+2))−(1/(k+3)))=(1/3)

$${solution} \\ $$$$\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{k}+\mathrm{2}}−\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{k}+\mathrm{3}}={I}=\left(\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{k}}−\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\right)−\left(\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{k}}−\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{3}}\right) \\ $$$${I}=−\frac{\mathrm{3}}{\mathrm{2}}+\frac{\mathrm{11}}{\mathrm{6}}=\frac{−\mathrm{9}+\mathrm{11}}{\mathrm{6}}=\frac{\mathrm{2}}{\mathrm{6}}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\because\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{{k}+\mathrm{2}}−\frac{\mathrm{1}}{{k}+\mathrm{3}}\right)=\frac{\mathrm{1}}{\mathrm{3}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com