Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 118811 by ajfour last updated on 19/Oct/20

Commented by ajfour last updated on 19/Oct/20

What is the side length of the equal sided hexagon inscribed within the triangle ABC as shown?

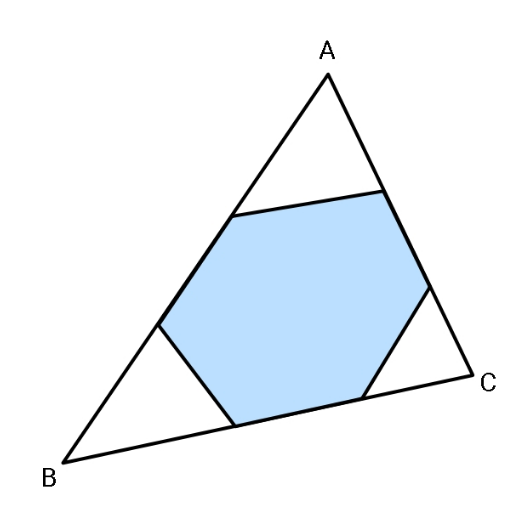
$${What}\:{is}\:{the}\:{side}\:{length}\:{of}\:{the}\:{equal} \\ $$$${sided}\:{hexagon}\:{inscribed}\:{within}\:{the} \\ $$$${triangle}\:{ABC}\:{as}\:{shown}? \\ $$

Commented by I want to learn more last updated on 20/Oct/20

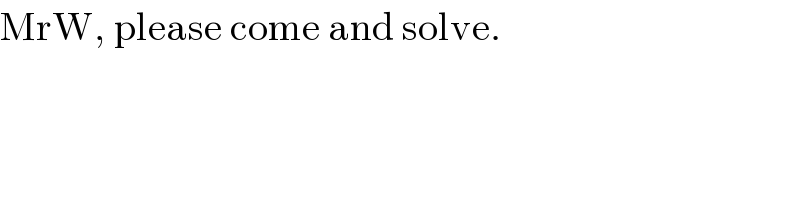
MrW, please come and solve.

$$\mathrm{MrW},\:\mathrm{please}\:\mathrm{come}\:\mathrm{and}\:\mathrm{solve}.\: \\ $$

Commented by mr W last updated on 21/Oct/20

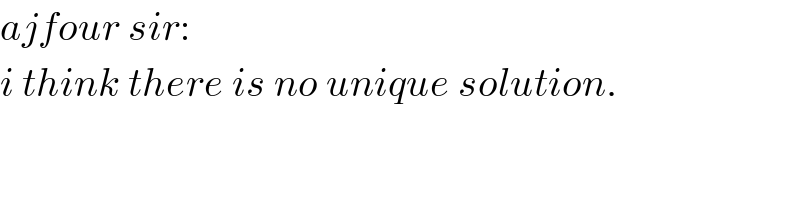
ajfour sir: i think there is no unique solution.

$${ajfour}\:{sir}: \\ $$$${i}\:{think}\:{there}\:{is}\:{no}\:{unique}\:{solution}. \\ $$

Commented by ajfour last updated on 21/Oct/20

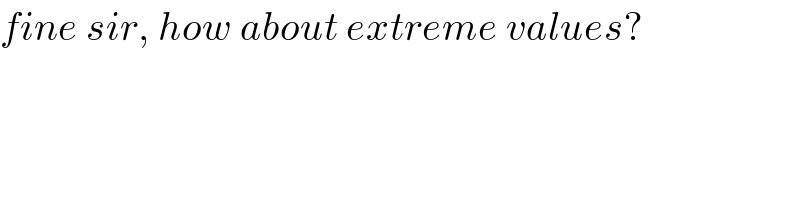
fine sir, how about extreme values?

$${fine}\:{sir},\:{how}\:{about}\:{extreme}\:{values}? \\ $$

Answered by 1549442205PVT last updated on 20/Oct/20

This problem has infinite many of solutions in case the triangle ABC is equilateral since we can construct an infinite number of hexagons with equal sides and side length is different

$$\mathrm{This}\:\mathrm{problem}\:\mathrm{has}\:\mathrm{infinite}\:\mathrm{many}\:\mathrm{of} \\ $$$$\mathrm{solutions}\:\mathrm{in}\:\mathrm{case}\:\mathrm{the}\:\mathrm{triangle}\:\:\mathrm{ABC}\: \\ $$$$\mathrm{is}\:\mathrm{equilateral}\:\mathrm{since}\:\mathrm{we}\:\mathrm{can}\:\mathrm{construct} \\ $$$$\mathrm{an}\:\mathrm{infinite}\:\mathrm{number}\:\mathrm{of}\:\mathrm{hexagons}\:\mathrm{with} \\ $$$$\mathrm{equal}\:\mathrm{sides}\:\mathrm{and}\:\mathrm{side}\:\mathrm{length}\:\mathrm{is}\:\mathrm{different} \\ $$

Commented by ajfour last updated on 20/Oct/20

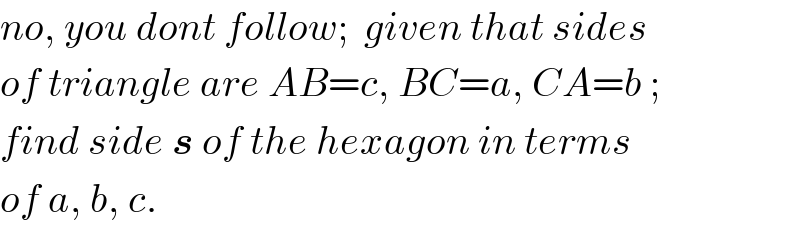
no, you dont follow; given that sides of triangle are AB=c, BC=a, CA=b ; find side s of the hexagon in terms of a, b, c.

$${no},\:{you}\:{dont}\:{follow};\:\:{given}\:{that}\:{sides} \\ $$$${of}\:{triangle}\:{are}\:{AB}={c},\:{BC}={a},\:{CA}={b}\:; \\ $$$${find}\:{side}\:\boldsymbol{{s}}\:{of}\:{the}\:{hexagon}\:{in}\:{terms} \\ $$$${of}\:{a},\:{b},\:{c}. \\ $$

Commented by 1549442205PVT last updated on 20/Oct/20

Commented by 1549442205PVT last updated on 20/Oct/20

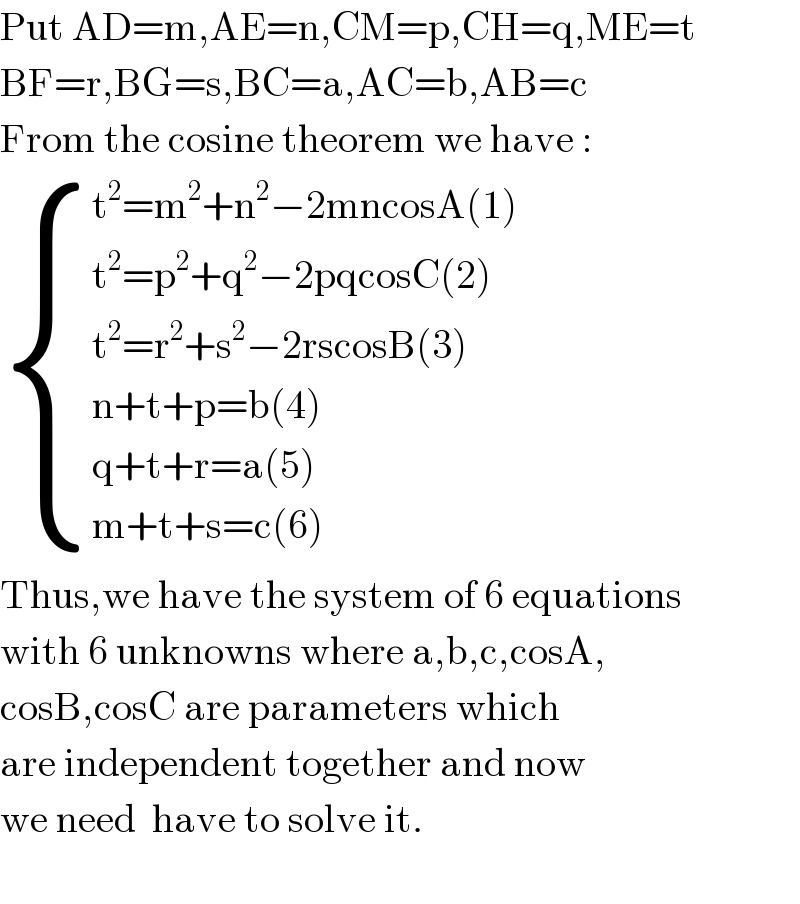
Put AD=m,AE=n,CM=p,CH=q,ME=t BF=r,BG=s,BC=a,AC=b,AB=c From the cosine theorem we have : { ((t^2 =m^2 +n^2 −2mncosA(1))),((t^2 =p^2 +q^2 −2pqcosC(2))),((t^2 =r^2 +s^2 −2rscosB(3))),((n+t+p=b(4))),((q+t+r=a(5))),((m+t+s=c(6))) :} Thus,we have the system of 6 equations with 6 unknowns where a,b,c,cosA, cosB,cosC are parameters which are independent together and now we need have to solve it.

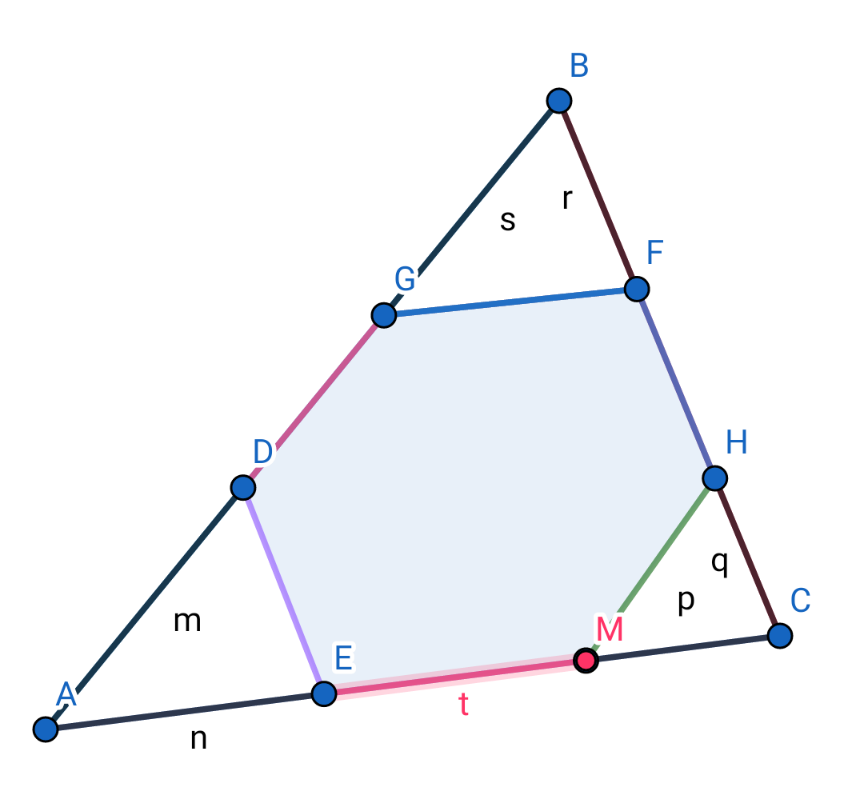
$$\mathrm{Put}\:\mathrm{AD}=\mathrm{m},\mathrm{AE}=\mathrm{n},\mathrm{CM}=\mathrm{p},\mathrm{CH}=\mathrm{q},\mathrm{ME}=\mathrm{t} \\ $$$$\mathrm{BF}=\mathrm{r},\mathrm{BG}=\mathrm{s},\mathrm{BC}=\mathrm{a},\mathrm{AC}=\mathrm{b},\mathrm{AB}=\mathrm{c} \\ $$$$\mathrm{From}\:\mathrm{the}\:\mathrm{cosine}\:\mathrm{theorem}\:\mathrm{we}\:\mathrm{have}\:: \\ $$$$\begin{cases}{\mathrm{t}^{\mathrm{2}} =\mathrm{m}^{\mathrm{2}} +\mathrm{n}^{\mathrm{2}} −\mathrm{2mncosA}\left(\mathrm{1}\right)}\\{\mathrm{t}^{\mathrm{2}} =\mathrm{p}^{\mathrm{2}} +\mathrm{q}^{\mathrm{2}} −\mathrm{2pqcosC}\left(\mathrm{2}\right)}\\{\mathrm{t}^{\mathrm{2}} =\mathrm{r}^{\mathrm{2}} +\mathrm{s}^{\mathrm{2}} −\mathrm{2rscosB}\left(\mathrm{3}\right)}\\{\mathrm{n}+\mathrm{t}+\mathrm{p}=\mathrm{b}\left(\mathrm{4}\right)}\\{\mathrm{q}+\mathrm{t}+\mathrm{r}=\mathrm{a}\left(\mathrm{5}\right)}\\{\mathrm{m}+\mathrm{t}+\mathrm{s}=\mathrm{c}\left(\mathrm{6}\right)}\end{cases} \\ $$$$\mathrm{Thus},\mathrm{we}\:\mathrm{have}\:\mathrm{the}\:\mathrm{system}\:\mathrm{of}\:\mathrm{6}\:\mathrm{equations} \\ $$$$\mathrm{with}\:\mathrm{6}\:\mathrm{unknowns}\:\mathrm{where}\:\mathrm{a},\mathrm{b},\mathrm{c},\mathrm{cosA}, \\ $$$$\mathrm{cosB},\mathrm{cosC}\:\mathrm{are}\:\mathrm{parameters}\:\mathrm{which}\: \\ $$$$\mathrm{are}\:\mathrm{independent}\:\mathrm{together}\:\mathrm{and}\:\mathrm{now} \\ $$$$\mathrm{we}\:\mathrm{need}\:\:\mathrm{have}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{it}. \\ $$$$ \\ $$

Commented by I want to learn more last updated on 20/Oct/20

Sir, 1549442205PVT which app you use to draw.

$$\mathrm{Sir},\:\mathrm{1549442205PVT}\:\:\mathrm{which}\:\mathrm{app}\:\mathrm{you}\:\mathrm{use}\:\mathrm{to}\:\mathrm{draw}. \\ $$

Commented by 1549442205PVT last updated on 21/Oct/20

Geogebra,Drawing of tinkutara done this

$$\mathrm{Geogebra},\mathrm{Drawing}\:\mathrm{of}\:\mathrm{tinkutara}\:\mathrm{done}\:\mathrm{this} \\ $$

Commented by mr W last updated on 21/Oct/20

6 equations, but 7 unknowns, does it mean that there is no unique solution?

$$\mathrm{6}\:{equations},\:{but}\:\mathrm{7}\:{unknowns}, \\ $$$${does}\:{it}\:{mean}\:{that}\:{there}\:{is}\:{no}\:{unique} \\ $$$${solution}? \\ $$

Commented by 1549442205PVT last updated on 25/Oct/20

Yes,Sir is right.I also have such thinkings

$$\mathrm{Yes},\mathrm{Sir}\:\mathrm{is}\:\mathrm{right}.\mathrm{I}\:\:\mathrm{also}\:\mathrm{have}\:\mathrm{such}\:\mathrm{thinkings} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com