Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 115871 by bemath last updated on 29/Sep/20

Commented by bemath last updated on 29/Sep/20

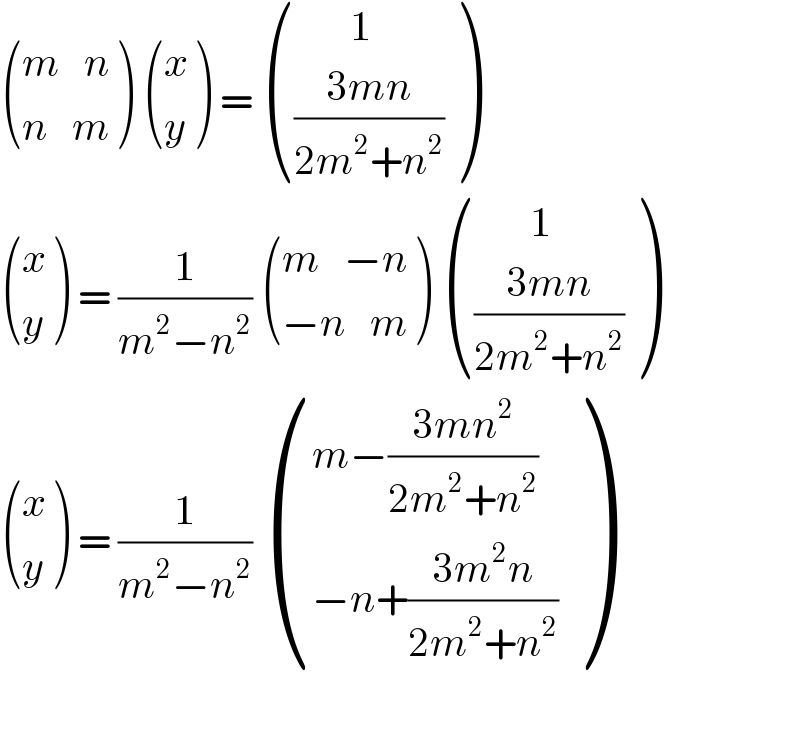
(((m n)),((n m)) ) ((x),(y) ) = ((( 1)),(((3mn)/(2m^2 +n^2 ))) ) ((x),(y) ) = (1/(m^2 −n^2 )) (((m −n)),((−n m)) ) ((( 1)),(((3mn)/(2m^2 +n^2 ))) ) ((x),(y) ) = (1/(m^2 −n^2 )) (((m−((3mn^2 )/(2m^2 +n^2 )))),((−n+((3m^2 n)/(2m^2 +n^2 )))) )

$$\begin{pmatrix}{{m}\:\:\:{n}}\\{{n}\:\:\:{m}}\end{pmatrix}\:\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:=\:\begin{pmatrix}{\:\:\:\:\:\:\:\mathrm{1}}\\{\frac{\mathrm{3}{mn}}{\mathrm{2}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} }}\end{pmatrix} \\ $$$$\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:=\:\frac{\mathrm{1}}{{m}^{\mathrm{2}} −{n}^{\mathrm{2}} }\:\begin{pmatrix}{{m}\:\:\:−{n}}\\{−{n}\:\:\:{m}}\end{pmatrix}\:\begin{pmatrix}{\:\:\:\:\:\:\:\mathrm{1}}\\{\frac{\mathrm{3}{mn}}{\mathrm{2}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} }}\end{pmatrix} \\ $$$$\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:=\:\frac{\mathrm{1}}{{m}^{\mathrm{2}} −{n}^{\mathrm{2}} }\:\begin{pmatrix}{{m}−\frac{\mathrm{3}{mn}^{\mathrm{2}} }{\mathrm{2}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} }}\\{−{n}+\frac{\mathrm{3}{m}^{\mathrm{2}} {n}}{\mathrm{2}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} }}\end{pmatrix} \\ $$$$ \\ $$

Answered by MJS_new last updated on 29/Sep/20

mx+ny=1 nx+my=((3mn)/(2m^2 +n^2 )) ⇒ x=((2m)/(2m^2 +n^2 ))∧y=(n/(2m^2 +n^2 )) ⇒ (x^2 +y^2 )(2m^2 +2n^2 )=((2(m^2 +n^2 )(4m^2 +n^2 ))/((2m^2 +n^2 )^2 ))=α transforming leads to 4m^4 +((2(2α−5))/(α−2))m^2 n^2 +n^4 =0 let m=((√(2p))/2)∧n=(√q); p, q ≥0 p^2 +((2α−5)/(α−2))pq+q^2 =0 (p+q)^2 =((pq)/(α−2))∧(p−q)^2 =−((4α−9)/(α−2))pq ⇒ α≠2∧(1/(α−2))≥0∧−((4α−9)/(α−2))≥0 ⇒ 2<α≤(9/4) but maybe I′m wrong...

$${mx}+{ny}=\mathrm{1} \\ $$$${nx}+{my}=\frac{\mathrm{3}{mn}}{\mathrm{2}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} } \\ $$$$\Rightarrow\:{x}=\frac{\mathrm{2}{m}}{\mathrm{2}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} }\wedge{y}=\frac{{n}}{\mathrm{2}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} } \\ $$$$\Rightarrow \\ $$$$\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)\left(\mathrm{2}{m}^{\mathrm{2}} +\mathrm{2}{n}^{\mathrm{2}} \right)=\frac{\mathrm{2}\left({m}^{\mathrm{2}} +{n}^{\mathrm{2}} \right)\left(\mathrm{4}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} \right)}{\left(\mathrm{2}{m}^{\mathrm{2}} +{n}^{\mathrm{2}} \right)^{\mathrm{2}} }=\alpha \\ $$$$\mathrm{transforming}\:\mathrm{leads}\:\mathrm{to} \\ $$$$\mathrm{4}{m}^{\mathrm{4}} +\frac{\mathrm{2}\left(\mathrm{2}\alpha−\mathrm{5}\right)}{\alpha−\mathrm{2}}{m}^{\mathrm{2}} {n}^{\mathrm{2}} +{n}^{\mathrm{4}} =\mathrm{0} \\ $$$$\mathrm{let}\:{m}=\frac{\sqrt{\mathrm{2}{p}}}{\mathrm{2}}\wedge{n}=\sqrt{{q}};\:{p},\:{q}\:\geqslant\mathrm{0} \\ $$$${p}^{\mathrm{2}} +\frac{\mathrm{2}\alpha−\mathrm{5}}{\alpha−\mathrm{2}}{pq}+{q}^{\mathrm{2}} =\mathrm{0} \\ $$$$\left({p}+{q}\right)^{\mathrm{2}} =\frac{{pq}}{\alpha−\mathrm{2}}\wedge\left({p}−{q}\right)^{\mathrm{2}} =−\frac{\mathrm{4}\alpha−\mathrm{9}}{\alpha−\mathrm{2}}{pq} \\ $$$$\Rightarrow\:\alpha\neq\mathrm{2}\wedge\frac{\mathrm{1}}{\alpha−\mathrm{2}}\geqslant\mathrm{0}\wedge−\frac{\mathrm{4}\alpha−\mathrm{9}}{\alpha−\mathrm{2}}\geqslant\mathrm{0} \\ $$$$\Rightarrow\:\mathrm{2}<\alpha\leqslant\frac{\mathrm{9}}{\mathrm{4}} \\ $$$$\mathrm{but}\:\mathrm{maybe}\:\mathrm{I}'\mathrm{m}\:\mathrm{wrong}... \\ $$

Commented by MJS_new last updated on 29/Sep/20

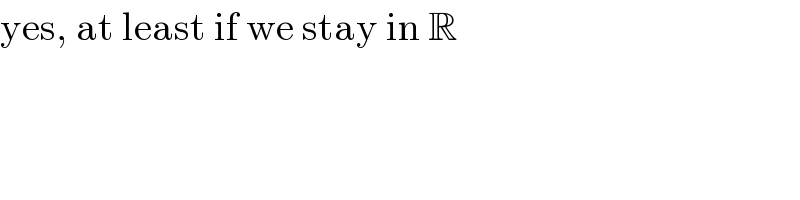
yes, at least if we stay in R

$$\mathrm{yes},\:\mathrm{at}\:\mathrm{least}\:\mathrm{if}\:\mathrm{we}\:\mathrm{stay}\:\mathrm{in}\:\mathbb{R} \\ $$

Commented by bemath last updated on 29/Sep/20

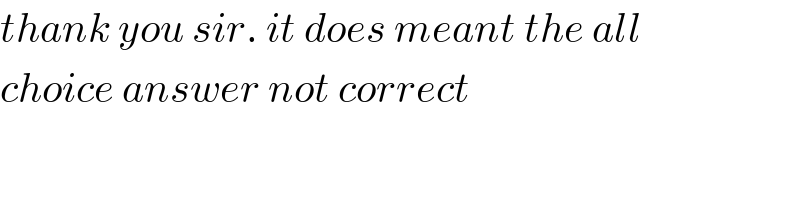
thank you sir. it does meant the all choice answer not correct

$${thank}\:{you}\:{sir}.\:{it}\:{does}\:{meant}\:{the}\:{all}\: \\ $$$${choice}\:{answer}\:{not}\:{correct} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com