Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 113333 by mohammad17 last updated on 12/Sep/20

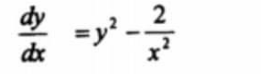
Answered by mr W last updated on 13/Sep/20

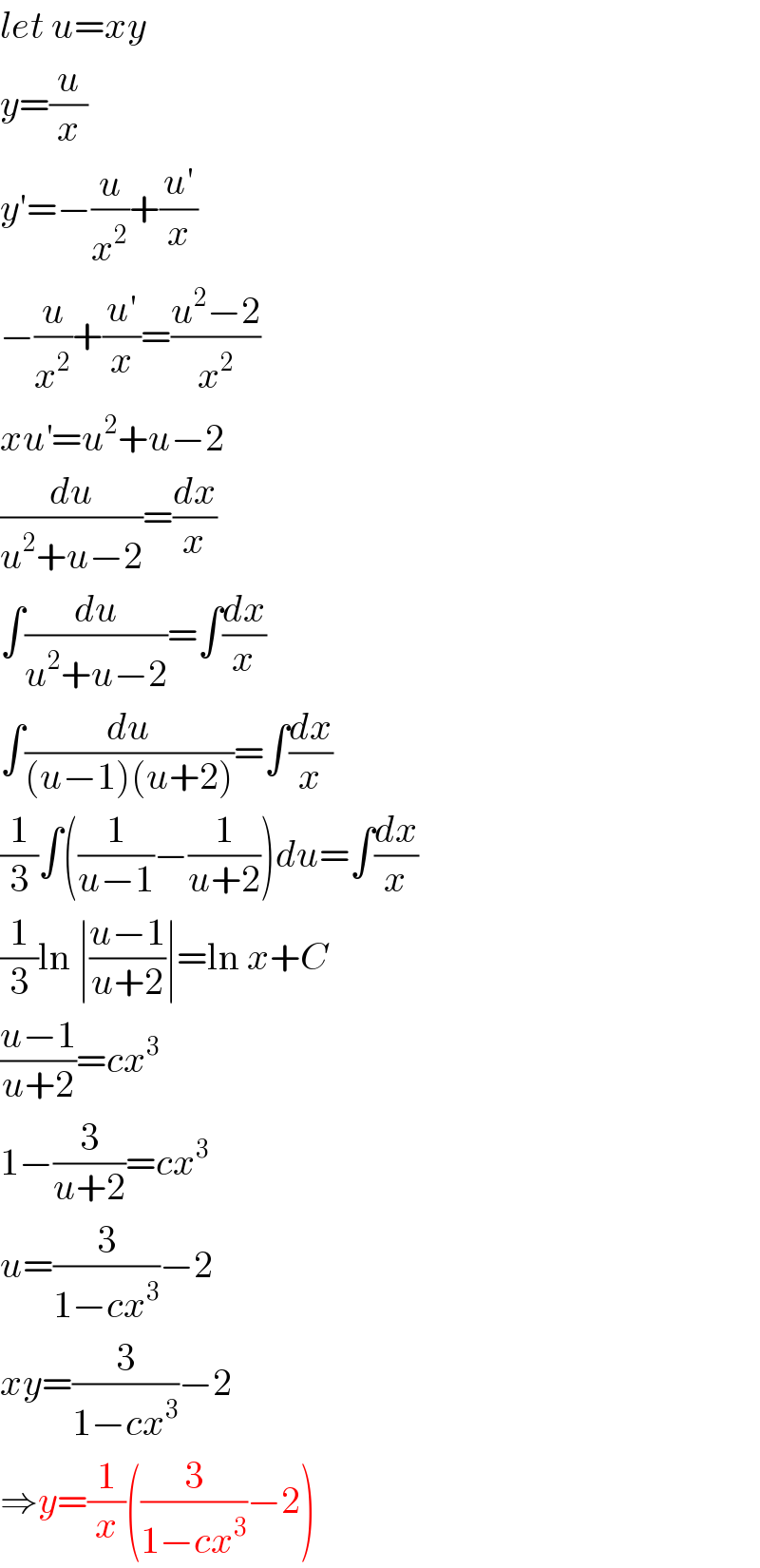
let u=xy y=(u/x) y′=−(u/x^2 )+((u′)/x) −(u/x^2 )+((u′)/x)=((u^2 −2)/x^2 ) xu^′ =u^2 +u−2 (du/(u^2 +u−2))=(dx/x) ∫(du/(u^2 +u−2))=∫(dx/x) ∫(du/((u−1)(u+2)))=∫(dx/x) (1/3)∫((1/(u−1))−(1/(u+2)))du=∫(dx/x) (1/3)ln ∣((u−1)/(u+2))∣=ln x+C ((u−1)/(u+2))=cx^3 1−(3/(u+2))=cx^3 u=(3/(1−cx^3 ))−2 xy=(3/(1−cx^3 ))−2 ⇒y=(1/x)((3/(1−cx^3 ))−2)

$${let}\:{u}={xy} \\ $$$${y}=\frac{{u}}{{x}} \\ $$$${y}'=−\frac{{u}}{{x}^{\mathrm{2}} }+\frac{{u}'}{{x}} \\ $$$$−\frac{{u}}{{x}^{\mathrm{2}} }+\frac{{u}'}{{x}}=\frac{{u}^{\mathrm{2}} −\mathrm{2}}{{x}^{\mathrm{2}} } \\ $$$${xu}^{'} ={u}^{\mathrm{2}} +{u}−\mathrm{2} \\ $$$$\frac{{du}}{{u}^{\mathrm{2}} +{u}−\mathrm{2}}=\frac{{dx}}{{x}} \\ $$$$\int\frac{{du}}{{u}^{\mathrm{2}} +{u}−\mathrm{2}}=\int\frac{{dx}}{{x}} \\ $$$$\int\frac{{du}}{\left({u}−\mathrm{1}\right)\left({u}+\mathrm{2}\right)}=\int\frac{{dx}}{{x}} \\ $$$$\frac{\mathrm{1}}{\mathrm{3}}\int\left(\frac{\mathrm{1}}{{u}−\mathrm{1}}−\frac{\mathrm{1}}{{u}+\mathrm{2}}\right){du}=\int\frac{{dx}}{{x}} \\ $$$$\frac{\mathrm{1}}{\mathrm{3}}\mathrm{ln}\:\mid\frac{{u}−\mathrm{1}}{{u}+\mathrm{2}}\mid=\mathrm{ln}\:{x}+{C} \\ $$$$\frac{{u}−\mathrm{1}}{{u}+\mathrm{2}}={cx}^{\mathrm{3}} \\ $$$$\mathrm{1}−\frac{\mathrm{3}}{{u}+\mathrm{2}}={cx}^{\mathrm{3}} \\ $$$${u}=\frac{\mathrm{3}}{\mathrm{1}−{cx}^{\mathrm{3}} }−\mathrm{2} \\ $$$${xy}=\frac{\mathrm{3}}{\mathrm{1}−{cx}^{\mathrm{3}} }−\mathrm{2} \\ $$$$\Rightarrow{y}=\frac{\mathrm{1}}{{x}}\left(\frac{\mathrm{3}}{\mathrm{1}−{cx}^{\mathrm{3}} }−\mathrm{2}\right) \\ $$

Answered by bobhans last updated on 13/Sep/20

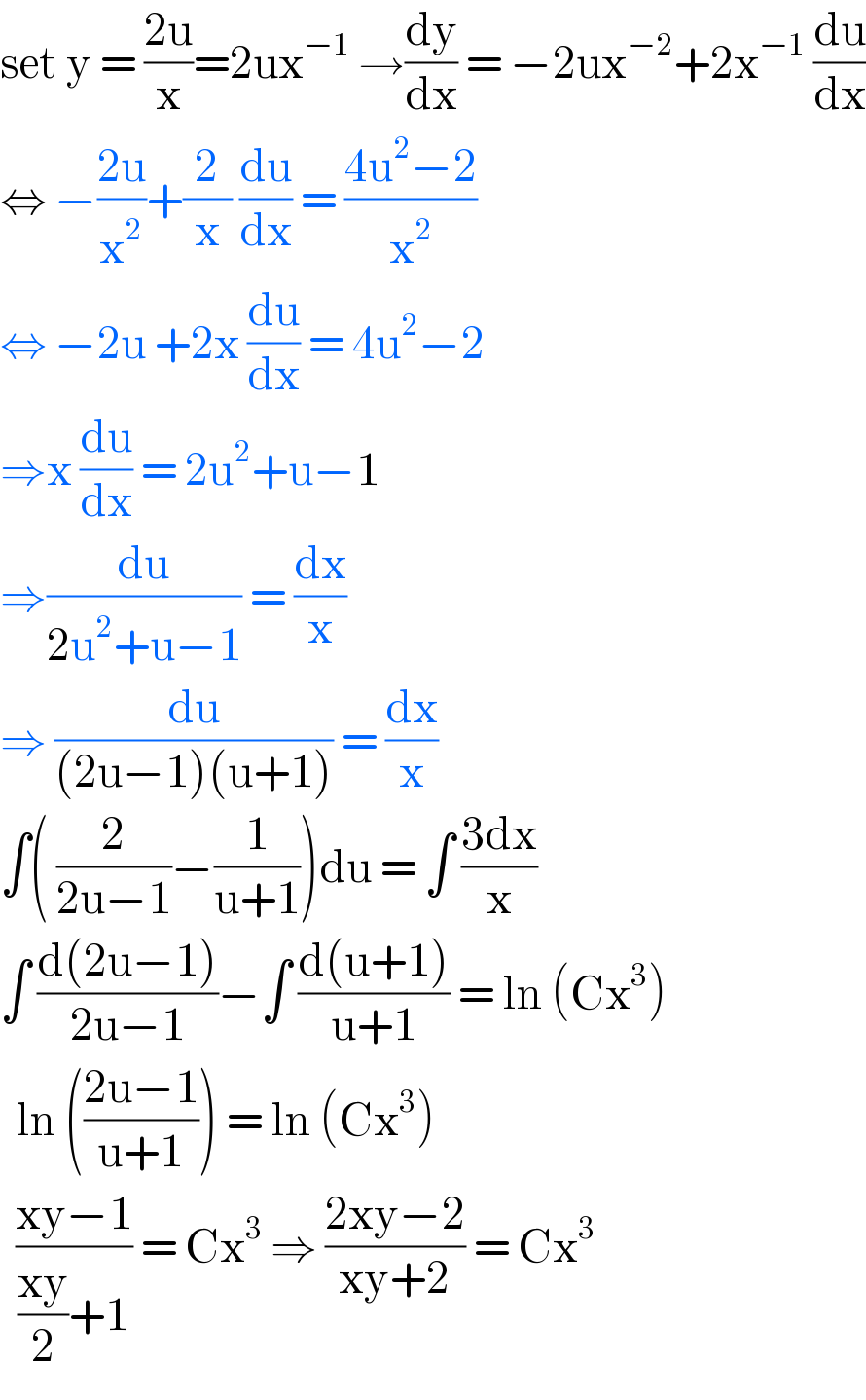
set y = ((2u)/x)=2ux^(−1) →(dy/dx) = −2ux^(−2) +2x^(−1) (du/dx) ⇔ −((2u)/x^2 )+(2/x) (du/dx) = ((4u^2 −2)/x^2 ) ⇔ −2u +2x (du/dx) = 4u^2 −2 ⇒x (du/dx) = 2u^2 +u−1 ⇒(du/(2u^2 +u−1)) = (dx/x) ⇒ (du/((2u−1)(u+1))) = (dx/x) ∫( (2/(2u−1))−(1/(u+1)))du = ∫ ((3dx)/x) ∫ ((d(2u−1))/(2u−1))−∫ ((d(u+1))/(u+1)) = ln (Cx^3 ) ln (((2u−1)/(u+1))) = ln (Cx^3 ) ((xy−1)/(((xy)/2)+1)) = Cx^3 ⇒ ((2xy−2)/(xy+2)) = Cx^3

$$\mathrm{set}\:\mathrm{y}\:=\:\frac{\mathrm{2u}}{\mathrm{x}}=\mathrm{2ux}^{−\mathrm{1}} \:\rightarrow\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:−\mathrm{2ux}^{−\mathrm{2}} +\mathrm{2x}^{−\mathrm{1}} \:\frac{\mathrm{du}}{\mathrm{dx}} \\ $$$$\Leftrightarrow\:−\frac{\mathrm{2u}}{\mathrm{x}^{\mathrm{2}} }+\frac{\mathrm{2}}{\mathrm{x}}\:\frac{\mathrm{du}}{\mathrm{dx}}\:=\:\frac{\mathrm{4u}^{\mathrm{2}} −\mathrm{2}}{\mathrm{x}^{\mathrm{2}} } \\ $$$$\Leftrightarrow\:−\mathrm{2u}\:+\mathrm{2x}\:\frac{\mathrm{du}}{\mathrm{dx}}\:=\:\mathrm{4u}^{\mathrm{2}} −\mathrm{2} \\ $$$$\Rightarrow\mathrm{x}\:\frac{\mathrm{du}}{\mathrm{dx}}\:=\:\mathrm{2u}^{\mathrm{2}} +\mathrm{u}−\mathrm{1} \\ $$$$\Rightarrow\frac{\mathrm{du}}{\mathrm{2u}^{\mathrm{2}} +\mathrm{u}−\mathrm{1}}\:=\:\frac{\mathrm{dx}}{\mathrm{x}} \\ $$$$\Rightarrow\:\frac{\mathrm{du}}{\left(\mathrm{2u}−\mathrm{1}\right)\left(\mathrm{u}+\mathrm{1}\right)}\:=\:\frac{\mathrm{dx}}{\mathrm{x}} \\ $$$$\int\left(\:\frac{\mathrm{2}}{\mathrm{2u}−\mathrm{1}}−\frac{\mathrm{1}}{\mathrm{u}+\mathrm{1}}\right)\mathrm{du}\:=\:\int\:\frac{\mathrm{3dx}}{\mathrm{x}} \\ $$$$\int\:\frac{\mathrm{d}\left(\mathrm{2u}−\mathrm{1}\right)}{\mathrm{2u}−\mathrm{1}}−\int\:\frac{\mathrm{d}\left(\mathrm{u}+\mathrm{1}\right)}{\mathrm{u}+\mathrm{1}}\:=\:\mathrm{ln}\:\left(\mathrm{Cx}^{\mathrm{3}} \right) \\ $$$$\:\:\mathrm{ln}\:\left(\frac{\mathrm{2u}−\mathrm{1}}{\mathrm{u}+\mathrm{1}}\right)\:=\:\mathrm{ln}\:\left(\mathrm{Cx}^{\mathrm{3}} \right) \\ $$$$\:\:\frac{\mathrm{xy}−\mathrm{1}}{\frac{\mathrm{xy}}{\mathrm{2}}+\mathrm{1}}\:=\:\mathrm{Cx}^{\mathrm{3}} \:\Rightarrow\:\frac{\mathrm{2xy}−\mathrm{2}}{\mathrm{xy}+\mathrm{2}}\:=\:\mathrm{Cx}^{\mathrm{3}} \\ $$

Commented by bobhans last updated on 13/Sep/20

hahaha..yes. you are right

$$\mathrm{hahaha}..\mathrm{yes}.\:\mathrm{you}\:\mathrm{are}\:\mathrm{right} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com