Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 112885 by malwan last updated on 10/Sep/20

Commented by malwan last updated on 10/Sep/20

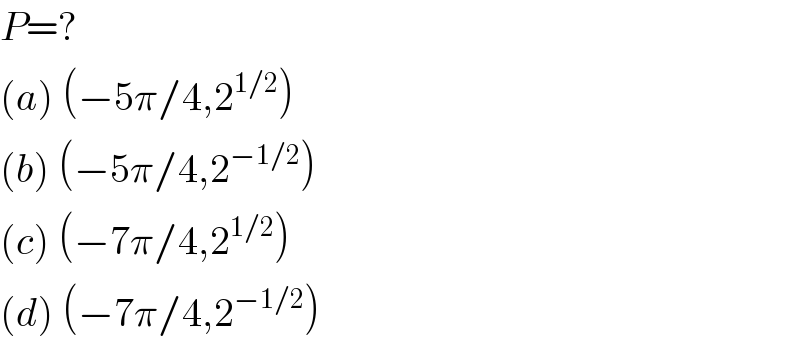
P=? (a) (−5π/4,2^(1/2) ) (b) (−5π/4,2^(−1/2) ) (c) (−7π/4,2^(1/2) ) (d) (−7π/4,2^(−1/2) )

$${P}=? \\ $$$$\left({a}\right)\:\left(−\mathrm{5}\pi/\mathrm{4},\mathrm{2}^{\mathrm{1}/\mathrm{2}} \right) \\ $$$$\left({b}\right)\:\left(−\mathrm{5}\pi/\mathrm{4},\mathrm{2}^{−\mathrm{1}/\mathrm{2}} \right) \\ $$$$\left({c}\right)\:\left(−\mathrm{7}\pi/\mathrm{4},\mathrm{2}^{\mathrm{1}/\mathrm{2}} \right) \\ $$$$\left({d}\right)\:\left(−\mathrm{7}\pi/\mathrm{4},\mathrm{2}^{−\mathrm{1}/\mathrm{2}} \right) \\ $$

Commented by malwan last updated on 10/Sep/20

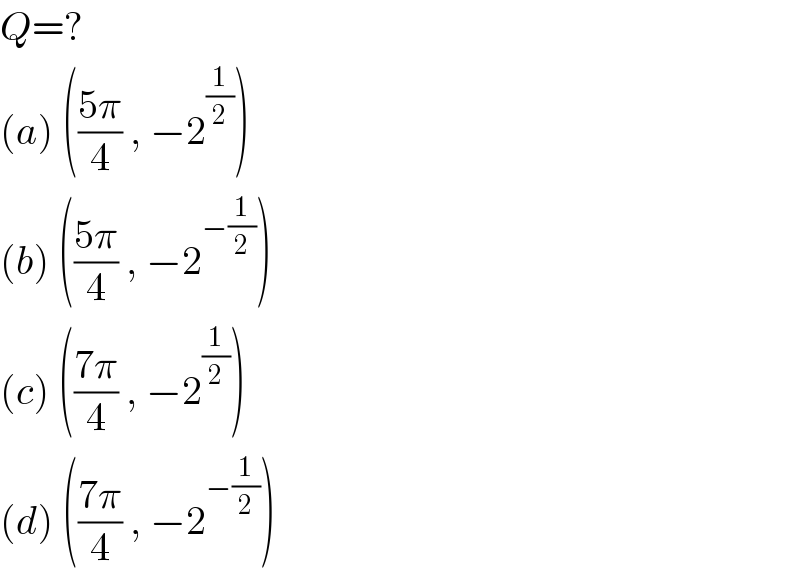
Q=? (a) (((5π)/4) , −2^(1/2) ) (b) (((5π)/4) , −2^(−(1/2)) ) (c) (((7π)/4) , −2^(1/2) ) (d) (((7π)/4) , −2^(−(1/2)) )

$${Q}=? \\ $$$$\left({a}\right)\:\left(\frac{\mathrm{5}\pi}{\mathrm{4}}\:,\:−\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{2}}} \right) \\ $$$$\left({b}\right)\:\left(\frac{\mathrm{5}\pi}{\mathrm{4}}\:,\:−\mathrm{2}^{−\frac{\mathrm{1}}{\mathrm{2}}} \right) \\ $$$$\left({c}\right)\:\left(\frac{\mathrm{7}\pi}{\mathrm{4}}\:,\:−\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{2}}} \right) \\ $$$$\left({d}\right)\:\left(\frac{\mathrm{7}\pi}{\mathrm{4}}\:,\:−\mathrm{2}^{−\frac{\mathrm{1}}{\mathrm{2}}} \right) \\ $$

Commented by Aziztisffola last updated on 10/Sep/20

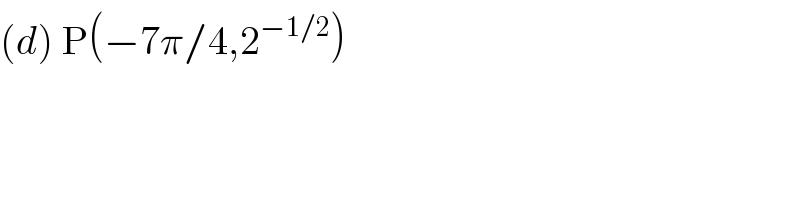
(d) P(−7π/4,2^(−1/2) )

$$\left({d}\right)\:\mathrm{P}\left(−\mathrm{7}\pi/\mathrm{4},\mathrm{2}^{−\mathrm{1}/\mathrm{2}} \right) \\ $$

Commented by Aziztisffola last updated on 10/Sep/20

(b) Q(((5π)/4) , −2^(−(1/2)) )

$$\left({b}\right)\:\mathrm{Q}\left(\frac{\mathrm{5}\pi}{\mathrm{4}}\:,\:−\mathrm{2}^{−\frac{\mathrm{1}}{\mathrm{2}}} \right) \\ $$

Commented by malwan last updated on 10/Sep/20

thank you

$${thank}\:{you} \\ $$

Commented by malwan last updated on 10/Sep/20

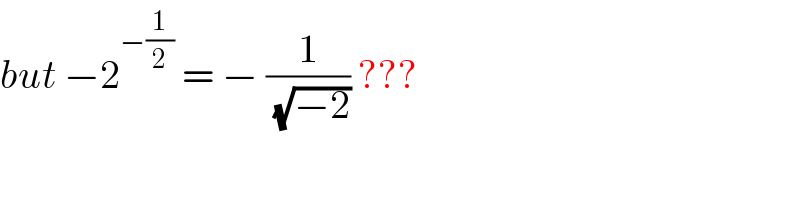
but −2^(−(1/2)) = − (1/( (√(−2)))) ???

$${but}\:−\mathrm{2}^{−\frac{\mathrm{1}}{\mathrm{2}}} \:=\:−\:\frac{\mathrm{1}}{\:\sqrt{−\mathrm{2}}}\:??? \\ $$

Commented by Aziztisffola last updated on 10/Sep/20

−2^(−(1/2)) =−(1/2^(1/2) )=−(1/( (√2)))

$$−\mathrm{2}^{−\frac{\mathrm{1}}{\mathrm{2}}} =−\frac{\mathrm{1}}{\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{2}}} }=−\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}} \\ $$$$ \\ $$

Commented by malwan last updated on 10/Sep/20

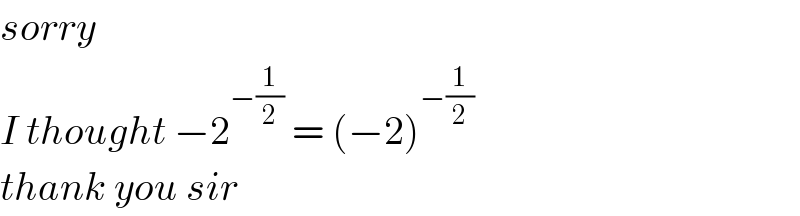
sorry I thought −2^(−(1/2)) = (−2)^(−(1/2)) thank you sir

$${sorry} \\ $$$${I}\:{thought}\:−\mathrm{2}^{−\frac{\mathrm{1}}{\mathrm{2}}} \:=\:\left(−\mathrm{2}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$${thank}\:{you}\:{sir} \\ $$

Commented by Aziztisffola last updated on 10/Sep/20

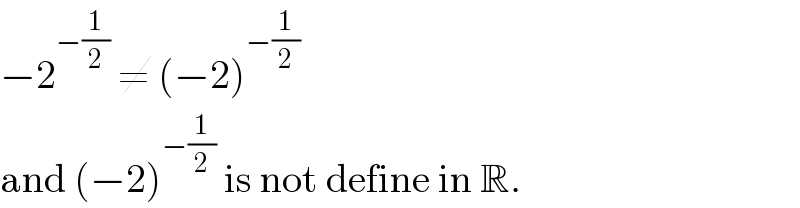
−2^(−(1/2)) ≠ (−2)^(−(1/2)) and (−2)^(−(1/2)) is not define in R.

$$−\mathrm{2}^{−\frac{\mathrm{1}}{\mathrm{2}}} \:\neq\:\left(−\mathrm{2}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$\mathrm{and}\:\left(−\mathrm{2}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \:\mathrm{is}\:\mathrm{not}\:\mathrm{define}\:\mathrm{in}\:\mathbb{R}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com