
Question Number 6716 by Tawakalitu. last updated on 15/Jul/16
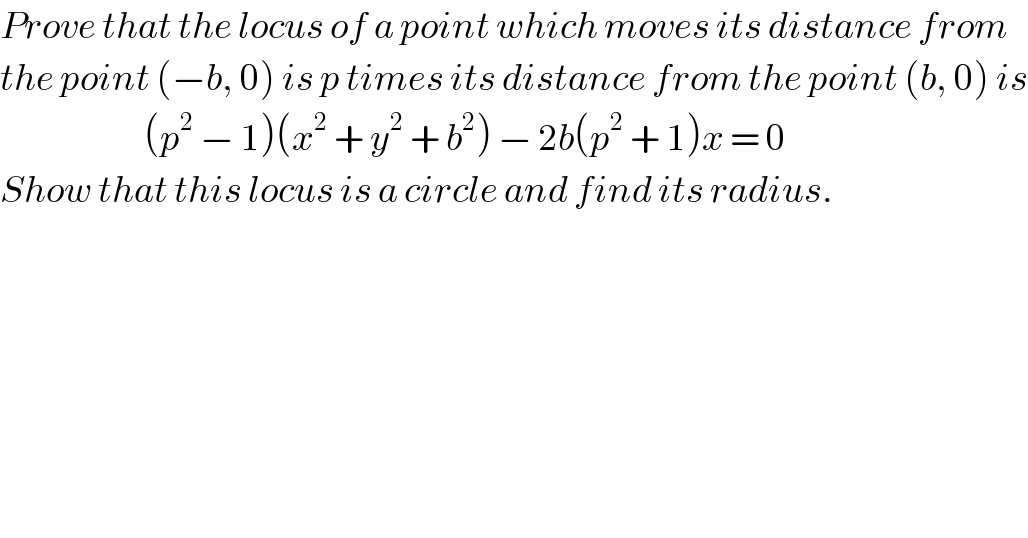
$${Prove}\:{that}\:{the}\:{locus}\:{of}\:{a}\:{point}\:{which}\:{moves}\:{its}\:{distance}\:{from}\: \\ $$$${the}\:{point}\:\left(−{b},\:\mathrm{0}\right)\:{is}\:{p}\:{times}\:{its}\:{distance}\:{from}\:{the}\:{point}\:\left({b},\:\mathrm{0}\right)\:{is} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left({p}^{\mathrm{2}} \:−\:\mathrm{1}\right)\left({x}^{\mathrm{2}} \:+\:{y}^{\mathrm{2}} \:+\:{b}^{\mathrm{2}} \right)\:−\:\mathrm{2}{b}\left({p}^{\mathrm{2}} \:+\:\mathrm{1}\right){x}\:=\:\mathrm{0} \\ $$$${Show}\:{that}\:{this}\:{locus}\:{is}\:{a}\:{circle}\:{and}\:{find}\:{its}\:{radius}. \\ $$
Answered by Yozzii last updated on 16/Jul/16
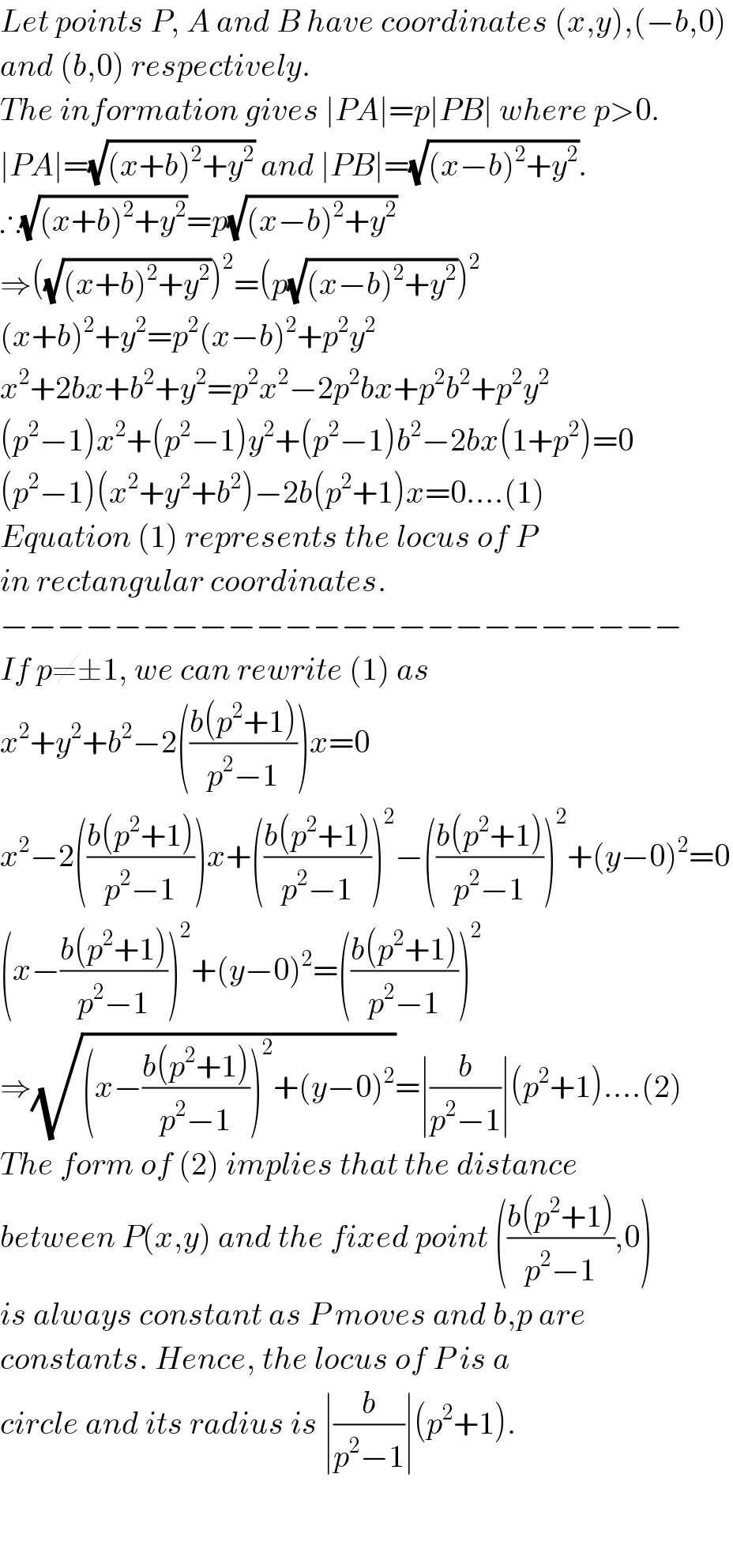
$${Let}\:{points}\:{P},\:{A}\:{and}\:{B}\:{have}\:{coordinates}\:\left({x},{y}\right),\left(−{b},\mathrm{0}\right)\: \\ $$$${and}\:\left({b},\mathrm{0}\right)\:{respectively}. \\ $$$${The}\:{information}\:{gives}\:\mid{PA}\mid={p}\mid{PB}\mid\:{where}\:{p}>\mathrm{0}. \\ $$$$\mid{PA}\mid=\sqrt{\left({x}+{b}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} }\:{and}\:\mid{PB}\mid=\sqrt{\left({x}−{b}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} }. \\ $$$$\therefore\sqrt{\left({x}+{b}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} }={p}\sqrt{\left({x}−{b}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} } \\ $$$$\Rightarrow\left(\sqrt{\left({x}+{b}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} }\right)^{\mathrm{2}} =\left({p}\sqrt{\left({x}−{b}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} }\right)^{\mathrm{2}} \\ $$$$\left({x}+{b}\right)^{\mathrm{2}} +{y}^{\mathrm{2}} ={p}^{\mathrm{2}} \left({x}−{b}\right)^{\mathrm{2}} +{p}^{\mathrm{2}} {y}^{\mathrm{2}} \\ $$$${x}^{\mathrm{2}} +\mathrm{2}{bx}+{b}^{\mathrm{2}} +{y}^{\mathrm{2}} ={p}^{\mathrm{2}} {x}^{\mathrm{2}} −\mathrm{2}{p}^{\mathrm{2}} {bx}+{p}^{\mathrm{2}} {b}^{\mathrm{2}} +{p}^{\mathrm{2}} {y}^{\mathrm{2}} \\ $$$$\left({p}^{\mathrm{2}} −\mathrm{1}\right){x}^{\mathrm{2}} +\left({p}^{\mathrm{2}} −\mathrm{1}\right){y}^{\mathrm{2}} +\left({p}^{\mathrm{2}} −\mathrm{1}\right){b}^{\mathrm{2}} −\mathrm{2}{bx}\left(\mathrm{1}+{p}^{\mathrm{2}} \right)=\mathrm{0} \\ $$$$\left({p}^{\mathrm{2}} −\mathrm{1}\right)\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)−\mathrm{2}{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right){x}=\mathrm{0}....\left(\mathrm{1}\right) \\ $$$${Equation}\:\left(\mathrm{1}\right)\:{represents}\:{the}\:{locus}\:{of}\:{P} \\ $$$${in}\:{rectangular}\:{coordinates}.\: \\ $$$$−−−−−−−−−−−−−−−−−−−−−−−− \\ $$$${If}\:{p}\neq\pm\mathrm{1},\:{we}\:{can}\:{rewrite}\:\left(\mathrm{1}\right)\:{as} \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{b}^{\mathrm{2}} −\mathrm{2}\left(\frac{{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right)}{{p}^{\mathrm{2}} −\mathrm{1}}\right){x}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} −\mathrm{2}\left(\frac{{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right)}{{p}^{\mathrm{2}} −\mathrm{1}}\right){x}+\left(\frac{{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right)}{{p}^{\mathrm{2}} −\mathrm{1}}\right)^{\mathrm{2}} −\left(\frac{{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right)}{{p}^{\mathrm{2}} −\mathrm{1}}\right)^{\mathrm{2}} +\left({y}−\mathrm{0}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\left({x}−\frac{{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right)}{{p}^{\mathrm{2}} −\mathrm{1}}\right)^{\mathrm{2}} +\left({y}−\mathrm{0}\right)^{\mathrm{2}} =\left(\frac{{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right)}{{p}^{\mathrm{2}} −\mathrm{1}}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\sqrt{\left({x}−\frac{{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right)}{{p}^{\mathrm{2}} −\mathrm{1}}\right)^{\mathrm{2}} +\left({y}−\mathrm{0}\right)^{\mathrm{2}} }=\mid\frac{{b}}{{p}^{\mathrm{2}} −\mathrm{1}}\mid\left({p}^{\mathrm{2}} +\mathrm{1}\right)....\left(\mathrm{2}\right) \\ $$$${The}\:{form}\:{of}\:\left(\mathrm{2}\right)\:{implies}\:{that}\:{the}\:{distance} \\ $$$${between}\:{P}\left({x},{y}\right)\:{and}\:{the}\:{fixed}\:{point}\:\left(\frac{{b}\left({p}^{\mathrm{2}} +\mathrm{1}\right)}{{p}^{\mathrm{2}} −\mathrm{1}},\mathrm{0}\right) \\ $$$${is}\:{always}\:{constant}\:{as}\:{P}\:{moves}\:{and}\:{b},{p}\:{are} \\ $$$${constants}.\:{Hence},\:{the}\:{locus}\:{of}\:{P}\:{is}\:{a} \\ $$$${circle}\:{and}\:{its}\:{radius}\:{is}\:\mid\frac{{b}}{{p}^{\mathrm{2}} −\mathrm{1}}\mid\left({p}^{\mathrm{2}} +\mathrm{1}\right). \\ $$$$ \\ $$$$ \\ $$
Commented by Tawakalitu. last updated on 16/Jul/16
$${Wow}\:{great}.\:{thanks} \\ $$
