
Question Number 20297 by Tinkutara last updated on 25/Aug/17
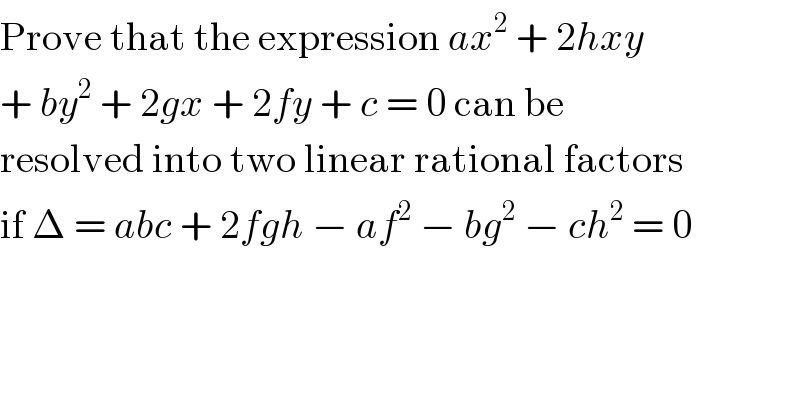
$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{the}\:\mathrm{expression}\:{ax}^{\mathrm{2}} \:+\:\mathrm{2}{hxy} \\ $$$$+\:{by}^{\mathrm{2}} \:+\:\mathrm{2}{gx}\:+\:\mathrm{2}{fy}\:+\:{c}\:=\:\mathrm{0}\:\mathrm{can}\:\mathrm{be} \\ $$$$\mathrm{resolved}\:\mathrm{into}\:\mathrm{two}\:\mathrm{linear}\:\mathrm{rational}\:\mathrm{factors} \\ $$$$\mathrm{if}\:\Delta\:=\:{abc}\:+\:\mathrm{2}{fgh}\:−\:{af}^{\mathrm{2}} \:−\:{bg}^{\mathrm{2}} \:−\:{ch}^{\mathrm{2}} \:=\:\mathrm{0} \\ $$
Answered by ajfour last updated on 25/Aug/17

$${let}\:{a}\left({x}−{x}_{\mathrm{0}} \right)^{\mathrm{2}} +{b}\left({y}−{y}_{\mathrm{0}} \right)^{\mathrm{2}} + \\ $$$$\:\mathrm{2}{h}\left({x}−{x}_{\mathrm{0}} \right)\left({y}−{y}_{\mathrm{0}} \right)={ax}^{\mathrm{2}} +{by}^{\mathrm{2}} +\mathrm{2}{hxy} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}{gx}+\mathrm{2}{fy}+{c}=\mathrm{0} \\ $$$${comparing}\:{coefficients}\:{of}\:{x}\:{and} \\ $$$${y},\:{and}\:{constant}\:{term}\:{we}\:{get}: \\ $$$$\:\:{ax}_{\mathrm{0}} +{hy}_{\mathrm{0}} =−{g}\:\:\:\:\:.....\left({i}\right) \\ $$$$\:\:{by}_{\mathrm{0}} +{hx}_{\mathrm{0}} =−{f}\:\:\:\:\:......\left({ii}\right) \\ $$$${ax}_{\mathrm{0}} ^{\mathrm{2}} +{by}_{\mathrm{0}} ^{\mathrm{2}} +\mathrm{2}{hx}_{\mathrm{0}} {y}_{\mathrm{0}} ={c}\:\:\:\left({iii}\right) \\ $$$${using}\:\left({i}\right)\:{and}\:\left({ii}\right)\:{in}\:\left({iii}\right): \\ $$$$\Rightarrow\:{x}_{\mathrm{0}} \left(−{g}−{hx}_{\mathrm{0}} \right)+{y}_{\mathrm{0}} \left(−{f}−{hx}_{\mathrm{0}} \right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\mathrm{2}{hx}_{\mathrm{0}} {y}_{\mathrm{0}} ={c} \\ $$$${or}\:\:\:\:\:{gx}_{\mathrm{0}} +{fy}_{\mathrm{0}} =−{c}\:\:\:\:\:....\left({iv}\right) \\ $$$$\left({i}\right)\:,\:\left({ii}\right),\:{and}\:\left({iv}\right)\:{form}\:{a}\:{system} \\ $$$${of}\:{three}\:{linear}\:{equations}\:{in}\:{two} \\ $$$${unknowns},\:{has}\:{unique}\:{solution}\:{only}\:{if} \\ $$$$\begin{vmatrix}{{a}}&{{h}}&{{g}}\\{{h}}&{{b}}&{{f}}\\{{g}}&{{f}}&{{c}}\end{vmatrix}=\mathrm{0} \\ $$$${first}\:{row}\:{we}\:{get}\:{from}\:{coefficients} \\ $$$${of}\:{x},\:{y},\:{and}\:{constant}\:{term}\:{of} \\ $$$${equation}\:\left({i}\right),\:\:{second}\:{row}\rightarrow\left({ii}\right) \\ $$$${third}\:{row}\:\rightarrow\:\left({iv}\right) \\ $$$$\Rightarrow{a}\left({bc}−{f}^{\mathrm{2}} \right)−{h}\left({ch}−{gf}\right)+{g}\left({hf}−{bg}\right)=\mathrm{0} \\ $$$$\Rightarrow\:\:{abc}+\mathrm{2}{fgh}−{af}^{\mathrm{2}} −{ch}^{\mathrm{2}} −{bg}^{\mathrm{2}} =\mathrm{0}\:. \\ $$
Commented by Tinkutara last updated on 25/Aug/17
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{Sir}! \\ $$
