
Question Number 193960 by pete last updated on 24/Jun/23
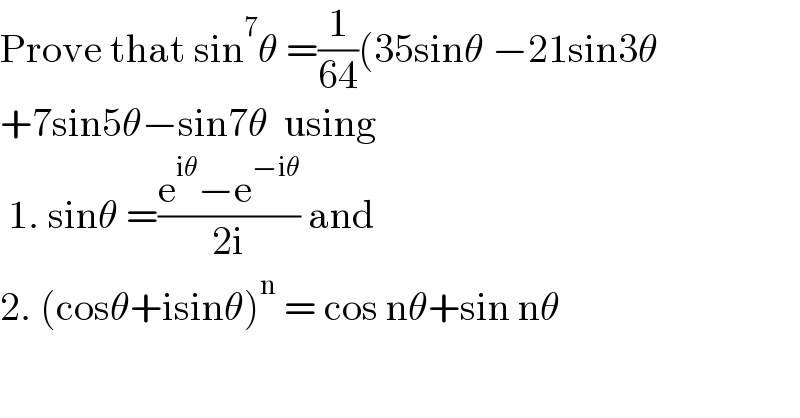
$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{sin7}\theta\:=\frac{\mathrm{1}}{\mathrm{64}}\left(\mathrm{35sin}\theta\:−\mathrm{21sin3}\theta\right. \\ $$$$+\mathrm{7sin5}\theta−\mathrm{sin7}\theta\:\:\mathrm{using} \\ $$$$\:\mathrm{1}.\:\mathrm{sin}\theta\:=\frac{\mathrm{e}^{\mathrm{i}\theta} −\mathrm{e}^{−\mathrm{i}\theta} }{\mathrm{2i}}\:\mathrm{and}\: \\ $$$$\mathrm{2}.\:\left(\mathrm{cos}\theta+\mathrm{isin}\theta\right)^{\mathrm{n}} \:=\:\mathrm{cos}\:\mathrm{n}\theta+\mathrm{sin}\:\mathrm{n}\theta \\ $$$$ \\ $$
