
Question Number 69231 by ~ À ® @ 237 ~ last updated on 21/Sep/19
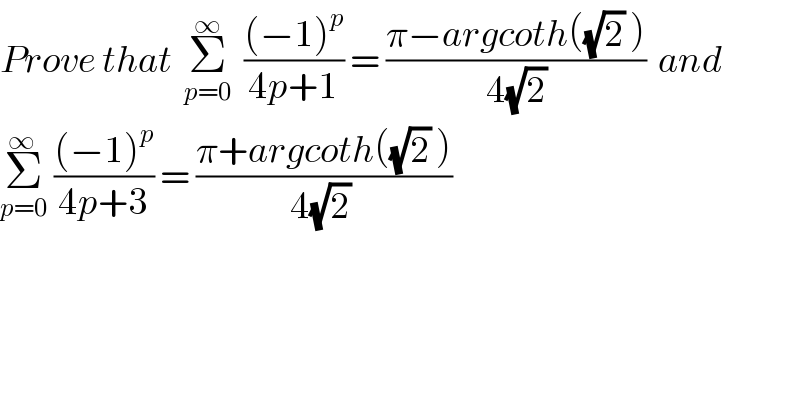
$${Prove}\:{that}\:\:\underset{{p}=\mathrm{0}} {\overset{\infty} {\sum}}\:\:\frac{\left(−\mathrm{1}\right)^{{p}} }{\mathrm{4}{p}+\mathrm{1}}\:=\:\frac{\pi−{argcoth}\left(\sqrt{\mathrm{2}}\:\right)}{\mathrm{4}\sqrt{\mathrm{2}}}\:\:{and} \\ $$$$\underset{{p}=\mathrm{0}} {\overset{\infty\:} {\sum}}\:\frac{\left(−\mathrm{1}\right)^{{p}} }{\mathrm{4}{p}+\mathrm{3}}\:=\:\frac{\pi+{argcoth}\left(\sqrt{\mathrm{2}}\:\right)}{\mathrm{4}\sqrt{\mathrm{2}}\:}\:\:\: \\ $$
Commented by mathmax by abdo last updated on 21/Sep/19
![let s(x)=Σ_(n=0) ^∞ (((−1)^n )/(4n+1))x^(4n+1) with ∣x∣<1 ⇒s^′ (x)=Σ_(n=0) ^∞ (−1)^n x^(4n) =Σ_(n=0) ^∞ (−x^4 )^n =(1/(1+x^4 )) ⇒s(x) =∫_0 ^x (dt/(1+t^4 )) +c c=s(0)=0 ⇒s(x) =∫_0 ^x (dt/(1+t^4 )) and Σ_(n=0) ^∞ (((−1)^n )/(4n+1)) =s(1)=∫_0 ^1 (dt/(t^4 +1)) let decompose F(t)=(1/(t^4 +1)) ⇒F(t)=(1/((t^2 +1)^2 −2t^2 )) =(1/((t^2 +1+(√2)t)(t^2 +1−(√2)t))) =((at +b)/(t^2 +(√2)t +1)) +((ct+d)/(t^2 −(√2)t +1)) F(−t)=F(t) ⇒((−at+b)/(t^2 −(√2)t +1)) +((−ct+d)/(t^2 +(√2)t +1)) =F(t) ⇒ c=−a and d=b ⇒ F(t) =((at+b)/(t^2 +(√2)t +1)) +((−at+b)/(t^2 −(√2)t +1)) F(o) =1 =2b ⇒b=(1/2) F(1) =((a+b)/(2+(√2))) +((−a+b)/(2−(√2))) =(1/2) ⇒(((2−(√2))(a+b)+(2+(√2))(−a+b))/2)=(1/2) ⇒(2−(√2))a +(2−(√2))b −(2+(√2))a+(2+(√2))b =1 ⇒ −2(√2)a +2 =1 ⇒a =(1/(2(√2))) ⇒ F(t) =(((1/(2(√2)))t +(1/2))/(t^2 +(√2)t +1)) +((−(1/(2(√2)))t+(1/2))/(t^2 −(√2)t +1)) =(1/(2(√2))){ ((t+(√2))/(t^2 +(√2)t+1)) −((t−(√2))/(t^2 −(√2)t +1))} ⇒ ∫_0 ^1 F(t)dt =(1/(4(√2))) ∫_0 ^1 ((2t+(√2)+(√2))/(t^2 +(√2)t +1))dt −(1/(4(√2))) ∫_0 ^1 ((2t−(√2)−(√2))/(t^2 −(√2)t +1))dt =(1/(4(√2)))[ln(t^2 +(√2)t +1)]_0 ^(1 ) +(1/4) ∫_0 ^1 (dt/(t^2 +(√2)t +1)) −(1/(4(√2))) [ln(t^2 −(√2)t +1)]_0 ^1 +(1/4) ∫_0 ^1 (dt/(t^2 −(√2)t +1)) =(1/(4(√2))){ln(2+(√2))−ln(2−(√2)) +(1/4) { ∫_0 ^1 (...)dt +∫_0 ^1 (....)dt} ∫_0 ^1 (dt/(t^2 +(√2)t +1)) =∫_0 ^1 (dt/(t^2 +2((√2)/2)t +(1/2)+(1/2))) =∫_0 ^1 (dt/((t+(1/(√2)))^(2 ) +(1/2))) =_(t+(1/(√2))=(u/(√2))) 2∫_1 ^(1+(√2)) (1/(u^2 +1)) (du/(√2)) =(√2)[arctan(u)]_1 ^(1+(√2)) =(√2){arctan(1+(√2))−(π/4)} =(√2){(π/2) −arctan((√2)−1)−(π/4)} =(√2){(π/4)−(π/8)} =(√2)×(π/8) ∫_0 ^1 (dt/(t^2 −(√2)t +1)) =∫_0 ^1 (dt/((t−(1/(√2)))^2 +(1/2))) =_(t−(1/(√2))=(u/(√2))) 2 ∫_(−1) ^((√2)−1) (1/(u^2 +1))(du/(√2)) =(√2)[arctan(u)]_(−1) ^((√2)−1) =(√2){(π/8) +(π/4)} =(√2)×((3π)/8) ⇒ ∫_0 ^1 F(t)dt =(1/(4(√2)))ln(((2+(√2))/(2−(√2)))) +(1/4){((π(√2))/8) +((3π(√2))/8)} =(1/(4(√2)))ln(((4+4(√2)+2)/2))+(1/4)((π(√2))/2) =(1/(4(√2)))ln(3+2(√2))+((π(√2))/8) ⇒ Σ_(n=0) ^∞ (((−1)^n )/(4n+1)) =(1/(4(√2)))ln(3+2(√2)) +((π(√2))/8)](Q69250.png)
$${let}\:{s}\left({x}\right)=\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{4}{n}+\mathrm{1}}{x}^{\mathrm{4}{n}+\mathrm{1}} \:{with}\:\:\mid{x}\mid<\mathrm{1}\:\Rightarrow{s}^{'} \left({x}\right)=\sum_{{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} {x}^{\mathrm{4}{n}} \\ $$$$=\sum_{{n}=\mathrm{0}} ^{\infty} \left(−{x}^{\mathrm{4}} \right)^{{n}} \:=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{4}} }\:\Rightarrow{s}\left({x}\right)\:=\int_{\mathrm{0}} ^{{x}} \:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{4}} }\:+{c} \\ $$$${c}={s}\left(\mathrm{0}\right)=\mathrm{0}\:\Rightarrow{s}\left({x}\right)\:=\int_{\mathrm{0}} ^{{x}} \:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{4}} }\:{and}\:\sum_{{n}=\mathrm{0}} ^{\infty} \frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{4}{n}+\mathrm{1}}\:={s}\left(\mathrm{1}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}^{\mathrm{4}} \:+\mathrm{1}} \\ $$$${let}\:{decompose}\:{F}\left({t}\right)=\frac{\mathrm{1}}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:\Rightarrow{F}\left({t}\right)=\frac{\mathrm{1}}{\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}{t}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{\left({t}^{\mathrm{2}} +\mathrm{1}+\sqrt{\mathrm{2}}{t}\right)\left({t}^{\mathrm{2}} \:+\mathrm{1}−\sqrt{\mathrm{2}}{t}\right)}\:=\frac{{at}\:+{b}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:+\frac{{ct}+{d}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$${F}\left(−{t}\right)={F}\left({t}\right)\:\Rightarrow\frac{−{at}+{b}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:+\frac{−{ct}+{d}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:={F}\left({t}\right)\:\Rightarrow \\ $$$${c}=−{a}\:\:{and}\:{d}={b}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{{at}+{b}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:+\frac{−{at}+{b}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$${F}\left({o}\right)\:=\mathrm{1}\:=\mathrm{2}{b}\:\Rightarrow{b}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${F}\left(\mathrm{1}\right)\:=\frac{{a}+{b}}{\mathrm{2}+\sqrt{\mathrm{2}}}\:+\frac{−{a}+{b}}{\mathrm{2}−\sqrt{\mathrm{2}}}\:=\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\frac{\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)\left({a}+{b}\right)+\left(\mathrm{2}+\sqrt{\mathrm{2}}\right)\left(−{a}+{b}\right)}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow\left(\mathrm{2}−\sqrt{\mathrm{2}}\right){a}\:+\left(\mathrm{2}−\sqrt{\mathrm{2}}\right){b}\:−\left(\mathrm{2}+\sqrt{\mathrm{2}}\right){a}+\left(\mathrm{2}+\sqrt{\mathrm{2}}\right){b}\:=\mathrm{1}\:\Rightarrow \\ $$$$−\mathrm{2}\sqrt{\mathrm{2}}{a}\:+\mathrm{2}\:=\mathrm{1}\:\Rightarrow{a}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\frac{\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{t}\:+\frac{\mathrm{1}}{\mathrm{2}}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:+\frac{−\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}{t}+\frac{\mathrm{1}}{\mathrm{2}}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\left\{\:\:\:\frac{{t}+\sqrt{\mathrm{2}}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}+\mathrm{1}}\:−\frac{{t}−\sqrt{\mathrm{2}}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\right\}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:{F}\left({t}\right){dt}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{2}{t}+\sqrt{\mathrm{2}}+\sqrt{\mathrm{2}}}{{t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}{dt}\:−\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{2}{t}−\sqrt{\mathrm{2}}−\sqrt{\mathrm{2}}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\left[{ln}\left({t}^{\mathrm{2}} +\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)\right]_{\mathrm{0}} ^{\mathrm{1}\:} \:\:+\frac{\mathrm{1}}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$$−\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\:\left[{ln}\left({t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} \:+\frac{\mathrm{1}}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}\left\{{ln}\left(\mathrm{2}+\sqrt{\mathrm{2}}\right)−{ln}\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)\:+\frac{\mathrm{1}}{\mathrm{4}}\:\left\{\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\left(...\right){dt}\:+\int_{\mathrm{0}} ^{\mathrm{1}} \left(....\right){dt}\right\}\right. \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{2}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}{t}\:\:+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{\left({t}+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)^{\mathrm{2}\:} +\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$$=_{{t}+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}=\frac{{u}}{\sqrt{\mathrm{2}}}} \:\:\:\:\mathrm{2}\int_{\mathrm{1}} ^{\mathrm{1}+\sqrt{\mathrm{2}}} \:\:\:\frac{\mathrm{1}}{{u}^{\mathrm{2}} \:+\mathrm{1}}\:\frac{{du}}{\sqrt{\mathrm{2}}}\:=\sqrt{\mathrm{2}}\left[{arctan}\left({u}\right)\right]_{\mathrm{1}} ^{\mathrm{1}+\sqrt{\mathrm{2}}} \\ $$$$=\sqrt{\mathrm{2}}\left\{{arctan}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)−\frac{\pi}{\mathrm{4}}\right\}\:=\sqrt{\mathrm{2}}\left\{\frac{\pi}{\mathrm{2}}\:−{arctan}\left(\sqrt{\mathrm{2}}−\mathrm{1}\right)−\frac{\pi}{\mathrm{4}}\right\} \\ $$$$=\sqrt{\mathrm{2}}\left\{\frac{\pi}{\mathrm{4}}−\frac{\pi}{\mathrm{8}}\right\}\:=\sqrt{\mathrm{2}}×\frac{\pi}{\mathrm{8}} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}^{\mathrm{2}} −\sqrt{\mathrm{2}}{t}\:+\mathrm{1}}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{dt}}{\left({t}−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)^{\mathrm{2}} \:+\frac{\mathrm{1}}{\mathrm{2}}}\:=_{{t}−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}=\frac{{u}}{\sqrt{\mathrm{2}}}} \mathrm{2}\:\:\int_{−\mathrm{1}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \:\frac{\mathrm{1}}{{u}^{\mathrm{2}} \:+\mathrm{1}}\frac{{du}}{\sqrt{\mathrm{2}}} \\ $$$$=\sqrt{\mathrm{2}}\left[{arctan}\left({u}\right)\right]_{−\mathrm{1}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \:=\sqrt{\mathrm{2}}\left\{\frac{\pi}{\mathrm{8}}\:+\frac{\pi}{\mathrm{4}}\right\}\:=\sqrt{\mathrm{2}}×\frac{\mathrm{3}\pi}{\mathrm{8}}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:{F}\left({t}\right){dt}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}{ln}\left(\frac{\mathrm{2}+\sqrt{\mathrm{2}}}{\mathrm{2}−\sqrt{\mathrm{2}}}\right)\:+\frac{\mathrm{1}}{\mathrm{4}}\left\{\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{8}}\:+\frac{\mathrm{3}\pi\sqrt{\mathrm{2}}}{\mathrm{8}}\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}{ln}\left(\frac{\mathrm{4}+\mathrm{4}\sqrt{\mathrm{2}}+\mathrm{2}}{\mathrm{2}}\right)+\frac{\mathrm{1}}{\mathrm{4}}\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{2}}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)+\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{8}}\:\Rightarrow \\ $$$$\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{4}{n}+\mathrm{1}}\:=\frac{\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}}{ln}\left(\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}\right)\:+\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{8}} \\ $$
