
Question Number 2980 by Filup last updated on 02/Dec/15
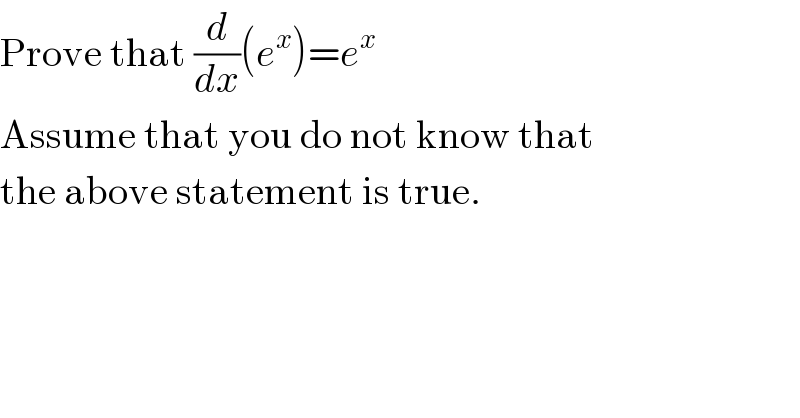
$$\mathrm{Prove}\:\mathrm{that}\:\frac{{d}}{{dx}}\left({e}^{{x}} \right)={e}^{{x}} \\ $$$$\mathrm{Assume}\:\mathrm{that}\:\mathrm{you}\:\mathrm{do}\:\mathrm{not}\:\mathrm{know}\:\mathrm{that} \\ $$$$\mathrm{the}\:\mathrm{above}\:\mathrm{statement}\:\mathrm{is}\:\mathrm{true}. \\ $$
Answered by RasheedAhmad last updated on 02/Dec/15
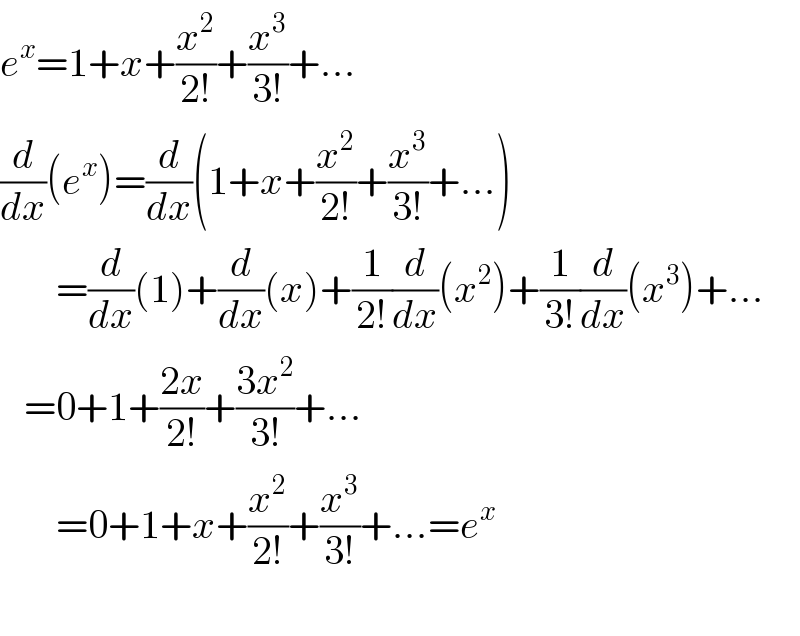
$${e}^{{x}} =\mathrm{1}+{x}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}!}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+... \\ $$$$\frac{{d}}{{dx}}\left({e}^{{x}} \right)=\frac{{d}}{{dx}}\left(\mathrm{1}+{x}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}!}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+...\right) \\ $$$$\:\:\:\:\:\:\:=\frac{{d}}{{dx}}\left(\mathrm{1}\right)+\frac{{d}}{{dx}}\left({x}\right)+\frac{\mathrm{1}}{\mathrm{2}!}\frac{{d}}{{dx}}\left({x}^{\mathrm{2}} \right)+\frac{\mathrm{1}}{\mathrm{3}!}\frac{{d}}{{dx}}\left({x}^{\mathrm{3}} \right)+... \\ $$$$\:\:\:=\mathrm{0}+\mathrm{1}+\frac{\mathrm{2}{x}}{\mathrm{2}!}+\frac{\mathrm{3}{x}^{\mathrm{2}} }{\mathrm{3}!}+... \\ $$$$\:\:\:\:\:\:\:=\mathrm{0}+\mathrm{1}+{x}+\frac{{x}^{\mathrm{2}} }{\mathrm{2}!}+\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+...={e}^{{x}} \\ $$$$ \\ $$
Answered by 123456 last updated on 02/Dec/15
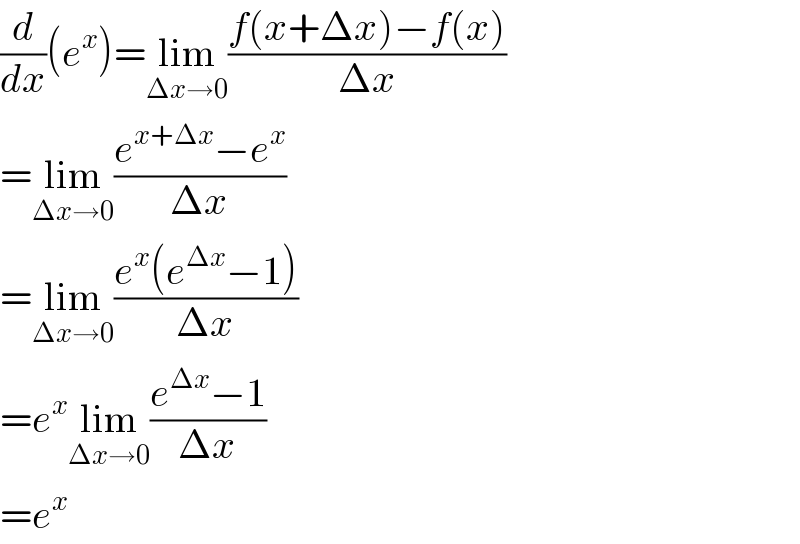
$$\frac{{d}}{{dx}}\left({e}^{{x}} \right)=\underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{f}\left({x}+\Delta{x}\right)−{f}\left({x}\right)}{\Delta{x}} \\ $$$$=\underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{x}+\Delta{x}} −{e}^{{x}} }{\Delta{x}} \\ $$$$=\underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{x}} \left({e}^{\Delta{x}} −\mathrm{1}\right)}{\Delta{x}} \\ $$$$={e}^{{x}} \underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{\Delta{x}} −\mathrm{1}}{\Delta{x}} \\ $$$$={e}^{{x}} \\ $$
Commented by 123456 last updated on 02/Dec/15

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{a}^{{x}} −\mathrm{1}}{{x}}=\mathrm{ln}\:{a} \\ $$
Commented by Filup last updated on 02/Dec/15
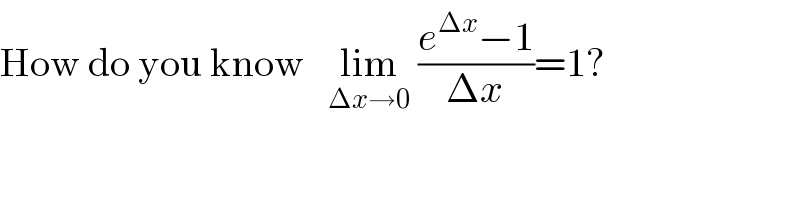
$$\mathrm{How}\:\mathrm{do}\:\mathrm{you}\:\mathrm{know}\:\:\:\underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{e}^{\Delta{x}} −\mathrm{1}}{\Delta{x}}=\mathrm{1}? \\ $$
Commented by Yozzi last updated on 03/Dec/15

$${Let}\:\underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{\Delta{x}} −\mathrm{1}}{\Delta{x}}={l}.\:{Using}\:{the}\:{Maclaurin} \\ $$$${series}\:{expansion}\:{for}\:{e}^{\Delta{x}\:} {we}\:{have} \\ $$$${e}^{\Delta{x}} =\mathrm{1}+\Delta{x}+\frac{\left(\Delta{x}\right)^{\mathrm{2}} }{\mathrm{2}}+\frac{\left(\Delta{x}\right)^{\mathrm{3}} }{\mathrm{6}}+\frac{\left(\Delta{x}\right)^{\mathrm{4}} }{\mathrm{24}}+... \\ $$$$\Rightarrow{e}^{\Delta{x}} −\mathrm{1}=\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\Delta{x}\right)^{{r}} }{{r}!} \\ $$$$\Rightarrow\frac{{e}^{\Delta{x}} −\mathrm{1}}{\Delta{x}}=\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\Delta{x}\right)^{{r}−\mathrm{1}} }{{r}!} \\ $$$${So},\:\underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{\Delta{x}} −\mathrm{1}}{\Delta{x}}=\underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(\Delta{x}\right)^{{r}−\mathrm{1}} }{{r}!} \\ $$$${l}=\underset{\Delta{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{1}}+\underset{{r}=\mathrm{2}} {\overset{\infty} {\sum}}\frac{\left(\Delta{x}\right)^{{r}−\mathrm{1}} }{{r}!} \\ $$$$ \\ $$
Commented by Filup last updated on 03/Dec/15

$$\mathrm{Thats}\:\mathrm{true},\:{but}\:\mathrm{to}\:\mathrm{derive}\:\mathrm{thus}\:\mathrm{series}\:\mathrm{you} \\ $$$$\mathrm{must}\:\mathrm{take}\:\mathrm{the}\:\mathrm{derivative}\:\mathrm{of}\:{e}^{{x}} ...? \\ $$
Commented by Yozzi last updated on 03/Dec/15
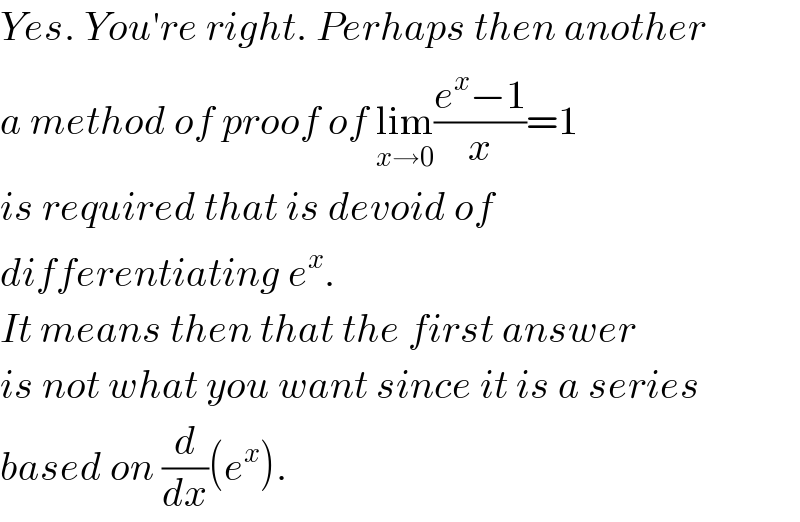
$${Yes}.\:{You}'{re}\:{right}.\:{Perhaps}\:{then}\:{another} \\ $$$${a}\:{method}\:{of}\:{proof}\:{of}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{x}} −\mathrm{1}}{{x}}=\mathrm{1} \\ $$$${is}\:{required}\:{that}\:{is}\:{devoid}\:{of}\: \\ $$$${differentiating}\:{e}^{{x}} . \\ $$$${It}\:{means}\:{then}\:{that}\:{the}\:{first}\:{answer} \\ $$$${is}\:{not}\:{what}\:{you}\:{want}\:{since}\:{it}\:{is}\:{a}\:{series} \\ $$$${based}\:{on}\:\frac{{d}}{{dx}}\left({e}^{{x}} \right).\: \\ $$
Commented by Yozzi last updated on 03/Dec/15
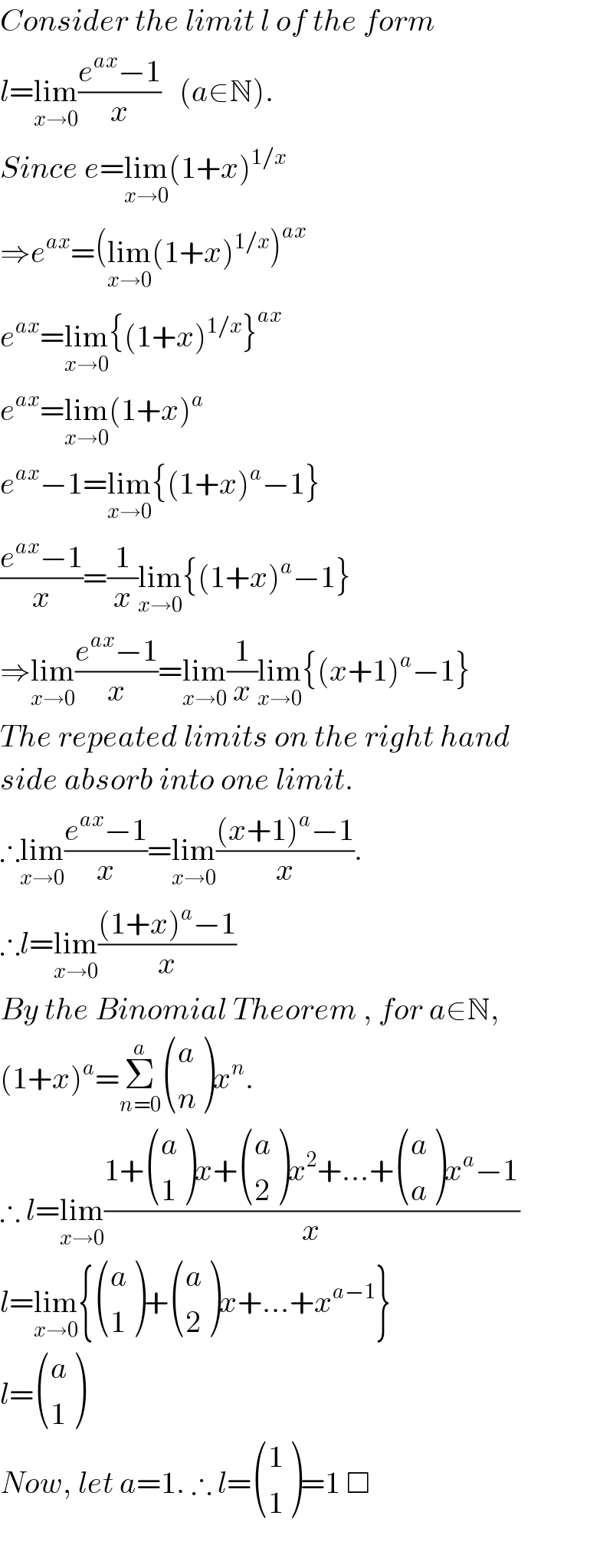
$${Consider}\:{the}\:{limit}\:{l}\:{of}\:{the}\:{form} \\ $$$${l}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{ax}} −\mathrm{1}}{{x}}\:\:\:\left({a}\in\mathbb{N}\right). \\ $$$${Since}\:{e}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\mathrm{1}+{x}\right)^{\mathrm{1}/{x}} \\ $$$$\Rightarrow{e}^{{ax}} =\left(\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\mathrm{1}+{x}\right)^{\mathrm{1}/{x}} \right)^{{ax}} \\ $$$${e}^{{ax}} =\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\left(\mathrm{1}+{x}\right)^{\mathrm{1}/{x}} \right\}^{{ax}} \\ $$$${e}^{{ax}} =\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\mathrm{1}+{x}\right)^{{a}} \\ $$$${e}^{{ax}} −\mathrm{1}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\left(\mathrm{1}+{x}\right)^{{a}} −\mathrm{1}\right\} \\ $$$$\frac{{e}^{{ax}} −\mathrm{1}}{{x}}=\frac{\mathrm{1}}{{x}}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\left(\mathrm{1}+{x}\right)^{{a}} −\mathrm{1}\right\} \\ $$$$\Rightarrow\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{ax}} −\mathrm{1}}{{x}}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{{x}}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\left({x}+\mathrm{1}\right)^{{a}} −\mathrm{1}\right\} \\ $$$${The}\:{repeated}\:{limits}\:{on}\:{the}\:{right}\:{hand} \\ $$$${side}\:{absorb}\:{into}\:{one}\:{limit}. \\ $$$$\therefore\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{ax}} −\mathrm{1}}{{x}}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left({x}+\mathrm{1}\right)^{{a}} −\mathrm{1}}{{x}}. \\ $$$$\therefore{l}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left(\mathrm{1}+{x}\right)^{{a}} −\mathrm{1}}{{x}} \\ $$$${By}\:{the}\:{Binomial}\:{Theorem}\:,\:{for}\:{a}\in\mathbb{N}, \\ $$$$\left(\mathrm{1}+{x}\right)^{{a}} =\underset{{n}=\mathrm{0}} {\overset{{a}} {\sum}}\begin{pmatrix}{{a}}\\{{n}}\end{pmatrix}{x}^{{n}} . \\ $$$$\therefore\:{l}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}+\begin{pmatrix}{{a}}\\{\mathrm{1}}\end{pmatrix}{x}+\begin{pmatrix}{{a}}\\{\mathrm{2}}\end{pmatrix}{x}^{\mathrm{2}} +...+\begin{pmatrix}{{a}}\\{{a}}\end{pmatrix}{x}^{{a}} −\mathrm{1}}{{x}} \\ $$$${l}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\begin{pmatrix}{{a}}\\{\mathrm{1}}\end{pmatrix}+\begin{pmatrix}{{a}}\\{\mathrm{2}}\end{pmatrix}{x}+...+{x}^{{a}−\mathrm{1}} \right\} \\ $$$${l}=\begin{pmatrix}{{a}}\\{\mathrm{1}}\end{pmatrix} \\ $$$${Now},\:{let}\:{a}=\mathrm{1}.\:\therefore\:{l}=\begin{pmatrix}{\mathrm{1}}\\{\mathrm{1}}\end{pmatrix}=\mathrm{1}\:\Box \\ $$$$ \\ $$
Commented by Yozzi last updated on 03/Dec/15
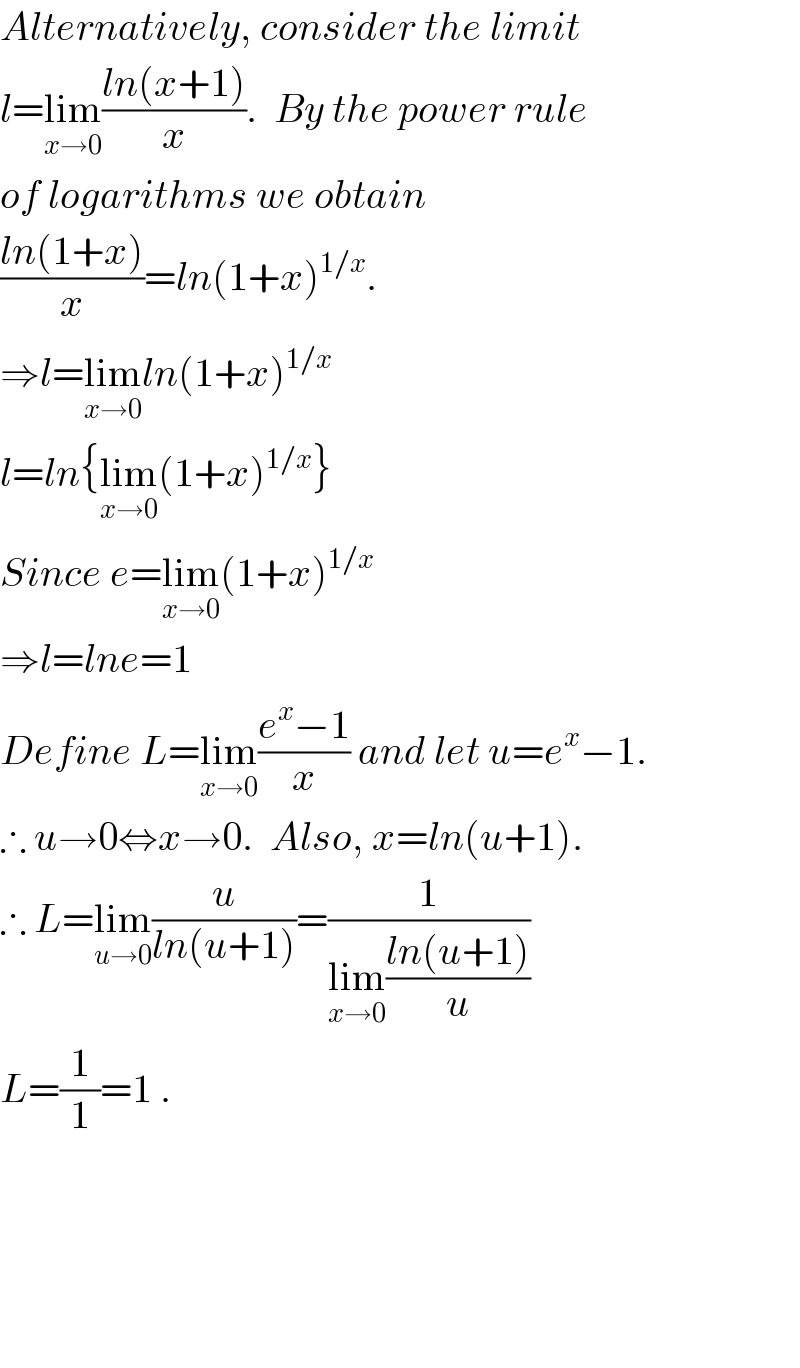
$${Alternatively},\:{consider}\:{the}\:{limit} \\ $$$${l}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{ln}\left({x}+\mathrm{1}\right)}{{x}}.\:\:{By}\:{the}\:{power}\:{rule} \\ $$$${of}\:{logarithms}\:{we}\:{obtain}\: \\ $$$$\frac{{ln}\left(\mathrm{1}+{x}\right)}{{x}}={ln}\left(\mathrm{1}+{x}\right)^{\mathrm{1}/{x}} . \\ $$$$\Rightarrow{l}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}{ln}\left(\mathrm{1}+{x}\right)^{\mathrm{1}/{x}} \\ $$$${l}={ln}\left\{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\mathrm{1}+{x}\right)^{\mathrm{1}/{x}} \right\} \\ $$$${Since}\:{e}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\mathrm{1}+{x}\right)^{\mathrm{1}/{x}} \\ $$$$\Rightarrow{l}={lne}=\mathrm{1} \\ $$$${Define}\:{L}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{e}^{{x}} −\mathrm{1}}{{x}}\:{and}\:{let}\:{u}={e}^{{x}} −\mathrm{1}. \\ $$$$\therefore\:{u}\rightarrow\mathrm{0}\Leftrightarrow{x}\rightarrow\mathrm{0}.\:\:{Also},\:{x}={ln}\left({u}+\mathrm{1}\right). \\ $$$$\therefore\:{L}=\underset{{u}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{u}}{{ln}\left({u}+\mathrm{1}\right)}=\frac{\mathrm{1}}{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{{ln}\left({u}+\mathrm{1}\right)}{{u}}} \\ $$$${L}=\frac{\mathrm{1}}{\mathrm{1}}=\mathrm{1}\:. \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by Rasheed Soomro last updated on 03/Dec/15
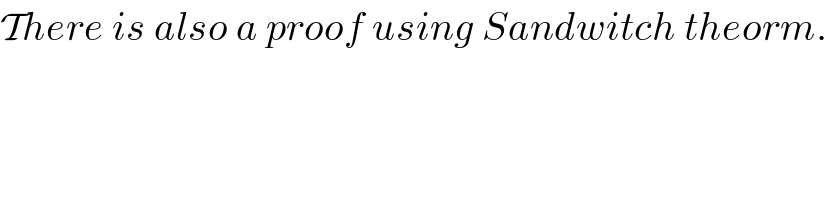
$$\mathcal{T}{here}\:{is}\:{also}\:{a}\:{proof}\:{using}\:{Sandwitch}\:{theorm}. \\ $$
Commented by Yozzi last updated on 03/Dec/15

$${Certainly}.\: \\ $$
