
Question Number 218624 by Nicholas666 last updated on 13/Apr/25
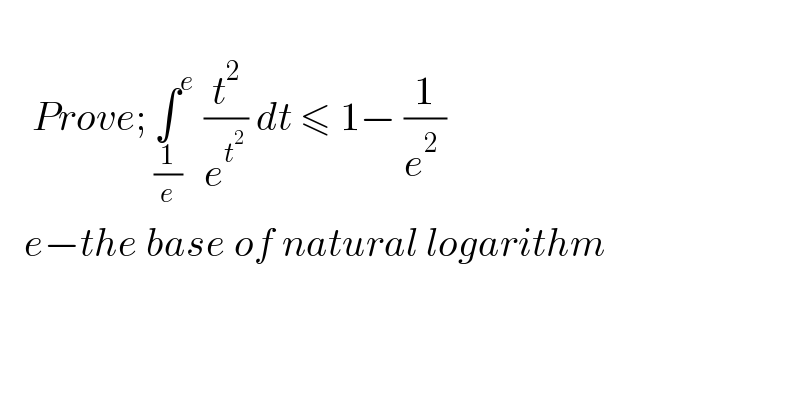
$$ \\ $$$$\:\:\:\:{Prove};\:\underset{\frac{\mathrm{1}}{{e}}} {\int}^{{e}} \:\frac{{t}^{\mathrm{2}} }{{e}^{{t}^{\mathrm{2}\:} } }\:{dt}\:\leqslant\:\mathrm{1}−\:\frac{\mathrm{1}}{{e}^{\mathrm{2}\:\:} } \\ $$$$\:\:\:{e}−{the}\:{base}\:{of}\:{natural}\:{logarithm} \\ $$$$ \\ $$
Answered by Nicholas666 last updated on 13/Apr/25
![∫_(1/e) ^e (t^2 /e^t^2 ) dt ≤1−(1/e^2 ) ⇒f′t=(d/dt)(t^2 e^(−t^2 ) )=2te^(−t^2 ) +t^2 (−2te^(−t^2 ) )= 2te^(−t^2 ) (1−t^2 ) ⇒f′(0.5)=2(0.5)e^(−(0.5)^2 ) = e^(−0.25) (1−0.25)=0.75e^(−25) >0 ⇒f′(2)=2(2)e^(−2^2 ) (1−2^2 )= 4e^(−4) (1−4)=−12e^(−4) <0 ⇒f(1)=(1^2 /e^(12) )=(1/e) ⇒∫_(1/e) ^e (t^2 /e^t^(2 ) )dt≤∫_(1/e) ^e (1/e)dt ⇒∫_(1/e) ^e (1/e)dt=(1/e)∫_(1/e) ^e 1 dt=(1/e)[t]_(1/e) ^e = (1/e)(e−(1/e))=(e^(2−1) /e^2 )=1−(1/e^2 ) ⇒∫_(1/e) ^e (t^2 /e^t^2 )dt ≤1−(1/e^2 ) Q.E.D](Q218625.png)
$$\int_{\mathrm{1}/{e}} ^{{e}} \:\frac{{t}^{\mathrm{2}} }{{e}^{{t}^{\mathrm{2}} } }\:{dt}\:\leqslant\mathrm{1}−\frac{\mathrm{1}}{{e}^{\mathrm{2}} } \\ $$$$\Rightarrow{f}'{t}=\frac{{d}}{{dt}}\left({t}^{\mathrm{2}} {e}^{−{t}^{\mathrm{2}} } \right)=\mathrm{2}{te}^{−{t}^{\mathrm{2}} } +{t}^{\mathrm{2}} \left(−\mathrm{2}{te}^{−{t}^{\mathrm{2}} } \right)= \\ $$$$\mathrm{2}{te}^{−{t}^{\mathrm{2}} } \left(\mathrm{1}−{t}^{\mathrm{2}} \right) \\ $$$$\Rightarrow{f}'\left(\mathrm{0}.\mathrm{5}\right)=\mathrm{2}\left(\mathrm{0}.\mathrm{5}\right){e}^{−\left(\mathrm{0}.\mathrm{5}\right)^{\mathrm{2}} } = \\ $$$${e}^{−\mathrm{0}.\mathrm{25}} \left(\mathrm{1}−\mathrm{0}.\mathrm{25}\right)=\mathrm{0}.\mathrm{75}{e}^{−\mathrm{25}} >\mathrm{0} \\ $$$$\Rightarrow{f}'\left(\mathrm{2}\right)=\mathrm{2}\left(\mathrm{2}\right){e}^{−\mathrm{2}^{\mathrm{2}} } \left(\mathrm{1}−\mathrm{2}^{\mathrm{2}} \right)= \\ $$$$\mathrm{4}{e}^{−\mathrm{4}} \left(\mathrm{1}−\mathrm{4}\right)=−\mathrm{12}{e}^{−\mathrm{4}} <\mathrm{0} \\ $$$$\Rightarrow{f}\left(\mathrm{1}\right)=\frac{\mathrm{1}^{\mathrm{2}} }{{e}^{\mathrm{12}} }=\frac{\mathrm{1}}{{e}} \\ $$$$\Rightarrow\int_{\mathrm{1}/{e}} ^{{e}} \frac{{t}^{\mathrm{2}} }{{e}^{{t}^{\mathrm{2}\:} } }{dt}\leqslant\int_{\mathrm{1}/{e}} ^{{e}} \frac{\mathrm{1}}{{e}}{dt} \\ $$$$\Rightarrow\int_{\mathrm{1}/{e}} ^{{e}} \frac{\mathrm{1}}{{e}}{dt}=\frac{\mathrm{1}}{{e}}\int_{\mathrm{1}/{e}} ^{{e}} \mathrm{1}\:{dt}=\frac{\mathrm{1}}{{e}}\left[{t}\right]_{\mathrm{1}/{e}} ^{{e}} \:= \\ $$$$\frac{\mathrm{1}}{{e}}\left({e}−\frac{\mathrm{1}}{{e}}\right)=\frac{{e}^{\mathrm{2}−\mathrm{1}} }{{e}^{\mathrm{2}} }=\mathrm{1}−\frac{\mathrm{1}}{{e}^{\mathrm{2}} } \\ $$$$\Rightarrow\int_{\mathrm{1}/{e}} ^{{e}} \frac{{t}^{\mathrm{2}} }{{e}^{{t}^{\mathrm{2}} } }{dt}\:\leqslant\mathrm{1}−\frac{\mathrm{1}}{{e}^{\mathrm{2}} }\:\:\:\:\:{Q}.{E}.{D} \\ $$$$ \\ $$
