
Question Number 122673 by mathocean1 last updated on 18/Nov/20
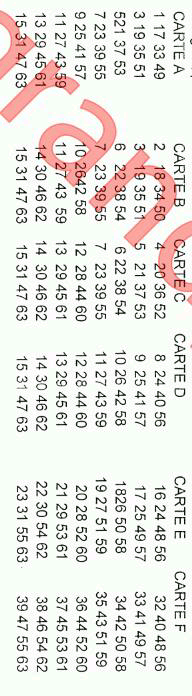
![Peter invite his son to choose secret integer n such that n ∈ [1;63] and to choose among these 6 following card (CARTE) the card which contains the choosed secret digit. i will link these cards in image. 1) How should the Peter′s son proceed to choose easily the choosed integer?](Q122673.png)
$${Peter}\:{invite}\:{his}\:{son}\:{to}\:\:{choose} \\ $$$${secret}\:{integer}\:{n}\:{such}\:{that}\: \\ $$$${n}\:\in\:\left[\mathrm{1};\mathrm{63}\right]\:{and}\:{to}\:{choose}\:{among} \\ $$$${these}\:\mathrm{6}\:{following}\:{card}\:\left({CARTE}\right) \\ $$$${the}\:{card}\:{which}\:{contains}\:{the} \\ $$$${choosed}\:{secret}\:{digit}.\:{i}\:{will} \\ $$$${link}\:{these}\:{cards}\:{in}\:{image}. \\ $$$$\left.\mathrm{1}\right)\:{How}\:{should}\:{the}\:{Peter}'{s}\:{son} \\ $$$${proceed}\:{to}\:{choose}\:{easily}\:\:{the}\: \\ $$$${choosed}\:{integer}? \\ $$$$ \\ $$
Commented by mathocean1 last updated on 20/Dec/20
a^(2000)
Answered by mathocean1 last updated on 18/Nov/20
Answered by $@y@m last updated on 19/Nov/20

$${Peter}\:{would}\:{add}\:{first}\:{integer}\:{of}\:{corresponding} \\ $$$${card}\:\left({CARTE}\right)\:{to}\:{find}\:{out}\:{the}\: \\ $$$${secret}\:{integer}\:{chosen}\:{by}\:{his}\:{son}. \\ $$
