
Permutation and CombinationQuestion and Answers: Page 2
Question Number 218129 Answers: 2 Comments: 0
Question Number 217402 Answers: 3 Comments: 0

Question Number 214923 Answers: 0 Comments: 0

Question Number 213796 Answers: 4 Comments: 0

Question Number 212686 Answers: 4 Comments: 4
Question Number 210290 Answers: 1 Comments: 0

Question Number 209281 Answers: 2 Comments: 2
Question Number 208662 Answers: 2 Comments: 0
Question Number 208263 Answers: 1 Comments: 0

Question Number 208238 Answers: 1 Comments: 0
Question Number 207937 Answers: 0 Comments: 0
Question Number 207179 Answers: 2 Comments: 0

Question Number 206827 Answers: 0 Comments: 1
$$\mathrm{log}\:\left({x}\right)\:=\:\mathrm{sin}\:\left({x}\right) \\ $$$${x}\:=\:? \\ $$
Question Number 206681 Answers: 3 Comments: 6
$$\:\: \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Question Number 206323 Answers: 0 Comments: 0

Question Number 206156 Answers: 1 Comments: 4

Question Number 205683 Answers: 1 Comments: 0
Question Number 205690 Answers: 0 Comments: 3

Question Number 205045 Answers: 0 Comments: 4
Question Number 203833 Answers: 1 Comments: 0
Question Number 203832 Answers: 2 Comments: 0
Question Number 203198 Answers: 0 Comments: 0

Question Number 202598 Answers: 2 Comments: 0

Question Number 202584 Answers: 1 Comments: 0
$$\:\: \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \_\_\_ \\ $$
Question Number 202374 Answers: 1 Comments: 2
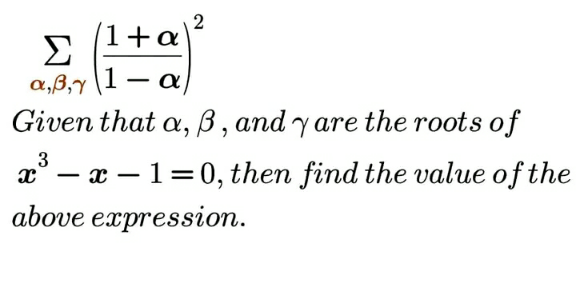
Question Number 202257 Answers: 0 Comments: 2

