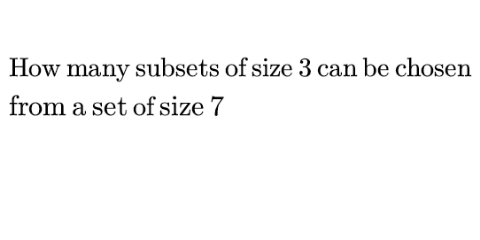Question and Answers Forum

Permutation and CombinationQuestion and Answers: Page 10

Question Number 135239 Answers: 0 Comments: 0

Question Number 135218 Answers: 2 Comments: 0

Permutation How many ways can 10 men and 7 women sit at a round table so that no 2 women are next to each other? 😎😎😎😎

$$\mathrm{Permutation} \\ $$How many ways can 10 men and 7 women sit at a round table so that no 2 women are next to each other? 😎😎😎😎

Question Number 135024 Answers: 0 Comments: 7

Question Number 134905 Answers: 2 Comments: 1

Combinatorics What is the number of ways of distributing 9 different objects among 5 persons such that each gets at least 1?

$$\mathrm{Combinatorics} \\ $$What is the number of ways of distributing 9 different objects among 5 persons such that each gets at least 1?

Question Number 134798 Answers: 3 Comments: 0

Binomial theorem Given that the coefficient of x^2 in the expansion of (1+ax) (3-2 x) ^5 is 1440, what is the value of the constant a?

$$\mathrm{Binomial}\:\mathrm{theorem} \\ $$Given that the coefficient of x^2 in the expansion of (1+ax) (3-2 x) ^5 is 1440, what is the value of the constant a?

Question Number 134644 Answers: 1 Comments: 1

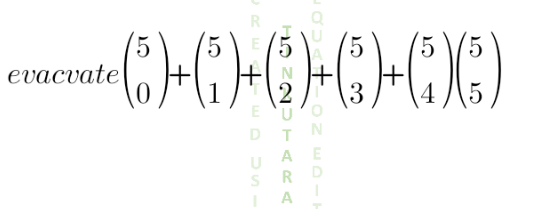
Question Number 134482 Answers: 0 Comments: 5

How many ways can this be done if you distribute 25 identical pieces of candy among five children?

$$ \\ $$How many ways can this be done if you distribute 25 identical pieces of candy among five children?

Question Number 134389 Answers: 1 Comments: 0

Eight dice are tossed. If the dice are identical in appearance , how many different−looking (distinguishable) occurrences are there?

$$\mathrm{Eight}\:\mathrm{dice}\:\mathrm{are}\:\mathrm{tossed}.\:\mathrm{If}\:\mathrm{the}\:\mathrm{dice}\:\mathrm{are}\:\mathrm{identical}\:\mathrm{in} \\ $$$$\mathrm{appearance}\:,\:\mathrm{how}\:\mathrm{many}\:\mathrm{different}−\mathrm{looking}\: \\ $$$$\left(\mathrm{distinguishable}\right)\:\mathrm{occurrences}\:\mathrm{are}\:\mathrm{there}? \\ $$

Question Number 134329 Answers: 2 Comments: 0

How many ways can 15 basketball players be assigned to Team A, Team B, and Team C with 5 players on each team?

$$ \\ $$How many ways can 15 basketball players be assigned to Team A, Team B, and Team C with 5 players on each team?

Question Number 134292 Answers: 1 Comments: 0

The digits 1, 2, 3, 4, 5, 6, and 7 are written on separate cards. Three are drawn and placed in order of drawing. In how many ways can numbers greater than 500 be formed?

$$ \\ $$The digits 1, 2, 3, 4, 5, 6, and 7 are written on separate cards. Three are drawn and placed in order of drawing. In how many ways can numbers greater than 500 be formed?

Question Number 134232 Answers: 2 Comments: 0

In how many ways can 5 men, 4 women, and 3 children be arranged in a row of 12 seats if the children sit together?

$$ \\ $$In how many ways can 5 men, 4 women, and 3 children be arranged in a row of 12 seats if the children sit together?

Question Number 134205 Answers: 0 Comments: 1

The numbers 1,2,3,4,5,6,7,8,9 and 10 are written around a circle in arbitrary order. We add all the numbers with their neighbours, we get 10 sums that way. What is the maximum possible value of the smallest of these sums?

$$ \\ $$The numbers 1,2,3,4,5,6,7,8,9 and 10 are written around a circle in arbitrary order. We add all the numbers with their neighbours, we get 10 sums that way. What is the maximum possible value of the smallest of these sums?

Question Number 133995 Answers: 0 Comments: 2

in how many ways can n men and n women be arranged in a row such that men and women alternate?

$${in}\:{how}\:{many}\:{ways}\:{can}\:{n}\:{men}\:{and} \\ $$$${n}\:{women}\:{be}\:{arranged}\:{in}\:{a}\:{row}\:{such} \\ $$$${that}\:{men}\:{and}\:{women}\:{alternate}? \\ $$

Question Number 133682 Answers: 0 Comments: 2

Question Number 133658 Answers: 0 Comments: 3

Question Number 133642 Answers: 4 Comments: 1

Question Number 133316 Answers: 2 Comments: 1

How many 6−letter words in which at least one letter appears more than once ,can be made from the letters in the word FLIGHT

$$\mathrm{How}\:\mathrm{many}\:\mathrm{6}−\mathrm{letter}\:\mathrm{words}\:\mathrm{in}\: \\ $$$$\mathrm{which}\:\mathrm{at}\:\mathrm{least}\:\mathrm{one}\:\mathrm{letter}\:\mathrm{appears} \\ $$$$\mathrm{more}\:\mathrm{than}\:\mathrm{once}\:,\mathrm{can}\:\mathrm{be}\:\mathrm{made}\:\mathrm{from} \\ $$$$\mathrm{the}\:\mathrm{letters}\:\mathrm{in}\:\mathrm{the}\:\mathrm{word}\:\mathrm{FLIGHT} \\ $$$$ \\ $$

Question Number 133314 Answers: 1 Comments: 0

How many rearrangements are there of the letters in the world (i) ENGINEERING (ii) MATHEMATICAL

$$\mathrm{How}\:\mathrm{many}\:\mathrm{rearrangements}\:\mathrm{are}\: \\ $$$$\mathrm{there}\:\mathrm{of}\:\mathrm{the}\:\mathrm{letters}\:\mathrm{in}\:\mathrm{the}\:\mathrm{world} \\ $$$$\left(\mathrm{i}\right)\:\mathrm{ENGINEERING} \\ $$$$\left(\mathrm{ii}\right)\:\mathrm{MATHEMATICAL}\: \\ $$

Question Number 132977 Answers: 0 Comments: 0

Show that: ^n C_r =((Π_(k=0) ^(r−1) n−k)/(r!))

$$\mathrm{Show}\:\mathrm{that}: \\ $$$$\:^{{n}} {C}_{{r}} =\frac{\underset{{k}=\mathrm{0}} {\overset{{r}−\mathrm{1}} {\prod}}{n}−{k}}{{r}!} \\ $$

Question Number 132854 Answers: 1 Comments: 0

It is required to seat 5 men and 4 women in a row so that the women occupy the even place. How many such arrangements are possible ?

$$\mathrm{It}\:\mathrm{is}\:\mathrm{required}\:\mathrm{to}\:\mathrm{seat}\:\mathrm{5}\:\mathrm{men}\:\mathrm{and}\:\mathrm{4}\:\mathrm{women}\: \\ $$$$\mathrm{in}\:\mathrm{a}\:\mathrm{row}\:\mathrm{so}\:\mathrm{that}\:\mathrm{the}\:\mathrm{women}\:\mathrm{occupy}\:\mathrm{the}\:\mathrm{even}\: \\ $$$$\mathrm{place}.\:\mathrm{How}\:\mathrm{many}\:\mathrm{such}\:\mathrm{arrangements}\:\mathrm{are} \\ $$$$\mathrm{possible}\:?\: \\ $$

Question Number 132726 Answers: 0 Comments: 0

use error fxn use polar coordinates to find ∫e^(−x^2 ) dx

$$\mathrm{use}\:\mathrm{error}\:\mathrm{fxn} \\ $$$$\mathrm{use}\:\mathrm{polar}\:\mathrm{coordinates}\:\mathrm{to}\:\mathrm{find} \\ $$$$\int\mathrm{e}^{−\mathrm{x}^{\mathrm{2}} } \mathrm{dx} \\ $$

Question Number 132439 Answers: 0 Comments: 1

it is a known that a particular machine will make product with a qualified rate of 90% when it is running well, but will do so with a qualified rate of only 30% when it is not running well. The probability that machine is running well is 75% normally . Suppose that one day the first product made by the machine is qualified. Find the probabiliy that the machine is running well at this time.

$$ \\ $$$$\mathrm{it}\:\mathrm{is}\:\mathrm{a}\:\mathrm{known}\:\mathrm{that}\:\mathrm{a}\:\mathrm{particular} \\ $$$$\mathrm{machine}\:\mathrm{will}\:\mathrm{make}\:\mathrm{product}\:\mathrm{with}\: \\ $$$$\mathrm{a}\:\mathrm{qualified}\:\mathrm{rate}\:\mathrm{of}\:\mathrm{90\%}\:\mathrm{when}\:\mathrm{it}\:\mathrm{is} \\ $$$$\mathrm{running}\:\mathrm{well},\:\:\mathrm{but}\:\mathrm{will}\:\mathrm{do}\:\mathrm{so}\:\mathrm{with}\: \\ $$$$\mathrm{a}\:\mathrm{qualified}\:\:\mathrm{rate}\:\mathrm{of}\:\mathrm{only}\:\mathrm{30\%}\:\mathrm{when}\: \\ $$$$\mathrm{it}\:\mathrm{is}\:\mathrm{not}\:\mathrm{running}\:\mathrm{well}.\:\mathrm{The} \\ $$$$\mathrm{probability}\:\mathrm{that}\:\mathrm{machine}\:\mathrm{is}\: \\ $$$$\mathrm{running}\:\mathrm{well}\:\mathrm{is}\:\mathrm{75\%}\:\mathrm{normally}\:. \\ $$$$\mathrm{Suppose}\:\mathrm{that}\:\mathrm{one}\:\mathrm{day}\:\:\mathrm{the}\:\mathrm{first}\: \\ $$$$\mathrm{product}\:\mathrm{made}\:\mathrm{by}\:\mathrm{the}\:\mathrm{machine}\:\mathrm{is}\: \\ $$$$\mathrm{qualified}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{probabiliy}\: \\ $$$$\mathrm{that}\:\mathrm{the}\:\mathrm{machine}\:\mathrm{is}\:\mathrm{running}\:\mathrm{well}\:\mathrm{at} \\ $$$$\mathrm{this}\:\mathrm{time}.\: \\ $$

Question Number 132382 Answers: 1 Comments: 0

What is the coefficient x^(10) in the expansion of (1+x^2 −x^3 )^8

$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{coefficient}\:\mathrm{x}^{\mathrm{10}} \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{expansion}\:\mathrm{of}\:\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} −\mathrm{x}^{\mathrm{3}} \right)^{\mathrm{8}} \\ $$

Question Number 132174 Answers: 0 Comments: 3

Question Number 131994 Answers: 2 Comments: 1

Question Number 131744 Answers: 1 Comments: 0

Pg 5 Pg 6 Pg 7 Pg 8 Pg 9 Pg 10 Pg 11 Pg 12 Pg 13 Pg 14

Contact: info@tinkutara.com