
OthersQuestion and Answers: Page 93
Question Number 61165 Answers: 1 Comments: 0

Question Number 61147 Answers: 1 Comments: 0
Question Number 61003 Answers: 3 Comments: 0

Question Number 60984 Answers: 2 Comments: 9
Question Number 60955 Answers: 1 Comments: 4

Question Number 60765 Answers: 0 Comments: 2
Question Number 60756 Answers: 1 Comments: 1
Question Number 60735 Answers: 3 Comments: 0
Question Number 60702 Answers: 0 Comments: 0

Question Number 60587 Answers: 2 Comments: 1
Question Number 60545 Answers: 1 Comments: 0

Question Number 60514 Answers: 1 Comments: 5
Question Number 60508 Answers: 0 Comments: 0
Question Number 60423 Answers: 1 Comments: 0

Question Number 60422 Answers: 1 Comments: 5

Question Number 60421 Answers: 1 Comments: 0

Question Number 60410 Answers: 0 Comments: 2
Question Number 60337 Answers: 0 Comments: 2
Question Number 60308 Answers: 0 Comments: 0

Question Number 60307 Answers: 0 Comments: 0
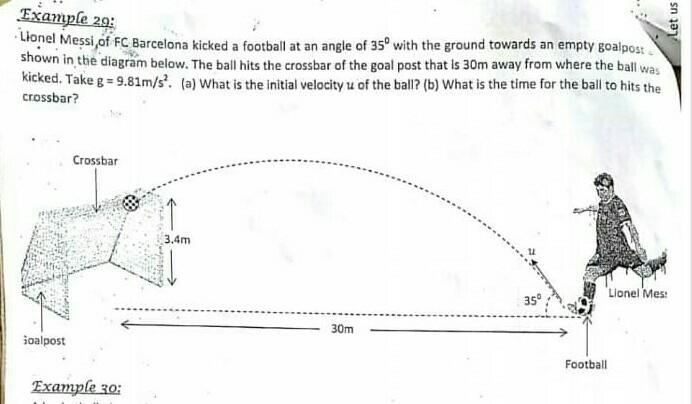
Question Number 60257 Answers: 0 Comments: 4
Question Number 60256 Answers: 1 Comments: 0

Question Number 60247 Answers: 0 Comments: 0
Question Number 60227 Answers: 0 Comments: 0
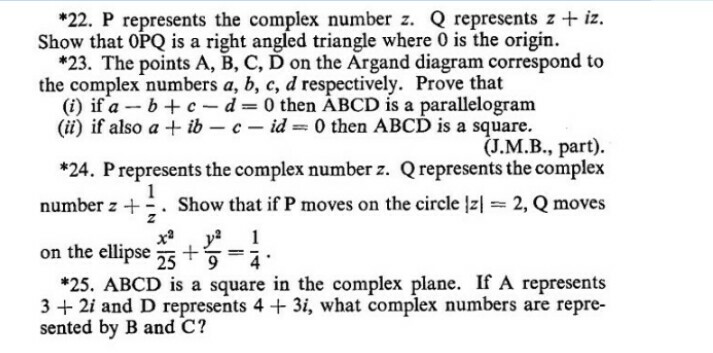
Question Number 60219 Answers: 1 Comments: 1
Question Number 60202 Answers: 0 Comments: 0
