
OthersQuestion and Answers: Page 92
Question Number 62395 Answers: 0 Comments: 1
Question Number 62341 Answers: 2 Comments: 3
Question Number 62288 Answers: 0 Comments: 0
Question Number 62241 Answers: 1 Comments: 0
Question Number 62139 Answers: 0 Comments: 0
$$\mathrm{6}+\mathrm{5}>\mathrm{3}×\mathrm{5}\:\mathrm{true}\:\mathrm{or}\:\mathrm{false} \\ $$
Question Number 62109 Answers: 1 Comments: 2
Question Number 62088 Answers: 0 Comments: 3
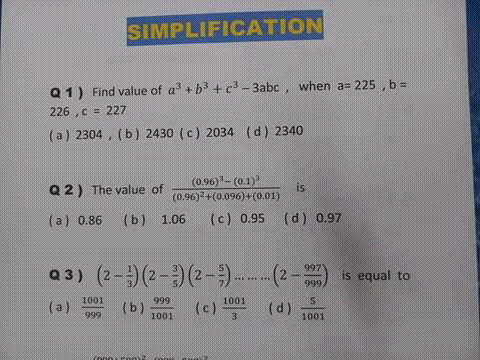
Question Number 62037 Answers: 1 Comments: 0
Question Number 62036 Answers: 0 Comments: 3
Question Number 62016 Answers: 0 Comments: 1
Question Number 61923 Answers: 1 Comments: 0
Question Number 61915 Answers: 1 Comments: 0
Question Number 61843 Answers: 0 Comments: 3
Question Number 61840 Answers: 1 Comments: 0
Question Number 61767 Answers: 0 Comments: 0

Question Number 61755 Answers: 1 Comments: 2
Question Number 61744 Answers: 0 Comments: 7
Question Number 61646 Answers: 0 Comments: 0
Question Number 61625 Answers: 1 Comments: 0
Question Number 61510 Answers: 0 Comments: 0
Question Number 61402 Answers: 0 Comments: 0

Question Number 61378 Answers: 0 Comments: 0
Question Number 61327 Answers: 1 Comments: 0

Question Number 61318 Answers: 1 Comments: 0

Question Number 61241 Answers: 5 Comments: 0

Question Number 61210 Answers: 2 Comments: 1
$${for}\:{what}\:{value}\:{of}\:\theta,\:\:{e}^{{i}\theta} =\mathrm{0}\:\: \\ $$
