
OthersQuestion and Answers: Page 84
Question Number 70132 Answers: 1 Comments: 1
$$\underset{{n}=\mathrm{1}} {\overset{\mathrm{3050}} {\sum}}\:{i}^{{n}} \\ $$
Question Number 70069 Answers: 1 Comments: 2
Question Number 70035 Answers: 0 Comments: 4

Question Number 70030 Answers: 1 Comments: 0
Question Number 70051 Answers: 2 Comments: 0
Question Number 70022 Answers: 0 Comments: 1
Question Number 70017 Answers: 0 Comments: 2
Question Number 69894 Answers: 0 Comments: 3
$$\int\:\frac{\mathrm{2}{x}^{\mathrm{5}} −{x}}{{x}^{\mathrm{3}} −\mathrm{2}}{dx} \\ $$
Question Number 69829 Answers: 2 Comments: 2

Question Number 69778 Answers: 2 Comments: 5
Question Number 69766 Answers: 0 Comments: 4
Question Number 69765 Answers: 1 Comments: 0
Question Number 69764 Answers: 1 Comments: 1
Question Number 69763 Answers: 1 Comments: 2
Question Number 69762 Answers: 1 Comments: 1
Question Number 69715 Answers: 1 Comments: 0
Question Number 69576 Answers: 1 Comments: 1
Question Number 69459 Answers: 0 Comments: 0

Question Number 69314 Answers: 1 Comments: 1
$${if}\:\mathrm{5}\:{x}\:{y}\:\mathrm{40}\:{are}\:{in}\:{GP}\:.{find}\:{x}\:{and}\:{y} \\ $$
Question Number 69247 Answers: 0 Comments: 1
$${show}\:{that}\: \\ $$$$\:{c}\mid{a}\:\Leftrightarrow\:−{c}\mid{a}. \\ $$
Question Number 69236 Answers: 0 Comments: 0
Question Number 69207 Answers: 1 Comments: 3
Question Number 69198 Answers: 0 Comments: 0
Question Number 69188 Answers: 2 Comments: 2

Question Number 69092 Answers: 1 Comments: 0
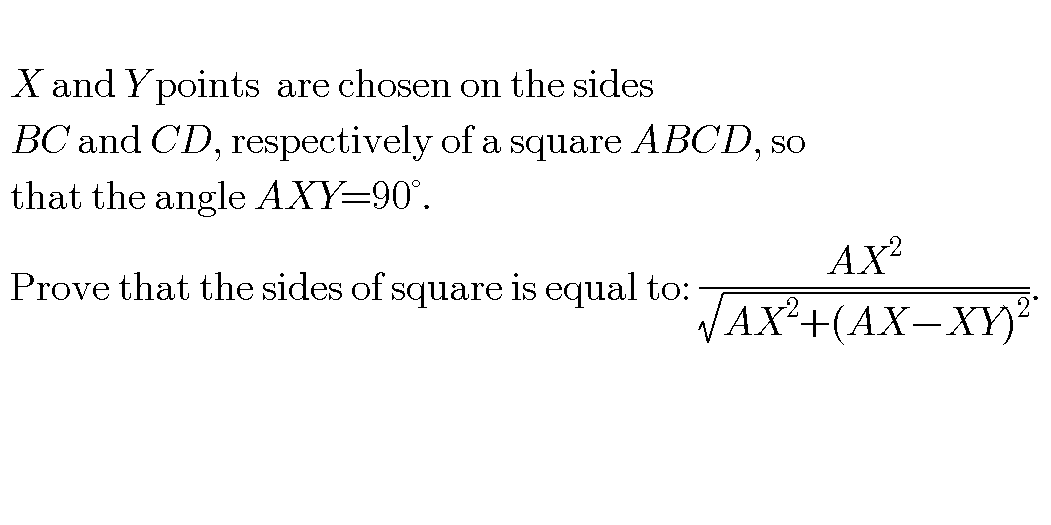
Question Number 69106 Answers: 0 Comments: 0

