Question and Answers Forum

The forces F_1 = (2i + bj) N, F_2 = (−i + 2j) N and F_3 = (ai −4j)N act through the points with position vectors r_1 = (i + 3j)m ,r_2 =(xi + 5j) m and r_3 =(−i + j)m respectively . Given that this system of forces is equivalent to a couple of magnitude 12 N m, find a) the valueof the scalars a and b b) the possible values of the scalar x.

$${The}\:{forces}\:\boldsymbol{\mathrm{F}}_{\mathrm{1}} =\:\left(\mathrm{2}\boldsymbol{{i}}\:+\:{b}\boldsymbol{{j}}\right)\:{N},\:\boldsymbol{{F}}_{\mathrm{2}} =\:\left(−\boldsymbol{{i}}\:+\:\mathrm{2}\boldsymbol{{j}}\right)\:{N} \\ $$$${and}\:\boldsymbol{{F}}_{\mathrm{3}} =\:\left({a}\boldsymbol{{i}}\:−\mathrm{4}\boldsymbol{{j}}\right){N}\:{act}\:{through}\:{the}\:{points}\:{with} \\ $$$${position}\:{vectors}\:\boldsymbol{{r}}_{\mathrm{1}} =\:\left(\boldsymbol{{i}}\:+\:\mathrm{3}\boldsymbol{{j}}\right){m}\:,\boldsymbol{{r}}_{\mathrm{2}} =\left({x}\boldsymbol{{i}}\:+\:\mathrm{5}\boldsymbol{{j}}\right)\:{m} \\ $$$${and}\:\boldsymbol{{r}}_{\mathrm{3}} =\left(−\boldsymbol{{i}}\:+\:\boldsymbol{{j}}\right){m}\:{respectively}\:. \\ $$$${Given}\:{that}\:{this}\:{system}\:{of}\:{forces}\:{is}\:{equivalent}\:{to}\:{a}\:{couple} \\ $$$${of}\:{magnitude}\:\mathrm{12}\:{N}\:{m},\:{find}\: \\ $$$$\left.{a}\right)\:{the}\:{valueof}\:{the}\:{scalars}\:{a}\:{and}\:{b} \\ $$$$\left.{b}\right)\:{the}\:{possible}\:{values}\:{of}\:{the}\:{scalar}\:{x}. \\ $$

Question Number 79735 Answers: 1 Comments: 0

write tanhx in terms of e, hence prove that tanh2x = ((2tanhx)/(1+tanh^2 x))

$${write}\:{tanhx}\:{in}\:{terms}\:{of}\:{e},\:{hence}\:{prove}\:{that}\: \\ $$$${tanh}\mathrm{2}{x}\:=\:\frac{\mathrm{2}{tanhx}}{\mathrm{1}+{tanh}^{\mathrm{2}} {x}} \\ $$

Question Number 79718 Answers: 0 Comments: 0

$$ \\ $$

Question Number 79705 Answers: 0 Comments: 3

if L{f(t)}=L{g(t)} then why f(t)=g(t)? is there any proof

$$\mathrm{if}\:\mathscr{L}\left\{\mathrm{f}\left(\mathrm{t}\right)\right\}=\mathscr{L}\left\{\mathrm{g}\left(\mathrm{t}\right)\right\} \\ $$$$\mathrm{then}\:\mathrm{why}\:\mathrm{f}\left(\mathrm{t}\right)=\mathrm{g}\left(\mathrm{t}\right)? \\ $$$$\mathrm{is}\:\mathrm{there}\:\mathrm{any}\:\mathrm{proof} \\ $$

Question Number 79491 Answers: 0 Comments: 4

Find the number of used place

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{used}\:\mathrm{place} \\ $$

Question Number 79423 Answers: 1 Comments: 0

solve for x and y sinh x − 2cosh y = 0 3cosh x + 6 sihn y = 5

$${solve}\:{for}\:{x}\:{and}\:{y}\: \\ $$$$\:\:\:{sinh}\:{x}\:−\:\mathrm{2}{cosh}\:{y}\:=\:\mathrm{0} \\ $$$$\:\:\:\mathrm{3}{cosh}\:{x}\:+\:\mathrm{6}\:{sihn}\:{y}\:=\:\mathrm{5} \\ $$

Question Number 79394 Answers: 1 Comments: 0

Question Number 79311 Answers: 0 Comments: 2

Question Number 79177 Answers: 1 Comments: 0

Question Number 79111 Answers: 0 Comments: 3

Show that E={(x,y,z) ∈ R^3 / x−2y+z=0} is a subspace vector of which we will determine one base. please help sirs...

$$\mathrm{Show}\:\mathrm{that} \\ $$$$\mathrm{E}=\left\{\left({x},\mathrm{y},{z}\right)\:\in\:\mathbb{R}^{\mathrm{3}} \:\:/\:\:{x}−\mathrm{2}{y}+{z}=\mathrm{0}\right\} \\ $$$$\mathrm{is}\:\mathrm{a}\:\mathrm{subspace}\:\mathrm{vector}\:\mathrm{of}\:\mathrm{which}\:\mathrm{we} \\ $$$$\mathrm{will}\:\mathrm{determine}\:\mathrm{one}\:\mathrm{base}. \\ $$$$\mathrm{please}\:\mathrm{help}\:\mathrm{sirs}... \\ $$

Question Number 79079 Answers: 1 Comments: 0

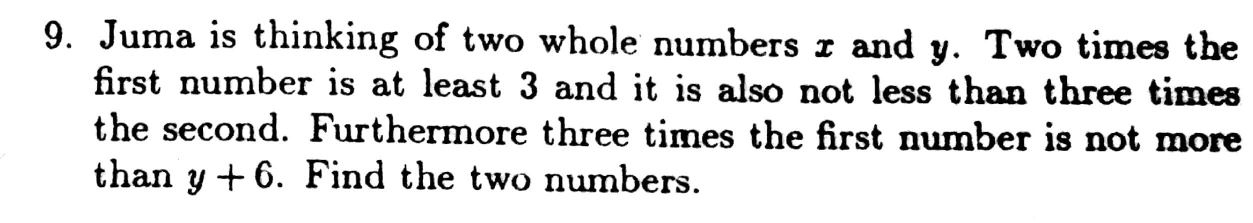
Question Number 79015 Answers: 0 Comments: 4

Rigorously over one month′s time, I developed a formula for general cubic. x^3 +ax^2 +bx+c=0 let x=((pt+q)/(t+1)) pq=m, p+q=s ________________________ m^2 {(a^2 +b)^2 −6a(ab−c)} +m{2(b^2 +ac)(a^2 +b)− 3(ab−c)(ab+3c)} +(b^2 +ac)^2 −6bc(ab−c)=0 ________________________ s=−(2/3){((m(a^2 +b)+b^2 +ac)/(ab−c))} +{(8/(27))[((m(a^2 +b)+b^2 +ac)/(ab−c))]^3 −8[((m^3 +bm^2 +acm+c^2 )/(ab−c))]}^(1/3) p,q = (s/2)±(√((s^2 /4)−m)) t=−(((3pq^2 +2apq+ap^2 +2bp+bq+3c))/((p^3 +ap^2 +bp+c))) x=((pt+q)/(t+1)) . (Please help checking..) (edited a digit 1 in place of 4)

$${Rigorously}\:{over}\:{one}\:{month}'{s} \\ $$$${time},\:{I}\:{developed}\:{a}\:{formula}\:{for} \\ $$$${general}\:{cubic}. \\ $$$${x}^{\mathrm{3}} +{ax}^{\mathrm{2}} +{bx}+{c}=\mathrm{0} \\ $$$${let}\:\:{x}=\frac{{pt}+{q}}{{t}+\mathrm{1}} \\ $$$$\boldsymbol{{pq}}=\boldsymbol{{m}},\:\boldsymbol{{p}}+\boldsymbol{{q}}=\boldsymbol{{s}} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$$\boldsymbol{{m}}^{\mathrm{2}} \left\{\left(\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}\right)^{\mathrm{2}} −\mathrm{6}\boldsymbol{{a}}\left(\boldsymbol{{ab}}−\boldsymbol{{c}}\right)\right\} \\ $$$$+\boldsymbol{{m}}\left\{\mathrm{2}\left(\boldsymbol{{b}}^{\mathrm{2}} +\boldsymbol{{ac}}\right)\left(\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}\right)−\right. \\ $$$$\left.\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{3}\left(\boldsymbol{{ab}}−\boldsymbol{{c}}\right)\left(\boldsymbol{{ab}}+\mathrm{3}\boldsymbol{{c}}\right)\right\} \\ $$$$\:\:\:+\left(\boldsymbol{{b}}^{\mathrm{2}} +\boldsymbol{{ac}}\right)^{\mathrm{2}} −\mathrm{6}\boldsymbol{{bc}}\left(\boldsymbol{{ab}}−\boldsymbol{{c}}\right)=\mathrm{0} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$$\boldsymbol{{s}}=−\frac{\mathrm{2}}{\mathrm{3}}\left\{\frac{\boldsymbol{{m}}\left(\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}\right)+\boldsymbol{{b}}^{\mathrm{2}} +\boldsymbol{{ac}}}{\boldsymbol{{ab}}−\boldsymbol{{c}}}\right\} \\ $$$$\:\:+\left\{\frac{\mathrm{8}}{\mathrm{27}}\left[\frac{\boldsymbol{{m}}\left(\boldsymbol{{a}}^{\mathrm{2}} +\boldsymbol{{b}}\right)+\boldsymbol{{b}}^{\mathrm{2}} +\boldsymbol{{ac}}}{\boldsymbol{{ab}}−\boldsymbol{{c}}}\right]^{\mathrm{3}} \right. \\ $$$$\left.\:\:\:−\mathrm{8}\left[\frac{\boldsymbol{{m}}^{\mathrm{3}} +\boldsymbol{{bm}}^{\mathrm{2}} +\boldsymbol{{acm}}+\boldsymbol{{c}}^{\mathrm{2}} }{\boldsymbol{{ab}}−\boldsymbol{{c}}}\right]\right\}^{\mathrm{1}/\mathrm{3}} \\ $$$$\boldsymbol{{p}},\boldsymbol{{q}}\:=\:\frac{\boldsymbol{{s}}}{\mathrm{2}}\pm\sqrt{\frac{\boldsymbol{{s}}^{\mathrm{2}} }{\mathrm{4}}−\boldsymbol{{m}}} \\ $$$$\boldsymbol{{t}}=−\frac{\left(\mathrm{3}\boldsymbol{{pq}}^{\mathrm{2}} +\mathrm{2}\boldsymbol{{apq}}+\boldsymbol{{ap}}^{\mathrm{2}} +\mathrm{2}\boldsymbol{{bp}}+\boldsymbol{{bq}}+\mathrm{3}\boldsymbol{{c}}\right)}{\left(\boldsymbol{{p}}^{\mathrm{3}} +\boldsymbol{{ap}}^{\mathrm{2}} +\boldsymbol{{bp}}+\boldsymbol{{c}}\right)} \\ $$$$\boldsymbol{{x}}=\frac{\boldsymbol{{pt}}+\boldsymbol{{q}}}{\boldsymbol{{t}}+\mathrm{1}}\:. \\ $$$$\left({Please}\:{help}\:{checking}..\right) \\ $$$$\left({edited}\:{a}\:{digit}\:\mathrm{1}\:{in}\:{place}\:{of}\:\mathrm{4}\right) \\ $$

Question Number 78880 Answers: 1 Comments: 0

x + (1/x) = 3 , x ∈ R (x^(2020) + (1/x^(2020) )) mod (10) = y y^2 − 1 = ?

$${x}\:+\:\frac{\mathrm{1}}{{x}}\:\:=\:\:\mathrm{3}\:\:\:,\:\:\:\:{x}\:\in\:\mathbb{R} \\ $$$$\left({x}^{\mathrm{2020}} \:+\:\frac{\mathrm{1}}{{x}^{\mathrm{2020}} }\right)\:\:{mod}\:\left(\mathrm{10}\right)\:\:=\:\:{y} \\ $$$${y}^{\mathrm{2}} \:−\:\mathrm{1}\:\:=\:\:? \\ $$

Question Number 78851 Answers: 0 Comments: 1

Question Number 78683 Answers: 0 Comments: 4

solve x^4 −18x−35=0 by using substitution x= u+v

$${solve}\:{x}^{\mathrm{4}} −\mathrm{18}{x}−\mathrm{35}=\mathrm{0}\:{by}\:{using}\:{substitution}\:{x}=\:{u}+{v} \\ $$

Pg 72 Pg 73 Pg 74 Pg 75 Pg 76 Pg 77 Pg 78 Pg 79 Pg 80 Pg 81

Contact: info@tinkutara.com