
OthersQuestion and Answers: Page 69
Question Number 91946 Answers: 2 Comments: 3
Question Number 91931 Answers: 0 Comments: 3
$$\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left(\Gamma\left({x}\right)\right)\:{dx} \\ $$
Question Number 91930 Answers: 0 Comments: 2
$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}cos}\:\frac{\mathrm{1}}{{x}}= \\ $$
Question Number 91785 Answers: 0 Comments: 5
Question Number 91783 Answers: 0 Comments: 1
Question Number 91715 Answers: 0 Comments: 4
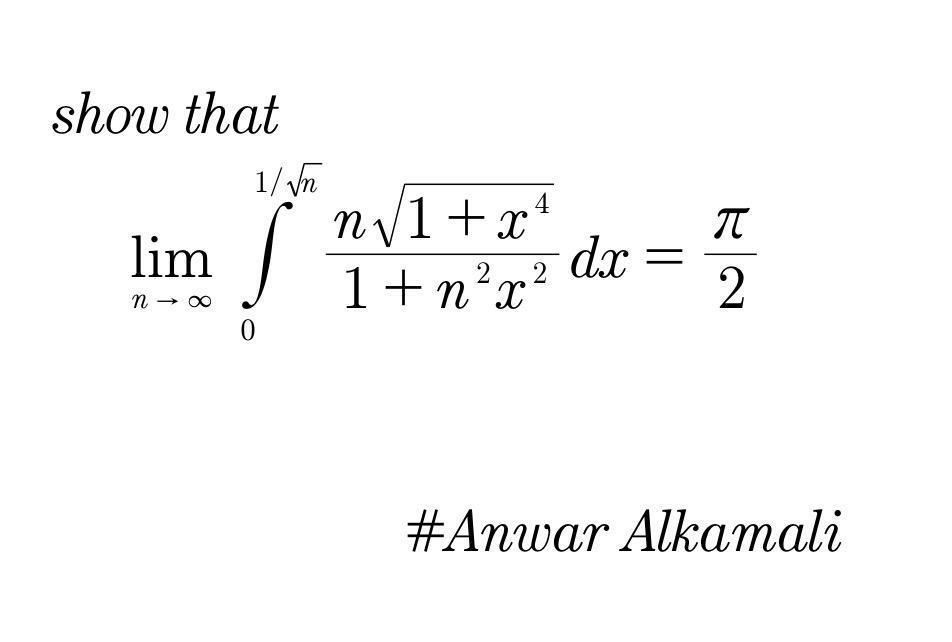
Question Number 91655 Answers: 2 Comments: 12
Question Number 91654 Answers: 1 Comments: 0
Question Number 91638 Answers: 1 Comments: 0
Question Number 91613 Answers: 1 Comments: 4
Question Number 91604 Answers: 0 Comments: 0

Question Number 91588 Answers: 0 Comments: 2
$${what}\:{is}\:{f}^{−\mathrm{1}} \:{for}\:{f}\left({x}\right)=\lfloor{x}\rfloor?? \\ $$
Question Number 91476 Answers: 0 Comments: 2
Question Number 91421 Answers: 2 Comments: 2
Question Number 91375 Answers: 0 Comments: 3
Question Number 91374 Answers: 0 Comments: 0
Question Number 91303 Answers: 0 Comments: 3
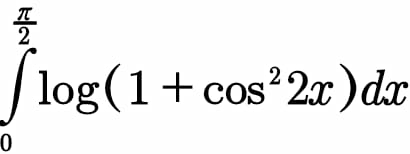
Question Number 91302 Answers: 2 Comments: 0

Question Number 91297 Answers: 0 Comments: 2
Question Number 91149 Answers: 1 Comments: 1
Question Number 91119 Answers: 0 Comments: 0
Question Number 91099 Answers: 1 Comments: 12
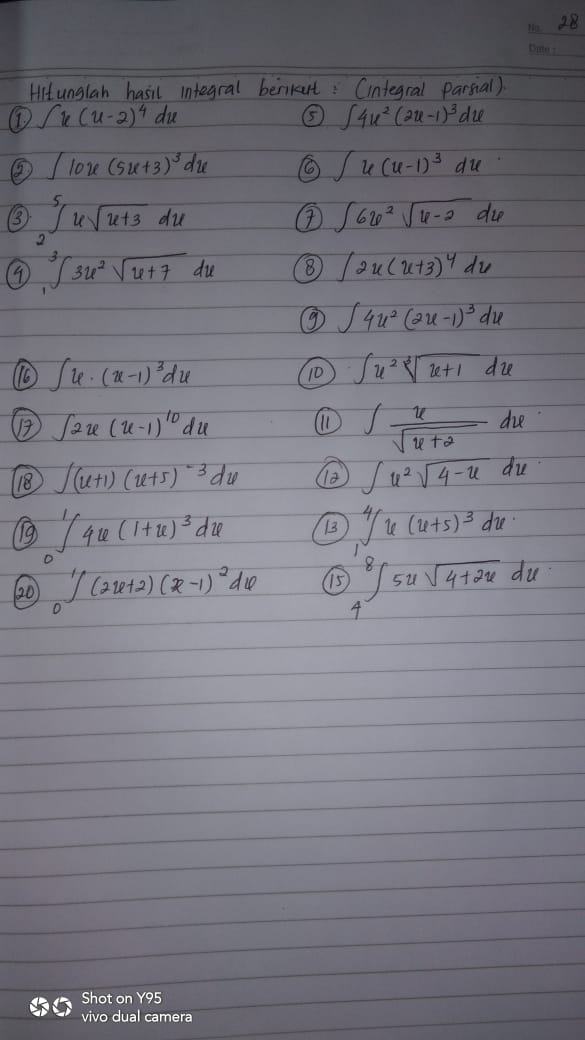
Question Number 90947 Answers: 0 Comments: 0
Question Number 90940 Answers: 1 Comments: 0
Question Number 90709 Answers: 0 Comments: 2
Question Number 90692 Answers: 1 Comments: 2

