
OthersQuestion and Answers: Page 61
Question Number 106373 Answers: 1 Comments: 0
Question Number 106366 Answers: 1 Comments: 0

Question Number 106363 Answers: 0 Comments: 0
Question Number 106360 Answers: 0 Comments: 0
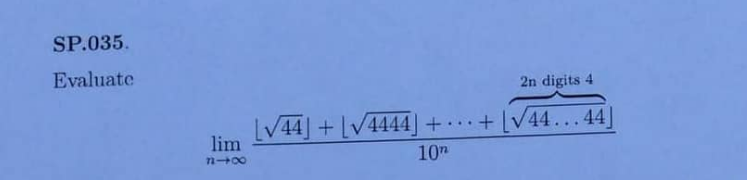
Question Number 106337 Answers: 3 Comments: 4
Question Number 106333 Answers: 0 Comments: 1

Question Number 106329 Answers: 2 Comments: 0
Question Number 106318 Answers: 0 Comments: 0
Question Number 106163 Answers: 0 Comments: 0

Question Number 106039 Answers: 3 Comments: 0
Question Number 105498 Answers: 1 Comments: 3
Question Number 105488 Answers: 1 Comments: 0
Question Number 105207 Answers: 1 Comments: 3
Question Number 105278 Answers: 1 Comments: 1
Question Number 105130 Answers: 0 Comments: 0
Question Number 105080 Answers: 2 Comments: 0
Question Number 105075 Answers: 1 Comments: 0
Question Number 104975 Answers: 0 Comments: 2
Question Number 104948 Answers: 0 Comments: 0

Question Number 104940 Answers: 1 Comments: 1

Question Number 104789 Answers: 0 Comments: 1
Question Number 104783 Answers: 2 Comments: 0
Question Number 104777 Answers: 3 Comments: 0
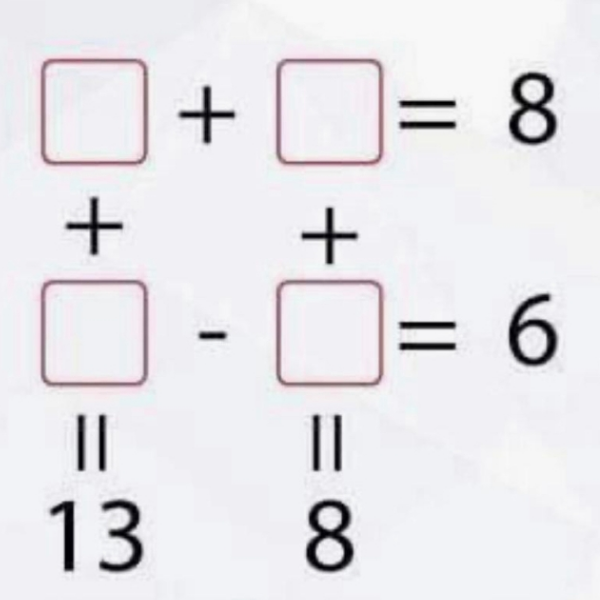
Question Number 104768 Answers: 1 Comments: 0
Question Number 104692 Answers: 2 Comments: 0

Question Number 104533 Answers: 3 Comments: 0
