
OthersQuestion and Answers: Page 60
Question Number 107704 Answers: 0 Comments: 0

Question Number 107628 Answers: 0 Comments: 0

Question Number 107533 Answers: 2 Comments: 0
Question Number 107512 Answers: 0 Comments: 3
Question Number 107420 Answers: 1 Comments: 1

Question Number 107403 Answers: 0 Comments: 0

Question Number 107367 Answers: 0 Comments: 0

Question Number 107362 Answers: 0 Comments: 1
$$\int_{\mathrm{0}} ^{\infty} \mathrm{x}^{\pi} \mathrm{e}^{−\mathrm{x}} \mathrm{dx} \\ $$
Question Number 107353 Answers: 2 Comments: 0
Question Number 107264 Answers: 2 Comments: 1
Question Number 107263 Answers: 0 Comments: 1
$$\underset{\mathrm{n}=\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\sqrt{\mathrm{n}} \\ $$
Question Number 107187 Answers: 1 Comments: 3
Question Number 107178 Answers: 1 Comments: 1
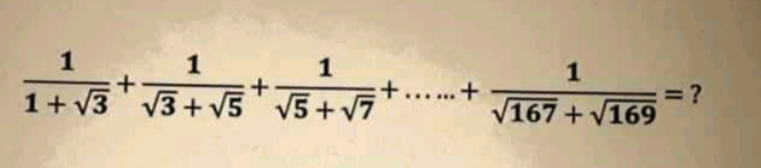
Question Number 107101 Answers: 0 Comments: 0
Question Number 107092 Answers: 1 Comments: 2

Question Number 106828 Answers: 0 Comments: 2
$$\:\int\:\frac{\mathrm{1}}{{xdx}}\:{is}\:{that}\:{true}! \\ $$
Question Number 106825 Answers: 5 Comments: 0
Question Number 106694 Answers: 3 Comments: 0

Question Number 106653 Answers: 0 Comments: 7
$$\mathrm{30}+\mathrm{144}+\mathrm{420}+\mathrm{960}+\mathrm{1890}+\mathrm{3360}+...{n} \\ $$
Question Number 106637 Answers: 1 Comments: 0
Question Number 106630 Answers: 2 Comments: 0
Question Number 106526 Answers: 0 Comments: 2

Question Number 106503 Answers: 0 Comments: 11
Question Number 106454 Answers: 0 Comments: 0

Question Number 106432 Answers: 0 Comments: 5

Question Number 106382 Answers: 0 Comments: 0

