Question and Answers Forum

All Questions Topic List

OthersQuestion and Answers: Page 58

Question Number 111466 Answers: 1 Comments: 2

Σ_(n=1) ^∞ (n^n /(n!))

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{{n}} }{{n}!} \\ $$

Question Number 111450 Answers: 0 Comments: 1

Question Number 111428 Answers: 0 Comments: 1

Σ_(n=1) ^∞ (n^3 /(n!))

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}^{\mathrm{3}} }{{n}!} \\ $$

Question Number 111132 Answers: 1 Comments: 0

prove by mathematical induction ⇒ 7^n −(3n+4)×4^(n−1) divided by 9

$$\mathrm{prove}\:\mathrm{by}\:\mathrm{mathematical}\:\mathrm{induction} \\ $$$$\Rightarrow\:\mathrm{7}^{\mathrm{n}} −\left(\mathrm{3n}+\mathrm{4}\right)×\mathrm{4}^{\mathrm{n}−\mathrm{1}} \:\mathrm{divided}\:\mathrm{by}\:\mathrm{9} \\ $$

Question Number 111152 Answers: 0 Comments: 0

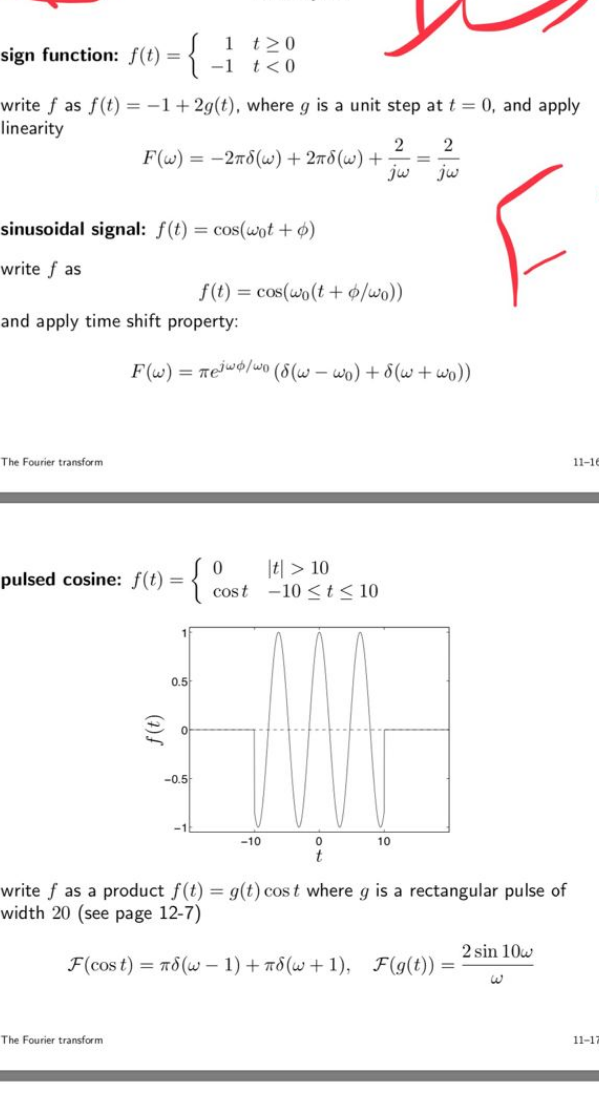
Question Number 111080 Answers: 1 Comments: 0

(√(bemath)) (1)Σ_(k=50) ^(100) (1/(k(151−k))) ? (2) without L′Hopital and series find the value of lim_(x→0) ((xcos x−sin x)/(x^2 sin x))

$$\:\:\sqrt{\mathrm{bemath}} \\ $$$$\left(\mathrm{1}\right)\underset{\mathrm{k}=\mathrm{50}} {\overset{\mathrm{100}} {\sum}}\:\frac{\mathrm{1}}{\mathrm{k}\left(\mathrm{151}−\mathrm{k}\right)}\:? \\ $$$$\left(\mathrm{2}\right)\:\mathrm{without}\:\mathrm{L}'\mathrm{Hopital}\:\mathrm{and}\:\mathrm{series}\: \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{xcos}\:\mathrm{x}−\mathrm{sin}\:\mathrm{x}}{\mathrm{x}^{\mathrm{2}} \mathrm{sin}\:\mathrm{x}} \\ $$

Question Number 110860 Answers: 1 Comments: 0

Question Number 110742 Answers: 0 Comments: 0

please give me a result of E(tanx)

$${please}\:{give}\:{me}\:{a}\:{result}\:{of}\:{E}\left({tanx}\right) \\ $$

Question Number 110385 Answers: 0 Comments: 3

Question Number 110920 Answers: 1 Comments: 1

Question Number 110919 Answers: 1 Comments: 0

lim_(n→∞) (Σ_(r=1) ^n (1/(3^r r!))(Π_(k=1) ^r (2k−1)))

$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{\mathrm{3}^{{r}} {r}!}\left(\underset{{k}=\mathrm{1}} {\overset{{r}} {\prod}}\left(\mathrm{2}{k}−\mathrm{1}\right)\right)\right) \\ $$

Question Number 109922 Answers: 1 Comments: 0

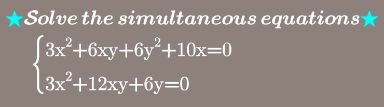
Question Number 109787 Answers: 0 Comments: 0

How to prove that ▽×(▽×E)= ▽▽.E−▽^2 E, where E is the eletric field?

$${How}\:{to}\:{prove}\:{that}\:\bigtriangledown×\left(\bigtriangledown×{E}\right)=\:\bigtriangledown\bigtriangledown.{E}−\bigtriangledown^{\mathrm{2}} {E},\:{where}\:{E}\:{is}\:{the}\:{eletric}\:{field}? \\ $$

Question Number 109776 Answers: 0 Comments: 0

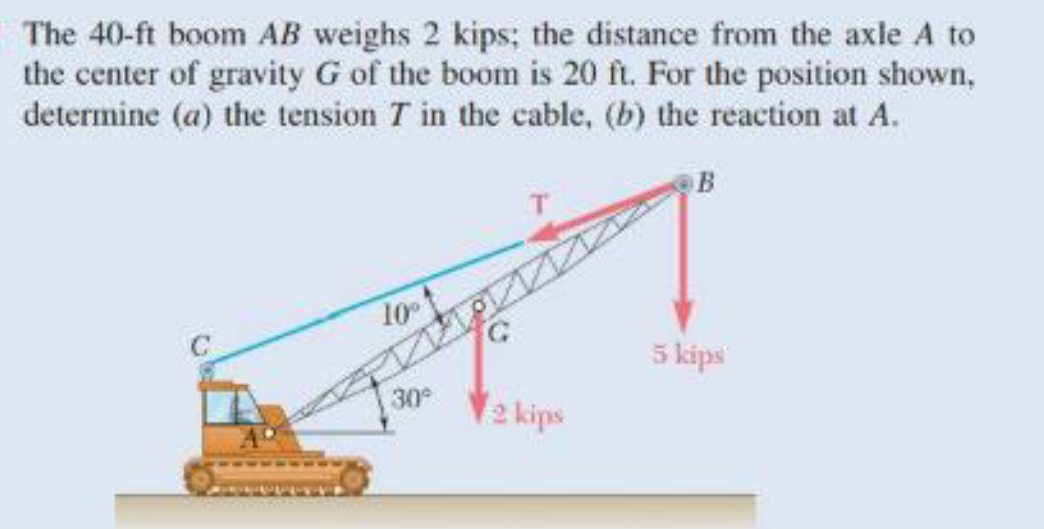
Question Number 109655 Answers: 0 Comments: 0

Question Number 109619 Answers: 0 Comments: 2

Σ_(n=1) ^∞ ((n!)/n^n )

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}!}{{n}^{{n}} } \\ $$

Question Number 109208 Answers: 1 Comments: 0

∫_0 ^∞ ((x^n −1)/(x−1)).(x/e^x )dx

$$\int_{\mathrm{0}} ^{\infty} \frac{{x}^{{n}} −\mathrm{1}}{{x}−\mathrm{1}}.\frac{{x}}{{e}^{{x}} }{dx} \\ $$

Question Number 109123 Answers: 0 Comments: 0

prove that : ∫_(π/4) ^((3π)/4) sin(x)−cos(x)dx ≥∫_π ^((3π)/2) sin(x)+cos(x)dx

$${prove}\:{that}\:: \\ $$$$\int_{\frac{\pi}{\mathrm{4}}} ^{\frac{\mathrm{3}\pi}{\mathrm{4}}} {sin}\left({x}\right)−{cos}\left({x}\right){dx}\:\geqslant\int_{\pi} ^{\frac{\mathrm{3}\pi}{\mathrm{2}}} {sin}\left({x}\right)+{cos}\left({x}\right){dx} \\ $$

Question Number 109119 Answers: 2 Comments: 0

if f(x^2 )=y ,f′(x)=(√(5x−1 )) then (dy/dx)=.....

$${if}\:{f}\left({x}^{\mathrm{2}} \right)={y}\:\:,{f}'\left({x}\right)=\sqrt{\mathrm{5}{x}−\mathrm{1}\:}\:{then}\: \\ $$$$\frac{{dy}}{{dx}}=..... \\ $$

Question Number 109117 Answers: 1 Comments: 0

Question Number 109082 Answers: 1 Comments: 0

prove that : ∫_(−(π/2)) ^(−(π/4)) 2cos(x)+sin(x)dx≤∫_(−(π/2)) ^(−(π/4)) cos(x)−sin(x)dx

$${prove}\:{that}\:: \\ $$$$\int_{−\frac{\pi}{\mathrm{2}}} ^{−\frac{\pi}{\mathrm{4}}} \mathrm{2}{cos}\left({x}\right)+{sin}\left({x}\right){dx}\leqslant\int_{−\frac{\pi}{\mathrm{2}}} ^{−\frac{\pi}{\mathrm{4}}} {cos}\left({x}\right)−{sin}\left({x}\right){dx} \\ $$

Question Number 108951 Answers: 0 Comments: 0

Σ_(n=1) ^∞ ((n!)/n^n )

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}!}{{n}^{{n}} } \\ $$

Question Number 108907 Answers: 1 Comments: 1

Three Catagories of Posts: Question , Answer & Comment Use Question for only questions. (Don′t use it for solutions & comments.)

$$\mathcal{T}{hree}\:\:\mathcal{C}{atagories}\:{of}\:\mathcal{P}{osts}: \\ $$$${Question}\:,\:\mathcal{A}{nswer}\:\&\:\mathcal{C}{omment} \\ $$$$ \\ $$$$\mathbb{U}\mathrm{se}\:{Question}\:\mathrm{for}\:\boldsymbol{\mathrm{only}}\:\mathrm{questions}. \\ $$$$\left(\mathcal{D}{on}'{t}\:{use}\:{it}\:{for}\:{solutions}\:\&\right. \\ $$$$\left.{comments}.\right) \\ $$

Question Number 108571 Answers: 2 Comments: 0

Question Number 108290 Answers: 0 Comments: 3

Why do all active content of forum of dates 12,13,14was disappear on my phone?

$$\mathrm{Why}\:\mathrm{do}\:\mathrm{all}\:\mathrm{active}\:\mathrm{content}\:\mathrm{of}\:\mathrm{forum}\:\mathrm{of} \\ $$$$\mathrm{dates}\:\mathrm{12},\mathrm{13},\mathrm{14was}\:\mathrm{disappear}\:\mathrm{on}\:\mathrm{my} \\ $$$$\mathrm{phone}? \\ $$

Question Number 108259 Answers: 0 Comments: 0

Σ_(n=1) ^∞ ((n!)/n^n )

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}!}{{n}^{{n}} } \\ $$

Pg 53 Pg 54 Pg 55 Pg 56 Pg 57 Pg 58 Pg 59 Pg 60 Pg 61 Pg 62

Contact: info@tinkutara.com