Question and Answers Forum

OthersQuestion and Answers: Page 53

Question Number 124642 Answers: 0 Comments: 0

Evaluate : ∫ (4/( (√(2−((1/( ((x^2 + 1))^(1/3) )))^4 ))))dx

$$\: \\ $$$$\:\:\:{Evaluate}\::\: \\ $$$$\:\:\:\int\:\frac{\mathrm{4}}{\:\sqrt{\mathrm{2}−\left(\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{{x}^{\mathrm{2}} \:+\:\mathrm{1}}}\right)^{\mathrm{4}} }}{dx} \\ $$

Question Number 124595 Answers: 4 Comments: 0

Given that ω = e^(iθ) , θ≠ nπ, n ∈ N show that (1) ((ω^2 −1)/ω) = 2i sin θ (2) (1 + ω)^n = 2^n cos^n ((1/2)θ)e^((1/2)(inθ))

$$\mathrm{Given}\:\mathrm{that}\:\:\omega\:=\:{e}^{{i}\theta} ,\:\theta\neq\:{n}\pi,\:{n}\:\in\:\mathbb{N} \\ $$$$\mathrm{show}\:\mathrm{that}\: \\ $$$$\:\left(\mathrm{1}\right)\:\frac{\omega^{\mathrm{2}} −\mathrm{1}}{\omega}\:=\:\mathrm{2}{i}\:\mathrm{sin}\:\theta \\ $$$$\:\left(\mathrm{2}\right)\:\left(\mathrm{1}\:+\:\omega\right)^{{n}} \:=\:\mathrm{2}^{{n}} \mathrm{cos}^{{n}} \left(\frac{\mathrm{1}}{\mathrm{2}}\theta\right){e}^{\frac{\mathrm{1}}{\mathrm{2}}\left({in}\theta\right)} \\ $$

Question Number 124528 Answers: 0 Comments: 0

Question Number 124487 Answers: 1 Comments: 1

Question Number 124474 Answers: 1 Comments: 1

((4/(−6+i(√5))))^4 =??? polar????

$$\left(\frac{\mathrm{4}}{−\mathrm{6}+{i}\sqrt{\mathrm{5}}}\right)^{\mathrm{4}} =??? \\ $$$$ \\ $$$${polar}???? \\ $$

Question Number 124461 Answers: 2 Comments: 2

∫_0 ^∞ e^(−x^7 ) sin(x^7 )dx

$$\int_{\mathrm{0}} ^{\infty} {e}^{−{x}^{\mathrm{7}} } {sin}\left({x}^{\mathrm{7}} \right){dx} \\ $$

Question Number 124459 Answers: 1 Comments: 1

1−(1/2)((1/2))+(1/4)((1/2).(3/4))−(1/8)(((1.3.5)/(2.4.6)))+...

$$\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)+\frac{\mathrm{1}}{\mathrm{4}}\left(\frac{\mathrm{1}}{\mathrm{2}}.\frac{\mathrm{3}}{\mathrm{4}}\right)−\frac{\mathrm{1}}{\mathrm{8}}\left(\frac{\mathrm{1}.\mathrm{3}.\mathrm{5}}{\mathrm{2}.\mathrm{4}.\mathrm{6}}\right)+... \\ $$

Question Number 124347 Answers: 1 Comments: 1

Question Number 124321 Answers: 2 Comments: 0

f(x,y,z)=z^2 yz^3 A=xzi−y^2 j+2x^2 yk div(fA)=? curl(fA)=?

$${f}\left({x},{y},{z}\right)={z}^{\mathrm{2}} {yz}^{\mathrm{3}} \\ $$$${A}={xzi}−{y}^{\mathrm{2}} {j}+\mathrm{2}{x}^{\mathrm{2}} {yk} \\ $$$${div}\left({fA}\right)=? \\ $$$${curl}\left({fA}\right)=? \\ $$

Question Number 124320 Answers: 2 Comments: 0

z+(1/z)=1 z^3 =??

$${z}+\frac{\mathrm{1}}{{z}}=\mathrm{1} \\ $$$${z}^{\mathrm{3}} =?? \\ $$

Question Number 124300 Answers: 0 Comments: 0

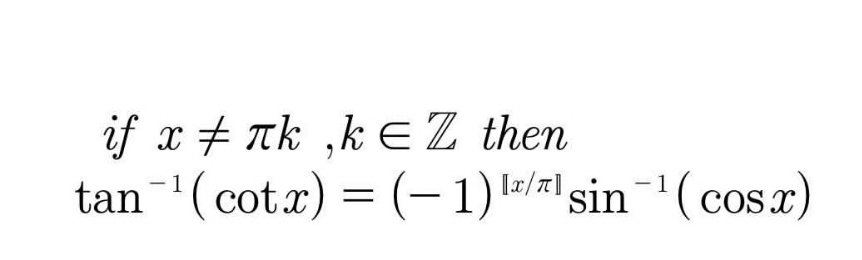
Question Number 124302 Answers: 1 Comments: 0

n=2^(n−1) sin((π/n))sin(((2π)/n))....sin(((n−1)/n)π) prove

$${n}=\mathrm{2}^{{n}−\mathrm{1}} {sin}\left(\frac{\pi}{{n}}\right){sin}\left(\frac{\mathrm{2}\pi}{{n}}\right)....{sin}\left(\frac{{n}−\mathrm{1}}{{n}}\pi\right)\:\:{prove}\: \\ $$$$ \\ $$

Question Number 124271 Answers: 0 Comments: 0

Question Number 124178 Answers: 1 Comments: 6

∫_0 ^(π/2) (1/( (√(cos^4 x+sin^4 x))))dx

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{1}}{\:\sqrt{{cos}^{\mathrm{4}} {x}+{sin}^{\mathrm{4}} {x}}}{dx} \\ $$

Question Number 123947 Answers: 0 Comments: 0

((9^2 −1^2 )/(9^2 −2^2 )).((18^2 −1^2 )/(18^2 −0^2 )).((27^2 −1^2 )/(27^2 −2^2 )).((36^2 −1^2 )/(36^2 −0^2 )).....

$$\frac{\mathrm{9}^{\mathrm{2}} −\mathrm{1}^{\mathrm{2}} }{\mathrm{9}^{\mathrm{2}} −\mathrm{2}^{\mathrm{2}} }.\frac{\mathrm{18}^{\mathrm{2}} −\mathrm{1}^{\mathrm{2}} }{\mathrm{18}^{\mathrm{2}} −\mathrm{0}^{\mathrm{2}} }.\frac{\mathrm{27}^{\mathrm{2}} −\mathrm{1}^{\mathrm{2}} }{\mathrm{27}^{\mathrm{2}} −\mathrm{2}^{\mathrm{2}} }.\frac{\mathrm{36}^{\mathrm{2}} −\mathrm{1}^{\mathrm{2}} }{\mathrm{36}^{\mathrm{2}} −\mathrm{0}^{\mathrm{2}} }..... \\ $$

Question Number 123845 Answers: 1 Comments: 1

Question Number 123823 Answers: 1 Comments: 0

Question Number 123807 Answers: 0 Comments: 1

((((1/2))!(1)!)/(((5/2))!))−((((3/2))!(2)!)/(((9/2))!))+((((5/2))!3!)/((((13)/2))!))−((((7/2))!4!)/((((17)/2))!))+....

$$\frac{\left(\frac{\mathrm{1}}{\mathrm{2}}\right)!\left(\mathrm{1}\right)!}{\left(\frac{\mathrm{5}}{\mathrm{2}}\right)!}−\frac{\left(\frac{\mathrm{3}}{\mathrm{2}}\right)!\left(\mathrm{2}\right)!}{\left(\frac{\mathrm{9}}{\mathrm{2}}\right)!}+\frac{\left(\frac{\mathrm{5}}{\mathrm{2}}\right)!\mathrm{3}!}{\left(\frac{\mathrm{13}}{\mathrm{2}}\right)!}−\frac{\left(\frac{\mathrm{7}}{\mathrm{2}}\right)!\mathrm{4}!}{\left(\frac{\mathrm{17}}{\mathrm{2}}\right)!}+.... \\ $$

Question Number 123776 Answers: 0 Comments: 0

Another unanswered question ((log1)/( (√1)))−((log3)/( (√3)))+((log5)/( (√5)))−((log7)/( (√7)))+..=((π/4)−(γ/2)−(1/2)log(2π))((1/( (√1)))−(1/( (√3)))+(1/( (√5)))−.)

$${Another}\:{unanswered}\:{question} \\ $$$$\frac{{log}\mathrm{1}}{\:\sqrt{\mathrm{1}}}−\frac{{log}\mathrm{3}}{\:\sqrt{\mathrm{3}}}+\frac{{log}\mathrm{5}}{\:\sqrt{\mathrm{5}}}−\frac{{log}\mathrm{7}}{\:\sqrt{\mathrm{7}}}+..=\left(\frac{\pi}{\mathrm{4}}−\frac{\gamma}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}{log}\left(\mathrm{2}\pi\right)\right)\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{3}}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}−.\right) \\ $$

Question Number 123794 Answers: 0 Comments: 0

...mathematical analysis... suppose (X_1 ,τ_1 ) and (X_2 ,τ_2 ) are two topological spaces. prove f:(X_1 ,τ_1 )→(X_2 ,τ_2 ) is a continuous function if only if for any subset A⊆X_1 : f(cl(A))⊆cl(f(A))

$$\:\:\:\:\:\:\:\:\:\:\:...{mathematical}\:\:{analysis}... \\ $$$$\:{suppose}\:\:\left({X}_{\mathrm{1}} ,\tau_{\mathrm{1}} \right)\:{and}\:\left({X}_{\mathrm{2}} ,\tau_{\mathrm{2}} \right) \\ $$$$\:{are}\:{two}\:{topological}\:{spaces}. \\ $$$${prove}\:\:{f}:\left({X}_{\mathrm{1}} ,\tau_{\mathrm{1}} \right)\rightarrow\left({X}_{\mathrm{2}} ,\tau_{\mathrm{2}} \right)\:{is}\: \\ $$$${a}\:{continuous}\:{function}\:{if}\:{only} \\ $$$${if}\:\:{for}\:{any}\:{subset}\:{A}\subseteq{X}_{\mathrm{1}} \:: \\ $$$$\:\:\:\:\:\:\:{f}\left({cl}\left({A}\right)\right)\subseteq{cl}\left({f}\left({A}\right)\right) \\ $$$$\: \\ $$

Question Number 123694 Answers: 0 Comments: 1

sinh((1/1^2 ))+sinh((1/2^2 ))+sinh((1/3^2 ))+....

$${sinh}\left(\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{2}} }\right)+{sinh}\left(\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }\right)+{sinh}\left(\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }\right)+.... \\ $$

Question Number 123653 Answers: 1 Comments: 0

Question Number 123651 Answers: 1 Comments: 0

Question Number 123650 Answers: 0 Comments: 4

1−(1/4)+(1/8)−(1/(10))+(1/(14))−(1/(16))+...

$$\mathrm{1}−\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{8}}−\frac{\mathrm{1}}{\mathrm{10}}+\frac{\mathrm{1}}{\mathrm{14}}−\frac{\mathrm{1}}{\mathrm{16}}+... \\ $$

Question Number 123620 Answers: 0 Comments: 0

((coth(π))/1^7 )+((coth(2π))/2^7 )+((coth(3π))/3^7 )+...

$$\frac{{coth}\left(\pi\right)}{\mathrm{1}^{\mathrm{7}} }+\frac{{coth}\left(\mathrm{2}\pi\right)}{\mathrm{2}^{\mathrm{7}} }+\frac{{coth}\left(\mathrm{3}\pi\right)}{\mathrm{3}^{\mathrm{7}} }+... \\ $$

Question Number 123460 Answers: 0 Comments: 1

sinh^(−1) (1)+sinh^(−1) ((1/2^2 ))+sinh^(−1) ((1/3^2 ))+sinh^(−1) ((1/4^2 ))+....

$${sinh}^{−\mathrm{1}} \left(\mathrm{1}\right)+{sinh}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }\right)+{sinh}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }\right)+{sinh}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{4}^{\mathrm{2}} }\right)+.... \\ $$$$ \\ $$

Pg 48 Pg 49 Pg 50 Pg 51 Pg 52 Pg 53 Pg 54 Pg 55 Pg 56 Pg 57

Contact: info@tinkutara.com