Question and Answers Forum

All Questions Topic List

OthersQuestion and Answers: Page 52

Question Number 126704 Answers: 0 Comments: 0

((e^π −1)/(e^π +1))=(π/(2+(π^2 /(6+(π^2 /(10+(π^2 /(14+....))))))))

$$\frac{{e}^{\pi} −\mathrm{1}}{{e}^{\pi} +\mathrm{1}}=\frac{\pi}{\mathrm{2}+\frac{\pi^{\mathrm{2}} }{\mathrm{6}+\frac{\pi^{\mathrm{2}} }{\mathrm{10}+\frac{\pi^{\mathrm{2}} }{\mathrm{14}+....}}}} \\ $$

Question Number 192126 Answers: 2 Comments: 1

prove that ∣z∣ > ((∣Re(z)∣ +∣Im(z)∣)/2) , ∀z∈C

$$\:\:\:\:\boldsymbol{{prove}}\:\boldsymbol{{that}} \\ $$$$\:\:\:\:\:\:\mid\boldsymbol{{z}}\mid\:>\:\frac{\mid\boldsymbol{{Re}}\left(\boldsymbol{{z}}\right)\mid\:+\mid\boldsymbol{{Im}}\left(\boldsymbol{{z}}\right)\mid}{\mathrm{2}}\:\:,\:\:\:\forall\boldsymbol{{z}}\in\mathbb{C} \\ $$

Question Number 126669 Answers: 1 Comments: 2

Σ_(n=1) ^∞ (H_n ^2 /n^4 )

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{H}_{{n}} ^{\mathrm{2}} }{{n}^{\mathrm{4}} } \\ $$

Question Number 126632 Answers: 2 Comments: 1

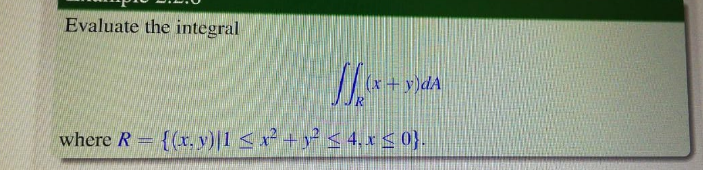
Question Number 126400 Answers: 1 Comments: 5

If a_n =6^n +8^n find (a_(1991) /(49)).

$${If}\:{a}_{{n}} =\mathrm{6}^{{n}} +\mathrm{8}^{{n}} \:{find}\:\frac{{a}_{\mathrm{1991}} }{\mathrm{49}}. \\ $$

Question Number 126200 Answers: 0 Comments: 3

(1/1^2 )−(1/2^3 )+(1/3^5 )−(1/4^7 )+(1/5^(11) )−(1/6^(13) )+....

$$\frac{\mathrm{1}}{\mathrm{1}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{3}} }+\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{5}} }−\frac{\mathrm{1}}{\mathrm{4}^{\mathrm{7}} }+\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{11}} }−\frac{\mathrm{1}}{\mathrm{6}^{\mathrm{13}} }+.... \\ $$

Question Number 126092 Answers: 2 Comments: 0

A particle starts from rest at time t = 0 and moves in a straightline with variable acceleration a m/s^2 where a = (t/5) , 0 ≤ t ≤ 5 , a = (t/5) + ((10)/t^2 ) , t ≥ 5, t being measured in seconds. Show that the velocity is 22(1/2) m/s when t = 5 and 11 m/s when t = 10. Show also that the distance travelled by the particle in the first 10 seconds is (43(1/3)−10 ln 2) m.

$$\mathrm{A}\:\mathrm{particle}\:\mathrm{starts}\:\mathrm{from}\:\mathrm{rest}\:\mathrm{at}\:\mathrm{time}\:{t}\:=\:\mathrm{0}\:\mathrm{and}\:\mathrm{moves}\:\mathrm{in}\: \\ $$$$\mathrm{a}\:\mathrm{straightline}\:\mathrm{with}\:\mathrm{variable}\:\mathrm{acceleration}\:{a}\:\mathrm{m}/\mathrm{s}^{\mathrm{2}} \:\mathrm{where}\: \\ $$$$\:{a}\:=\:\frac{{t}}{\mathrm{5}}\:,\:\mathrm{0}\:\leqslant\:{t}\:\leqslant\:\mathrm{5}\:,\:{a}\:=\:\frac{{t}}{\mathrm{5}}\:+\:\frac{\mathrm{10}}{{t}^{\mathrm{2}} }\:,\:{t}\:\geqslant\:\mathrm{5},\:{t}\:\mathrm{being}\:\mathrm{measured}\:\mathrm{in}\:\mathrm{seconds}. \\ $$$$\mathrm{Show}\:\mathrm{that}\:\mathrm{the}\:\mathrm{velocity}\:\mathrm{is}\:\mathrm{22}\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{m}/\mathrm{s}\:\mathrm{when}\:{t}\:=\:\mathrm{5}\:\mathrm{and} \\ $$$$\mathrm{11}\:\mathrm{m}/\mathrm{s}\:\mathrm{when}\:{t}\:=\:\mathrm{10}. \\ $$$$\mathrm{Show}\:\mathrm{also}\:\mathrm{that}\:\mathrm{the}\:\mathrm{distance}\:\mathrm{travelled}\:\mathrm{by}\:\mathrm{the}\:\mathrm{particle} \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{first}\:\mathrm{10}\:\mathrm{seconds}\:\mathrm{is}\:\:\left(\mathrm{43}\frac{\mathrm{1}}{\mathrm{3}}−\mathrm{10}\:\mathrm{ln}\:\mathrm{2}\right)\:\mathrm{m}. \\ $$

Question Number 125994 Answers: 1 Comments: 0

((Σ_(n=0) ^∞ e^(−n^2 ) )/(Σ_(n=0) ^∞ e^(−2n^2 ) ))

$$\frac{\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}{e}^{−{n}^{\mathrm{2}} } }{\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}{e}^{−\mathrm{2}{n}^{\mathrm{2}} } } \\ $$

Question Number 127492 Answers: 0 Comments: 0

((i!)/(π!)) (Exact form)

$$\frac{{i}!}{\pi!}\:\left({Exact}\:{form}\right) \\ $$

Question Number 125939 Answers: 0 Comments: 0

∫_0 ^1 ((cos2x−tanx.cot(tanx))/(sin2x−tan(tanx)log(cos^2 x)))dx

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{cos}\mathrm{2}{x}−{tanx}.{cot}\left({tanx}\right)}{{sin}\mathrm{2}{x}−{tan}\left({tanx}\right){log}\left({cos}^{\mathrm{2}} {x}\right)}{dx} \\ $$$$ \\ $$

Question Number 125884 Answers: 0 Comments: 0

Σ_(n=0) ^∞ ((((√5)−2)^n (((2n)),(n) ))/(((2n+1)(((√5)+(1/( (√5))))^(n+(1/2)) +((√5)−(1/( (√5))))^(n−(1/2)) ))))

$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\sqrt{\mathrm{5}}−\mathrm{2}\right)^{{n}} \begin{pmatrix}{\mathrm{2}{n}}\\{{n}}\end{pmatrix}}{\left(\left(\mathrm{2}{n}+\mathrm{1}\right)\left(\left(\sqrt{\mathrm{5}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}\right)^{{n}+\frac{\mathrm{1}}{\mathrm{2}}} +\left(\sqrt{\mathrm{5}}−\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}\right)^{{n}−\frac{\mathrm{1}}{\mathrm{2}}} \right)\right)} \\ $$

Question Number 125857 Answers: 1 Comments: 0

1+4((1/2))^7 +7(((1.3)/(2.4)))^7 +10(((1.3.5)/(2.4.6)))^7 +...

$$\mathrm{1}+\mathrm{4}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{7}} +\mathrm{7}\left(\frac{\mathrm{1}.\mathrm{3}}{\mathrm{2}.\mathrm{4}}\right)^{\mathrm{7}} +\mathrm{10}\left(\frac{\mathrm{1}.\mathrm{3}.\mathrm{5}}{\mathrm{2}.\mathrm{4}.\mathrm{6}}\right)^{\mathrm{7}} +... \\ $$

Question Number 125781 Answers: 0 Comments: 0

∫_0 ^∞ ((√x)/(1−x^2 )).(1/(e^(2πx) −1))dx

$$\int_{\mathrm{0}} ^{\infty} \frac{\sqrt{{x}}}{\mathrm{1}−{x}^{\mathrm{2}} }.\frac{\mathrm{1}}{{e}^{\mathrm{2}\pi{x}} −\mathrm{1}}{dx} \\ $$

Question Number 125708 Answers: 1 Comments: 0

∫_0 ^∞ ((1−tanhx)/( ((tanhx))^(1/5) ))dx

$$\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{1}−{tanhx}}{\:\sqrt[{\mathrm{5}}]{{tanhx}}}{dx} \\ $$

Question Number 125652 Answers: 0 Comments: 0

Question Number 125585 Answers: 0 Comments: 5

((((1/(1!)))^2 −((1/(2!)))^2 +((1/(3!)))^2 −((1/(4!)))^2 +... )/(((1/(1!)))^2 +((1/(2!)))^2 +((1/(3!)))^2 +......))

$$\frac{\left(\frac{\mathrm{1}}{\mathrm{1}!}\right)^{\mathrm{2}} −\left(\frac{\mathrm{1}}{\mathrm{2}!}\right)^{\mathrm{2}} +\left(\frac{\mathrm{1}}{\mathrm{3}!}\right)^{\mathrm{2}} −\left(\frac{\mathrm{1}}{\mathrm{4}!}\right)^{\mathrm{2}} +...\:}{\left(\frac{\mathrm{1}}{\mathrm{1}!}\right)^{\mathrm{2}} +\left(\frac{\mathrm{1}}{\mathrm{2}!}\right)^{\mathrm{2}} +\left(\frac{\mathrm{1}}{\mathrm{3}!}\right)^{\mathrm{2}} +......} \\ $$

Question Number 125490 Answers: 0 Comments: 0

∫_0 ^1 ((x^3 −2)/((x^3 +1)^2 ))(√(x^3 −x^2 +1)) dx

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{3}} −\mathrm{2}}{\left({x}^{\mathrm{3}} +\mathrm{1}\right)^{\mathrm{2}} }\sqrt{{x}^{\mathrm{3}} −{x}^{\mathrm{2}} +\mathrm{1}}\:{dx} \\ $$

Question Number 125411 Answers: 0 Comments: 0

Σ_(n=1) ^∞ ((√n)/(n^2 +1))

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\sqrt{{n}}}{{n}^{\mathrm{2}} +\mathrm{1}} \\ $$

Question Number 125283 Answers: 0 Comments: 0

∫_0 ^1 ((x^2 sin^2 (log(x+1)))/((1+(√(1+x)))^2 ))dx

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{2}} {sin}^{\mathrm{2}} \left({log}\left({x}+\mathrm{1}\right)\right)}{\left(\mathrm{1}+\sqrt{\mathrm{1}+{x}}\right)^{\mathrm{2}} }{dx} \\ $$

Question Number 125167 Answers: 0 Comments: 1

f(x)=∫((cos(sinx)+cos^2 x)/(1+sinxsin(sinx)))dx Find f(1)

$${f}\left({x}\right)=\int\frac{{cos}\left({sinx}\right)+{cos}^{\mathrm{2}} {x}}{\mathrm{1}+{sinxsin}\left({sinx}\right)}{dx} \\ $$$${Find}\:{f}\left(\mathrm{1}\right) \\ $$

Question Number 125086 Answers: 0 Comments: 1

Question Number 125014 Answers: 0 Comments: 0

Question Number 124892 Answers: 0 Comments: 2

1−(1/(3.3!))+(1/(5.5!))−(1/(7.7!))+...

$$\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}.\mathrm{3}!}+\frac{\mathrm{1}}{\mathrm{5}.\mathrm{5}!}−\frac{\mathrm{1}}{\mathrm{7}.\mathrm{7}!}+... \\ $$

Question Number 124891 Answers: 0 Comments: 0

∫_0 ^(π/(24)) log(tanθ)dθ

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{24}}} {log}\left({tan}\theta\right){d}\theta \\ $$

Question Number 124861 Answers: 0 Comments: 0

((((1/2))!)/(((3/2))!))−((((3/2))!1!)/(((5/2))!))+((((5/2))!2!)/(((7/2))!))−....

$$\frac{\left(\frac{\mathrm{1}}{\mathrm{2}}\right)!}{\left(\frac{\mathrm{3}}{\mathrm{2}}\right)!}−\frac{\left(\frac{\mathrm{3}}{\mathrm{2}}\right)!\mathrm{1}!}{\left(\frac{\mathrm{5}}{\mathrm{2}}\right)!}+\frac{\left(\frac{\mathrm{5}}{\mathrm{2}}\right)!\mathrm{2}!}{\left(\frac{\mathrm{7}}{\mathrm{2}}\right)!}−.... \\ $$

Question Number 124718 Answers: 0 Comments: 1

Σ_(n=1) ^n n^2 log(n+1) (In explicit form )

$$\underset{{n}=\mathrm{1}} {\overset{{n}} {\sum}}{n}^{\mathrm{2}} {log}\left({n}+\mathrm{1}\right)\:\:\:\:\left({In}\:{explicit}\:{form}\:\right) \\ $$

Pg 47 Pg 48 Pg 49 Pg 50 Pg 51 Pg 52 Pg 53 Pg 54 Pg 55 Pg 56

Terms of Service

Contact: info@tinkutara.com