Question and Answers Forum

OthersQuestion and Answers: Page 46

Question Number 135906 Answers: 1 Comments: 0

Edit: this is already fixed. Therefore this thread is closed... There′s a glitch! Whenever you backspace from a line to delete it, you′ll end up directly in front of the first thing you typed, whatever it is. Also, is there a way for this app to run in portrait orrentation on tablets? Because I can′t seem to get it to rotate to that mode. I saw in your screenshots on the Google Play page that it was possible, but I can′t get it to work. I would perfer editing in that orrentation over the landscape rotation.

$${Edit}:\:{this}\:{is}\:{already}\:{fixed}.\:{Therefore}\:{this}\:{thread}\:{is}\:{closed}... \\ $$$$ \\ $$$$\mathrm{There}'\mathrm{s}\:\mathrm{a}\:\mathrm{glitch}! \\ $$$$\mathrm{Whenever}\:\mathrm{you}\:\mathrm{backspace}\:\mathrm{from}\:\mathrm{a}\:\mathrm{line}\:\mathrm{to}\:\mathrm{delete}\:\mathrm{it},\:\mathrm{you}'\mathrm{ll}\:\mathrm{end}\:\mathrm{up}\:\mathrm{directly}\:\mathrm{in}\:\mathrm{front}\:\mathrm{of}\:\mathrm{the}\:\mathrm{first}\:\mathrm{thing}\:\mathrm{you}\:\mathrm{typed}, \\ $$$$\mathrm{whatever}\:\mathrm{it}\:\mathrm{is}. \\ $$$$ \\ $$$$\mathrm{Also},\:\mathrm{is}\:\mathrm{there}\:\mathrm{a}\:\mathrm{way}\:\mathrm{for}\:\mathrm{this}\:\mathrm{app}\:\mathrm{to}\:\mathrm{run}\:\mathrm{in}\:\mathrm{portrait}\:\mathrm{orrentation}\:\mathrm{on}\:\mathrm{tablets}?\:\mathrm{Because}\:\mathrm{I}\:\mathrm{can}'\mathrm{t}\:\mathrm{seem}\:\mathrm{to}\:\mathrm{get}\:\mathrm{it}\:\mathrm{to}\:\mathrm{rotate} \\ $$$$\mathrm{to}\:\mathrm{that}\:\mathrm{mode}.\:\mathrm{I}\:\mathrm{saw}\:\mathrm{in}\:\mathrm{your}\:\mathrm{screenshots}\:\mathrm{on}\:\mathrm{the}\:\mathrm{Google}\:\mathrm{Play}\:\mathrm{page}\:\mathrm{that}\:\mathrm{it}\:\mathrm{was}\:\mathrm{possible},\:\mathrm{but}\:\mathrm{I}\:\mathrm{can}'\mathrm{t}\:\mathrm{get}\:\mathrm{it}\:\mathrm{to}\:\mathrm{work}. \\ $$$$\mathrm{I}\:\mathrm{would}\:\mathrm{perfer}\:\mathrm{editing}\:\mathrm{in}\:\mathrm{that}\:\mathrm{orrentation}\:\mathrm{over}\:\mathrm{the}\:\mathrm{landscape}\:\mathrm{rotation}. \\ $$

Question Number 135884 Answers: 1 Comments: 0

Σ_(n=1) ^∞ (H_n ^((7)) /n^2 )−(H_n ^((7)) /((n+1)^2 )) Σ_(k=1) ^n (1/k^m )=H_n ^((m))

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{H}_{{n}} ^{\left(\mathrm{7}\right)} }{{n}^{\mathrm{2}} }−\frac{{H}_{{n}} ^{\left(\mathrm{7}\right)} }{\left({n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{k}^{{m}} }={H}_{{n}} ^{\left({m}\right)} \\ $$

Question Number 135808 Answers: 0 Comments: 0

Question Number 135798 Answers: 0 Comments: 3

Question Number 135757 Answers: 1 Comments: 4

((1.1)/2)+(((1+(1/2))1)/2^2 )+(((1+(1/2)+(1/3))2)/2^3 )+(((1+(1/2)+(1/3)+(1/4))3)/2^4 )+(((1+(1/2)+(1/3)+(1/4)+(1/5))5)/2^5 )+...

Question Number 135653 Answers: 1 Comments: 9

Question Number 135638 Answers: 0 Comments: 0

Σ_(n=0) ^∞ ((((√5)−2)^(2n+1) )/((2n+1)^2 ))=(π^2 /(24))−(1/(12))log^2 (2+(√5))

$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\sqrt{\mathrm{5}}−\mathrm{2}\right)^{\mathrm{2}{n}+\mathrm{1}} }{\left(\mathrm{2}{n}+\mathrm{1}\right)^{\mathrm{2}} }=\frac{\pi^{\mathrm{2}} }{\mathrm{24}}−\frac{\mathrm{1}}{\mathrm{12}}{log}^{\mathrm{2}} \left(\mathrm{2}+\sqrt{\mathrm{5}}\right) \\ $$

Question Number 135432 Answers: 0 Comments: 0

Question Number 135431 Answers: 2 Comments: 0

Question Number 135366 Answers: 1 Comments: 0

∫_0 ^1 (1/( ((6x−15x^2 +20x^3 −15x^4 +6x^5 −x^6 ))^(1/6) ))dx=(π/3) Or ∫_0 ^1 (1/( ((kx−((k(k−1))/2)x^2 +((k(k−1)(k−2))/6)x^3 −...))^(1/k) ))dx=(π/(ksin((π/k))))

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\:\sqrt[{\mathrm{6}}]{\mathrm{6}{x}−\mathrm{15}{x}^{\mathrm{2}} +\mathrm{20}{x}^{\mathrm{3}} −\mathrm{15}{x}^{\mathrm{4}} +\mathrm{6}{x}^{\mathrm{5}} −{x}^{\mathrm{6}} }}{dx}=\frac{\pi}{\mathrm{3}} \\ $$$${Or} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{1}}{\:\sqrt[{{k}}]{{kx}−\frac{{k}\left({k}−\mathrm{1}\right)}{\mathrm{2}}{x}^{\mathrm{2}} +\frac{{k}\left({k}−\mathrm{1}\right)\left({k}−\mathrm{2}\right)}{\mathrm{6}}{x}^{\mathrm{3}} −...}}{dx}=\frac{\pi}{{ksin}\left(\frac{\pi}{{k}}\right)} \\ $$

Question Number 135335 Answers: 0 Comments: 0

Question Number 135284 Answers: 0 Comments: 3

2^x +4^x =9^x . Solve for x

$$\mathrm{2}^{{x}} +\mathrm{4}^{{x}} =\mathrm{9}^{{x}} .\:{Solve}\:{for}\:{x} \\ $$

Question Number 135246 Answers: 0 Comments: 3

Question Number 135187 Answers: 0 Comments: 0

Question Number 135169 Answers: 0 Comments: 0

What is the remainder when x^(81) +x^(49) +x^(25) + x^9 + x is divided by x^3 +x

$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{remainder}\:\mathrm{when}\: \\ $$$$\mathrm{x}^{\mathrm{81}} +\mathrm{x}^{\mathrm{49}} +\mathrm{x}^{\mathrm{25}} +\:\mathrm{x}^{\mathrm{9}} +\:\mathrm{x}\:\mathrm{is}\:\mathrm{divided} \\ $$$$\mathrm{by}\:\mathrm{x}^{\mathrm{3}} +\mathrm{x}\: \\ $$

Question Number 135165 Answers: 0 Comments: 1

Solve Brachistochrone Curve Problem

$${Solve}\:{Brachistochrone}\:{Curve}\:{Problem} \\ $$

Question Number 134984 Answers: 2 Comments: 0

(1^2 /2)+(((1+(1/2))^2 )/2^2 )+(((1+(1/2)+(1/3))^2 )/2^3 )+(((1+(1/2)+(1/3)+(1/4))^2 )/2^4 )+... Find in a closed form

$$\frac{\mathrm{1}^{\mathrm{2}} }{\mathrm{2}}+\frac{\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} }{\mathrm{2}^{\mathrm{2}} }+\frac{\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} }{\mathrm{2}^{\mathrm{3}} }+\frac{\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{4}}\right)^{\mathrm{2}} }{\mathrm{2}^{\mathrm{4}} }+... \\ $$$$ \\ $$Find in a closed form

Question Number 134957 Answers: 0 Comments: 8

Question Number 134941 Answers: 0 Comments: 1

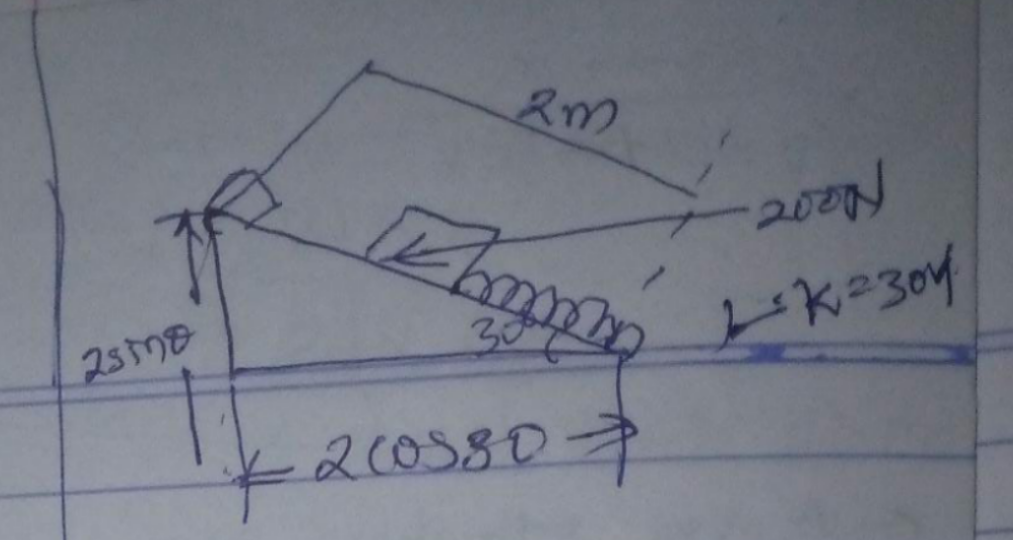
Question Number 134940 Answers: 0 Comments: 0

let X=R wth the usual metric. prove that the open and bounded interval A=(1,2) is an open set in R.

$${let}\:{X}=\mathbb{R}\:{wth}\:{the}\:{usual}\:{metric}.\:{prove}\:{that}\:{the}\:{open} \\ $$$${and}\:{bounded}\:{interval}\:{A}=\left(\mathrm{1},\mathrm{2}\right)\:{is}\:{an}\:{open}\:{set}\:{in}\:\mathbb{R}. \\ $$

Question Number 134915 Answers: 1 Comments: 0

Question Number 134704 Answers: 1 Comments: 0

Question Number 134657 Answers: 0 Comments: 0

(1/(πe))+(3/(2π^2 e^2 ))+((11)/(6π^3 e^3 ))+((25)/(12π^4 e^4 ))+((137)/(60π^5 e^5 ))+...=((log(π)+1−log(πe−1))/(πe−1))

$$\frac{\mathrm{1}}{\pi{e}}+\frac{\mathrm{3}}{\mathrm{2}\pi^{\mathrm{2}} {e}^{\mathrm{2}} }+\frac{\mathrm{11}}{\mathrm{6}\pi^{\mathrm{3}} {e}^{\mathrm{3}} }+\frac{\mathrm{25}}{\mathrm{12}\pi^{\mathrm{4}} {e}^{\mathrm{4}} }+\frac{\mathrm{137}}{\mathrm{60}\pi^{\mathrm{5}} {e}^{\mathrm{5}} }+...=\frac{{log}\left(\pi\right)+\mathrm{1}−{log}\left(\pi{e}−\mathrm{1}\right)}{\pi{e}−\mathrm{1}} \\ $$

Question Number 134530 Answers: 1 Comments: 0

The speed of a train is reduced from 80km/hr to 40km/hr in a distance of 500m on applying the brakes. (i) How much further will the train travels before coming to rest. (ii) Assuming the retardation remains constant, how long will it take to bring the train to rest after the application of the brakes?

$$\mathrm{The}\:\mathrm{speed}\:\mathrm{of}\:\mathrm{a}\:\mathrm{train}\:\mathrm{is}\:\mathrm{reduced}\:\mathrm{from}\:\:\mathrm{80km}/\mathrm{hr}\:\:\mathrm{to}\:\:\mathrm{40km}/\mathrm{hr}\:\:\mathrm{in}\:\mathrm{a} \\ $$$$\mathrm{distance}\:\mathrm{of}\:\:\mathrm{500m}\:\:\mathrm{on}\:\mathrm{applying}\:\mathrm{the}\:\mathrm{brakes}. \\ $$$$\left(\mathrm{i}\right)\:\:\mathrm{How}\:\mathrm{much}\:\mathrm{further}\:\mathrm{will}\:\mathrm{the}\:\mathrm{train}\:\mathrm{travels}\:\mathrm{before}\:\mathrm{coming}\:\mathrm{to}\:\mathrm{rest}. \\ $$$$\left(\mathrm{ii}\right)\:\:\mathrm{Assuming}\:\mathrm{the}\:\mathrm{retardation}\:\mathrm{remains}\:\mathrm{constant},\:\mathrm{how}\:\mathrm{long}\:\mathrm{will}\:\mathrm{it} \\ $$$$\mathrm{take}\:\mathrm{to}\:\mathrm{bring}\:\mathrm{the}\:\mathrm{train}\:\mathrm{to}\:\mathrm{rest}\:\mathrm{after}\:\mathrm{the}\:\mathrm{application}\:\mathrm{of}\:\mathrm{the}\:\mathrm{brakes}? \\ $$

Question Number 134453 Answers: 0 Comments: 0

Question Number 134439 Answers: 1 Comments: 0

sin1−((sin2)/2)+((sin3)/3)−((sin4)/4)+...=(1/2)

$${sin}\mathrm{1}−\frac{{sin}\mathrm{2}}{\mathrm{2}}+\frac{{sin}\mathrm{3}}{\mathrm{3}}−\frac{{sin}\mathrm{4}}{\mathrm{4}}+...=\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Pg 41 Pg 42 Pg 43 Pg 44 Pg 45 Pg 46 Pg 47 Pg 48 Pg 49 Pg 50

Contact: info@tinkutara.com