
OthersQuestion and Answers: Page 21
Question Number 182779 Answers: 4 Comments: 1
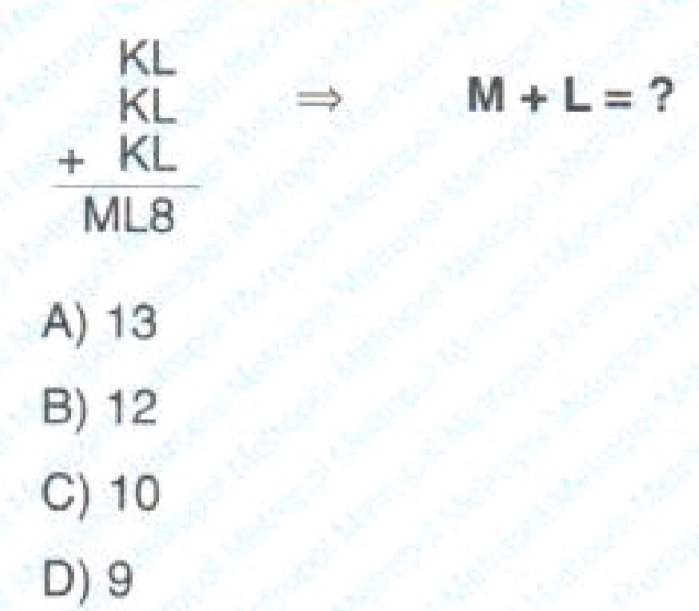
Question Number 182576 Answers: 0 Comments: 0

Question Number 182566 Answers: 0 Comments: 0
Question Number 182534 Answers: 1 Comments: 3
Question Number 182423 Answers: 4 Comments: 0

Question Number 182394 Answers: 1 Comments: 1

Question Number 182315 Answers: 0 Comments: 1

Question Number 182253 Answers: 0 Comments: 3

Question Number 182252 Answers: 0 Comments: 0

Question Number 182139 Answers: 1 Comments: 1
Question Number 182069 Answers: 1 Comments: 1
Question Number 182031 Answers: 0 Comments: 0

Question Number 181977 Answers: 0 Comments: 0
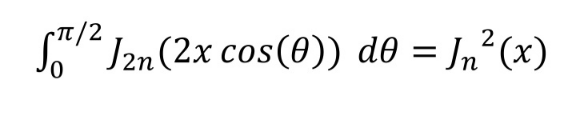
Question Number 181764 Answers: 3 Comments: 0
Question Number 181758 Answers: 0 Comments: 0
Question Number 181757 Answers: 1 Comments: 0
Question Number 181756 Answers: 2 Comments: 0
Question Number 181755 Answers: 1 Comments: 2
Question Number 181743 Answers: 1 Comments: 0
Question Number 181735 Answers: 2 Comments: 0
$$\int\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }−\mathrm{2x}}\mathrm{dx} \\ $$
Question Number 181723 Answers: 1 Comments: 0
Question Number 181750 Answers: 0 Comments: 1
$$\int\mathrm{xe}^{\mathrm{x}^{\mathrm{2}} +\mathrm{x}} \mathrm{dx} \\ $$$$ \\ $$$$. \\ $$
Question Number 181681 Answers: 0 Comments: 0

Question Number 181618 Answers: 2 Comments: 0
Question Number 181617 Answers: 0 Comments: 0
Question Number 181615 Answers: 1 Comments: 0
