Question and Answers Forum

OthersQuestion and Answers: Page 20

Question Number 182779 Answers: 4 Comments: 1

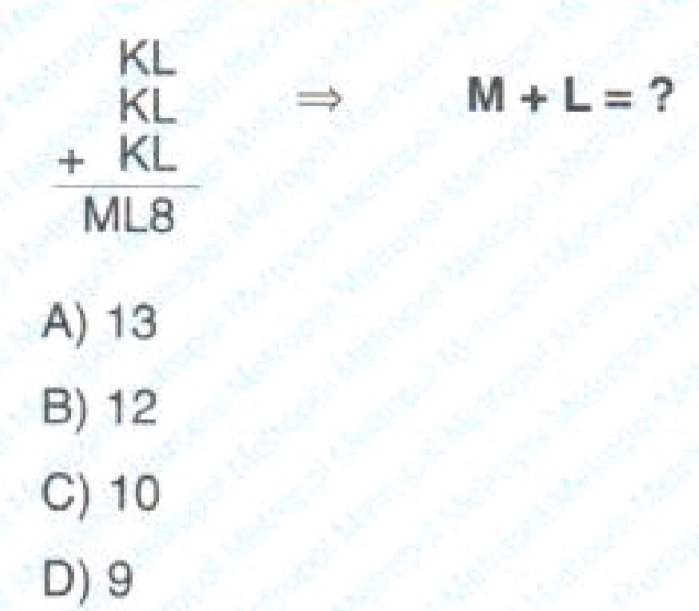
Question Number 182576 Answers: 0 Comments: 0

Question Number 182566 Answers: 0 Comments: 0

Solve (x^2 +( xy^2 )^(1/3) )(dy/dx) = y^2

$$\mathrm{Solve} \\ $$$$\left(\mathrm{x}^{\mathrm{2}} \:+\left(\:\mathrm{xy}^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \right)\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:\mathrm{y}^{\mathrm{2}} \\ $$

Question Number 182534 Answers: 1 Comments: 3

xy + (xy^2 )^(1/3) = y^2 Solve

$$\mathrm{xy}\:+\:\left(\mathrm{xy}^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:=\:\mathrm{y}^{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{Solve} \\ $$

Question Number 182423 Answers: 4 Comments: 0

Question Number 182394 Answers: 1 Comments: 1

Question Number 182315 Answers: 0 Comments: 1

Question Number 182253 Answers: 0 Comments: 3

Question Number 182252 Answers: 0 Comments: 0

Question Number 182139 Answers: 1 Comments: 1

Find constant a, b, so that y(t)=(t+3)e^(2t) is solution of IVP y^′ =ay+e^(2t) , y(0)=b .

$$\mathrm{Find}\:\mathrm{constant}\:\mathrm{a},\:\mathrm{b},\:\mathrm{so}\:\mathrm{that} \\ $$$$\mathrm{y}\left(\mathrm{t}\right)=\left(\mathrm{t}+\mathrm{3}\right)\mathrm{e}^{\mathrm{2t}} \:\mathrm{is}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{IVP} \\ $$$$\mathrm{y}^{'} =\mathrm{ay}+\mathrm{e}^{\mathrm{2t}} ,\:\:\:\:\:\:\:\:\:\:\:\mathrm{y}\left(\mathrm{0}\right)=\mathrm{b} \\ $$$$ \\ $$$$. \\ $$

Question Number 182069 Answers: 1 Comments: 1

(1+x^2 )(dy/dx)+3xy=5x solve

$$\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{3xy}=\mathrm{5x} \\ $$$$ \\ $$$$\mathrm{solve} \\ $$

Question Number 182031 Answers: 0 Comments: 0

Question Number 181977 Answers: 0 Comments: 0

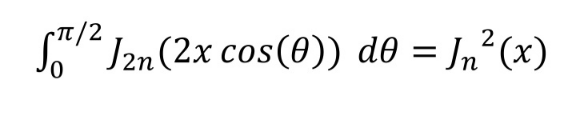
Question Number 181764 Answers: 3 Comments: 0

(dy/dx)+cos(x)y=cos(x) Solve

$$\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{cos}\left(\mathrm{x}\right)\mathrm{y}=\mathrm{cos}\left(\mathrm{x}\right) \\ $$$$ \\ $$$$\mathrm{Solve} \\ $$$$ \\ $$

Question Number 181758 Answers: 0 Comments: 0

How likely have at least two of 23 student birthday on the same day? M.m

$$\mathrm{How}\:\mathrm{likely}\:\mathrm{have}\:\mathrm{at}\:\mathrm{least}\:\mathrm{two}\:\mathrm{of}\:\mathrm{23} \\ $$$$\mathrm{student}\:\mathrm{birthday}\:\mathrm{on}\:\mathrm{the}\:\mathrm{same}\:\mathrm{day}? \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 181757 Answers: 1 Comments: 0

What is the largest digit of 11^5 ∙17^3 ∙33^3 .

$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{largest}\:\mathrm{digit}\:\mathrm{of}\:\mathrm{11}^{\mathrm{5}} \centerdot\mathrm{17}^{\mathrm{3}} \centerdot\mathrm{33}^{\mathrm{3}} \\ $$$$ \\ $$$$. \\ $$

Question Number 181756 Answers: 2 Comments: 0

Without using calculator, what is the value of Cos(((7π)/6))? .

$$\mathrm{Without}\:\mathrm{using}\:\mathrm{calculator},\:\mathrm{what}\:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{value}\:\mathrm{of}\:\mathrm{Cos}\left(\frac{\mathrm{7}\pi}{\mathrm{6}}\right)? \\ $$$$ \\ $$$$. \\ $$

Question Number 181755 Answers: 1 Comments: 2

Find the point of intersection (x,y) of f(x)=2x−3 and g(x)=−x^3 . .

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{point}\:\mathrm{of}\:\mathrm{intersection}\:\left(\mathrm{x},\mathrm{y}\right) \\ $$$$\mathrm{of}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{2x}−\mathrm{3}\:\mathrm{and}\:\mathrm{g}\left(\mathrm{x}\right)=−\mathrm{x}^{\mathrm{3}} . \\ $$$$ \\ $$$$. \\ $$

Question Number 181743 Answers: 1 Comments: 0

(dy/dx)+xy=xy^2 Solve

$$\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{xy}=\mathrm{xy}^{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{Solve} \\ $$

Question Number 181735 Answers: 2 Comments: 0

∫(1/( (√(1+x^2 ))−2x))dx

$$\int\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }−\mathrm{2x}}\mathrm{dx} \\ $$

Question Number 181723 Answers: 1 Comments: 0

(dy/dx)=((xy^2 +2y^3 )/(x^3 +x^2 y+xy^2 )) Solve

$$\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{xy}^{\mathrm{2}} +\mathrm{2y}^{\mathrm{3}} }{\mathrm{x}^{\mathrm{3}} +\mathrm{x}^{\mathrm{2}} \mathrm{y}+\mathrm{xy}^{\mathrm{2}} } \\ $$$$ \\ $$$$\mathrm{Solve} \\ $$$$ \\ $$

Question Number 181750 Answers: 0 Comments: 1

∫xe^(x^2 +x) dx .

$$\int\mathrm{xe}^{\mathrm{x}^{\mathrm{2}} +\mathrm{x}} \mathrm{dx} \\ $$$$ \\ $$$$. \\ $$

Question Number 181681 Answers: 0 Comments: 0

Question Number 181618 Answers: 2 Comments: 0

(dy/dx)+2xy=x^2 y(0)=3 Solve .

$$\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{2xy}=\mathrm{x}^{\mathrm{2}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{y}\left(\mathrm{0}\right)=\mathrm{3} \\ $$$$ \\ $$$$\mathrm{Solve} \\ $$$$ \\ $$$$. \\ $$

Question Number 181617 Answers: 0 Comments: 0

Solve: x(dy/dx)+(x+1)y=e^x^2 .

$$\mathrm{Solve}: \\ $$$$\mathrm{x}\frac{\mathrm{dy}}{\mathrm{dx}}+\left(\mathrm{x}+\mathrm{1}\right)\mathrm{y}=\mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \\ $$$$ \\ $$$$. \\ $$

Question Number 181615 Answers: 1 Comments: 0

Determine whether this is Homogenous or not (dy/dx)=(y/(y−2x)) .

$$\mathrm{Determine}\:\mathrm{whether}\:\mathrm{this}\:\mathrm{is}\:\mathrm{Homogenous} \\ $$$$\mathrm{or}\:\mathrm{not} \\ $$$$\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{y}}{\mathrm{y}−\mathrm{2x}} \\ $$$$ \\ $$$$. \\ $$

Pg 15 Pg 16 Pg 17 Pg 18 Pg 19 Pg 20 Pg 21 Pg 22 Pg 23 Pg 24

Contact: info@tinkutara.com