Question and Answers Forum

All Questions   Topic List

OthersQuestion and Answers: Page 19

Question Number 184126    Answers: 1   Comments: 0

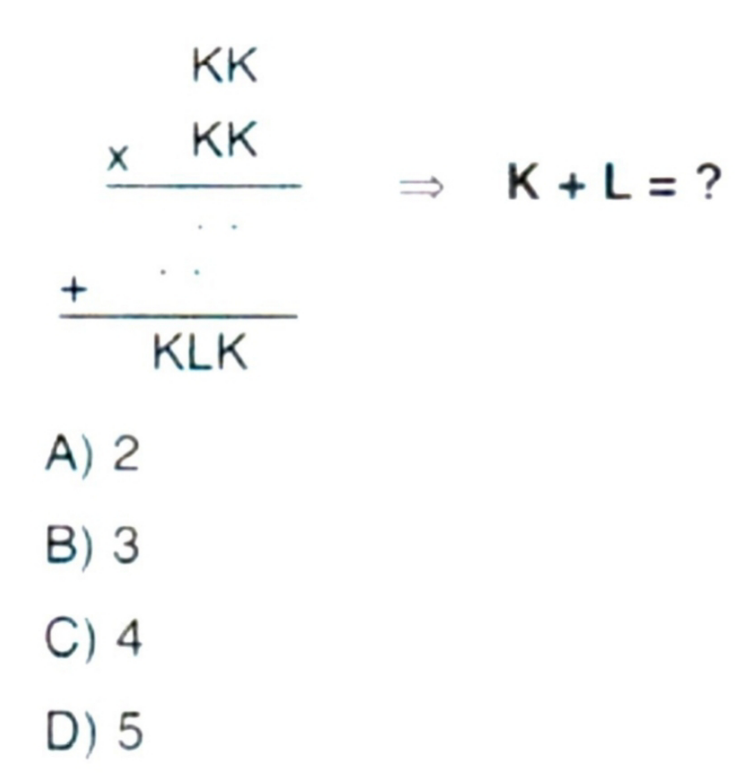
Question Number 184125    Answers: 0   Comments: 0

Question Number 184048    Answers: 5   Comments: 0

{ ((u_0 = 2)),((u_(n+1) = ((2u_n −1)/u_n ))) :} Find u_n .

$$\:\:\begin{cases}{{u}_{\mathrm{0}} \:=\:\mathrm{2}}\\{{u}_{{n}+\mathrm{1}} \:=\:\frac{\mathrm{2}{u}_{{n}} \:−\mathrm{1}}{{u}_{{n}} }}\end{cases} \\ $$$$\:\:\:{Find}\:{u}_{{n}} . \\ $$

Question Number 184030    Answers: 1   Comments: 0

How many words can be made from 5 letters if (a) all letters are different (b) 2 letters are identical (c) all letters are different but 2 partucular letters cannot be adjacent. M.m

$$\mathrm{How}\:\mathrm{many}\:\mathrm{words}\:\mathrm{can}\:\mathrm{be}\:\mathrm{made}\: \\ $$$$\mathrm{from}\:\mathrm{5}\:\mathrm{letters}\:\mathrm{if} \\ $$$$\left(\mathrm{a}\right)\:\mathrm{all}\:\mathrm{letters}\:\mathrm{are}\:\mathrm{different} \\ $$$$\left(\mathrm{b}\right)\:\mathrm{2}\:\mathrm{letters}\:\mathrm{are}\:\mathrm{identical} \\ $$$$\left(\mathrm{c}\right)\:\mathrm{all}\:\mathrm{letters}\:\mathrm{are}\:\mathrm{different}\:\mathrm{but}\:\mathrm{2} \\ $$$$\mathrm{partucular}\:\mathrm{letters}\:\mathrm{cannot}\:\mathrm{be} \\ $$$$\mathrm{adjacent}. \\ $$$$ \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 184028    Answers: 0   Comments: 0

H^(A^P P) Y Y_(E_A R) ! ⌊e⌋⌊i-i⌋⌊e⌋⌊𝛑⌋^(−)

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{H}}^{\boldsymbol{\mathrm{A}}^{\boldsymbol{\mathrm{P}}} \boldsymbol{\mathrm{P}}} \boldsymbol{\mathrm{Y}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{Y}}_{\boldsymbol{\mathrm{E}}_{\boldsymbol{\mathrm{A}}} \boldsymbol{\mathrm{R}}} \:! \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\overline {\lfloor\boldsymbol{\mathrm{e}}\rfloor\lfloor\boldsymbol{\mathrm{i}}-\boldsymbol{\mathrm{i}}\rfloor\lfloor\boldsymbol{\mathrm{e}}\rfloor\lfloor\boldsymbol{\pi}\rfloor}\:\: \\ $$

Question Number 184010    Answers: 0   Comments: 3

determinant ((( determinant (((2023))) )))_( is^ _(a number_(which is divisible_(by_(•_• ) ) ) ) ) (i)its sum of digits & (ii)its sum of squares of digits

$$\:\:\:\:\:\:\:\:\:\:\underset{\:\underset{\underset{\underset{\underset{\underset{\bullet} {\bullet}} {\boldsymbol{\mathrm{by}}}} {\boldsymbol{\mathrm{which}}\:\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{divisible}}}} {\boldsymbol{\mathrm{a}}\:\boldsymbol{\mathrm{number}}}} {\boldsymbol{\mathrm{is}}^{\:} }} {\begin{array}{|c|}{\:\begin{array}{|c|}{\mathrm{2023}}\\\hline\end{array}\:}\\\hline\end{array}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\boldsymbol{\mathrm{i}}\right)\boldsymbol{\mathrm{its}}\:\boldsymbol{\mathrm{sum}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{digits}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\& \\ $$$$\:\:\:\:\:\:\:\left(\boldsymbol{\mathrm{ii}}\right)\boldsymbol{\mathrm{its}}\:\boldsymbol{\mathrm{sum}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{squares}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{digits}} \\ $$

Question Number 183913    Answers: 0   Comments: 0

Question Number 183737    Answers: 2   Comments: 0

Question Number 183687    Answers: 3   Comments: 0

∫(1/(lnx))dx Help out

$$\int\frac{\mathrm{1}}{\mathrm{lnx}}\mathrm{dx} \\ $$$$ \\ $$$$ \\ $$$$\mathrm{Help}\:\mathrm{out} \\ $$

Question Number 183667    Answers: 0   Comments: 8

If w is one of the complex cube roots of unity, show that (a+wb+w^2 c)(a+w^2 b+wc) is equal to (α^2 +b^2 +c^2 −ab−bc−cα). Kindly help me out, Thank you.

$$\mathrm{If}\:\mathrm{w}\:\mathrm{is}\:\mathrm{one}\:\mathrm{of}\:\mathrm{the}\:\mathrm{complex}\:\mathrm{cube}\: \\ $$$$\mathrm{roots}\:\mathrm{of}\:\mathrm{unity},\:\mathrm{show}\:\mathrm{that} \\ $$$$\left(\mathrm{a}+\mathrm{wb}+\mathrm{w}^{\mathrm{2}} \mathrm{c}\right)\left(\mathrm{a}+\mathrm{w}^{\mathrm{2}} \mathrm{b}+\mathrm{wc}\right)\:\mathrm{is}\:\mathrm{equal} \\ $$$$\mathrm{to}\:\left(\alpha^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} −\mathrm{ab}−\mathrm{bc}−\mathrm{c}\alpha\right). \\ $$$$ \\ $$$$ \\ $$$$\mathrm{Kindly}\:\mathrm{help}\:\mathrm{me}\:\mathrm{out},\:\mathrm{Thank}\:\mathrm{you}. \\ $$

Question Number 183600    Answers: 1   Comments: 3

y′^(′′) + 8y′^(′′) +12y′ = 0 Solve with better explanation

$$\mathrm{y}'^{''} \:+\:\mathrm{8y}'^{''} \:+\mathrm{12y}'\:=\:\mathrm{0} \\ $$$$ \\ $$$$\mathrm{Solve}\:\mathrm{with}\:\mathrm{better}\:\mathrm{explanation} \\ $$

Question Number 183465    Answers: 1   Comments: 0

Find the least value of (1−2x)(1−x). M.m

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{least}\:\mathrm{value}\:\mathrm{of}\: \\ $$$$\left(\mathrm{1}−\mathrm{2x}\right)\left(\mathrm{1}−\mathrm{x}\right). \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 183464    Answers: 2   Comments: 0

Find the Maximum value of 3x(4−x) M.m

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{Maximum}\:\mathrm{value}\:\mathrm{of} \\ $$$$\mathrm{3x}\left(\mathrm{4}−\mathrm{x}\right) \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 183211    Answers: 1   Comments: 0

y^((iv)) +16y^((iii)) +9y^((ii)) +256y^((i)) +256y=0 M.m

$$\mathrm{y}^{\left(\mathrm{iv}\right)} +\mathrm{16y}^{\left(\mathrm{iii}\right)} +\mathrm{9y}^{\left(\mathrm{ii}\right)} +\mathrm{256y}^{\left(\mathrm{i}\right)} +\mathrm{256y}=\mathrm{0} \\ $$$$ \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 183210    Answers: 1   Comments: 0

(d^3 y/dx^3 )+4(d^2 y/dx^2 )+(dy/dx)−6y=0 M.m

$$\frac{\mathrm{d}^{\mathrm{3}} \mathrm{y}}{\mathrm{dx}^{\mathrm{3}} }+\mathrm{4}\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }+\frac{\mathrm{dy}}{\mathrm{dx}}−\mathrm{6y}=\mathrm{0} \\ $$$$ \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 183209    Answers: 1   Comments: 0

Solve the Differential equation below (d^3 y/dx^3 )+8(d^2 y/dx^2 )+12(dy/dx)=0 M.m

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{Differential}\:\mathrm{equation}\:\mathrm{below} \\ $$$$\frac{\mathrm{d}^{\mathrm{3}} \mathrm{y}}{\mathrm{dx}^{\mathrm{3}} }+\mathrm{8}\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }+\mathrm{12}\frac{\mathrm{dy}}{\mathrm{dx}}=\mathrm{0} \\ $$$$ \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 183117    Answers: 2   Comments: 0

Question Number 183101    Answers: 1   Comments: 3

∫((x+4y)/(2x^2 +9xy))dx M.m

$$\int\frac{\mathrm{x}+\mathrm{4y}}{\mathrm{2x}^{\mathrm{2}} +\mathrm{9xy}}\mathrm{dx} \\ $$$$ \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 183073    Answers: 2   Comments: 0

∫sin^2 x dx Integrate this question

$$\int\mathrm{sin}^{\mathrm{2}} \mathrm{x}\:\mathrm{dx} \\ $$$$ \\ $$$$\mathrm{Integrate}\:\mathrm{this}\:\mathrm{question} \\ $$

Question Number 183135    Answers: 1   Comments: 0

Question Number 183039    Answers: 1   Comments: 0

A Golfer practising on a range with an accelerated tree 4.9m above the fairway is able to strike a ball so that it leaves the club with a horizontal velocity of 20m/s. (Assume the acceleration due to gravity is 9.8m/s^2 and the effect of air resistance maybe ignored unless othewise stated 1) How long after the ball leaves the club will it land on the fairway? 2) What horizontal distance will the ball travel before striking the fairway? 3) What is the acceleration of the ball 0.5s after being hit? 4) Calculate the speed of the ball 0.8s after it leaves the club? M.m

$$\mathrm{A}\:\mathrm{Golfer}\:\mathrm{practising}\:\mathrm{on}\:\mathrm{a}\:\mathrm{range} \\ $$$$\mathrm{with}\:\mathrm{an}\:\mathrm{accelerated}\:\mathrm{tree}\:\mathrm{4}.\mathrm{9m}\:\mathrm{above} \\ $$$$\mathrm{the}\:\mathrm{fairway}\:\mathrm{is}\:\mathrm{able}\:\mathrm{to}\:\mathrm{strike}\:\mathrm{a}\:\mathrm{ball} \\ $$$$\mathrm{so}\:\mathrm{that}\:\mathrm{it}\:\mathrm{leaves}\:\mathrm{the}\:\mathrm{club}\:\mathrm{with}\:\mathrm{a}\: \\ $$$$\mathrm{horizontal}\:\mathrm{velocity}\:\mathrm{of}\:\mathrm{20m}/\mathrm{s}. \\ $$$$\left(\mathrm{Assume}\:\mathrm{the}\:\mathrm{acceleration}\:\mathrm{due}\:\mathrm{to}\:\right. \\ $$$$\mathrm{gravity}\:\mathrm{is}\:\mathrm{9}.\mathrm{8m}/\mathrm{s}^{\mathrm{2}} \:\mathrm{and}\:\mathrm{the}\:\mathrm{effect}\:\mathrm{of}\:\mathrm{air} \\ $$$$\mathrm{resistance}\:\mathrm{maybe}\:\mathrm{ignored}\:\mathrm{unless} \\ $$$$\mathrm{othewise}\:\mathrm{stated}\: \\ $$$$\left.\mathrm{1}\right)\:\mathrm{How}\:\mathrm{long}\:\mathrm{after}\:\mathrm{the}\:\mathrm{ball}\:\mathrm{leaves}\:\mathrm{the} \\ $$$$\mathrm{club}\:\mathrm{will}\:\mathrm{it}\:\mathrm{land}\:\mathrm{on}\:\mathrm{the}\:\mathrm{fairway}? \\ $$$$\left.\mathrm{2}\right)\:\mathrm{What}\:\mathrm{horizontal}\:\mathrm{distance}\:\mathrm{will}\:\mathrm{the} \\ $$$$\mathrm{ball}\:\mathrm{travel}\:\mathrm{before}\:\mathrm{striking}\:\mathrm{the}\:\mathrm{fairway}? \\ $$$$\left.\mathrm{3}\right)\:\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{acceleration}\:\mathrm{of}\:\mathrm{the}\:\mathrm{ball} \\ $$$$\mathrm{0}.\mathrm{5s}\:\mathrm{after}\:\mathrm{being}\:\mathrm{hit}? \\ $$$$\left.\mathrm{4}\right)\:\mathrm{Calculate}\:\mathrm{the}\:\mathrm{speed}\:\mathrm{of}\:\mathrm{the}\:\mathrm{ball}\: \\ $$$$\mathrm{0}.\mathrm{8s}\:\mathrm{after}\:\mathrm{it}\:\mathrm{leaves}\:\mathrm{the}\:\mathrm{club}? \\ $$$$ \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 183038    Answers: 1   Comments: 0

Solve (dy/dx)+xy=x^2 M.m

$$\mathrm{Solve} \\ $$$$\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{xy}=\mathrm{x}^{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 183036    Answers: 1   Comments: 0

A bullet of mass 1kg is fired and get embedded into a block of wood of mass 1kg initially at rest the velocity of the bullet before collision is 90m/s 1) What is the velocity of the system after collision? 2) Calculate the kinetic energy before and after the collision. 3)How much energy is lost in collision?

$$\mathrm{A}\:\mathrm{bullet}\:\mathrm{of}\:\mathrm{mass}\:\mathrm{1kg}\:\mathrm{is}\:\mathrm{fired}\:\mathrm{and}\:\mathrm{get} \\ $$$$\mathrm{embedded}\:\mathrm{into}\:\mathrm{a}\:\mathrm{block}\:\mathrm{of}\:\mathrm{wood}\:\mathrm{of} \\ $$$$\mathrm{mass}\:\mathrm{1kg}\:\mathrm{initially}\:\mathrm{at}\:\mathrm{rest}\:\mathrm{the}\:\mathrm{velocity} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{bullet}\:\mathrm{before}\:\mathrm{collision}\:\mathrm{is}\:\mathrm{90m}/\mathrm{s} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{velocity}\:\mathrm{of}\:\mathrm{the}\:\mathrm{system} \\ $$$$\mathrm{after}\:\mathrm{collision}? \\ $$$$\left.\mathrm{2}\right)\:\mathrm{Calculate}\:\mathrm{the}\:\mathrm{kinetic}\:\mathrm{energy}\:\mathrm{before} \\ $$$$\mathrm{and}\:\mathrm{after}\:\mathrm{the}\:\mathrm{collision}. \\ $$$$\left.\mathrm{3}\right)\mathrm{How}\:\mathrm{much}\:\mathrm{energy}\:\mathrm{is}\:\mathrm{lost}\:\mathrm{in}\:\mathrm{collision}? \\ $$

Question Number 182984    Answers: 2   Comments: 0

Find the equation for the plane through the point A(6, 2, −4), B(−2, 4, 8), C(4, −2, 2). −Vector Analysis M.m

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{for}\:\mathrm{the}\:\mathrm{plane} \\ $$$$\mathrm{through}\:\mathrm{the}\:\mathrm{point}\:\mathrm{A}\left(\mathrm{6},\:\mathrm{2},\:−\mathrm{4}\right), \\ $$$$\mathrm{B}\left(−\mathrm{2},\:\mathrm{4},\:\mathrm{8}\right),\:\mathrm{C}\left(\mathrm{4},\:−\mathrm{2},\:\mathrm{2}\right).\:−\mathrm{Vector}\:\mathrm{Analysis} \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 182941    Answers: 0   Comments: 0

Solve: [x^2 +(xy^2 )^(1/3) ](dy/dx)=y M.m

$$\mathrm{Solve}: \\ $$$$\left[\mathrm{x}^{\mathrm{2}} +\left(\mathrm{xy}^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \right]\frac{\mathrm{dy}}{\mathrm{dx}}=\mathrm{y} \\ $$$$ \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 182934    Answers: 1   Comments: 0

Is that right ! IF : Σ_(k = 1) ^n (⌊(n/k)⌋−⌊((n−1)/k)⌋) = 2 so n is a prime number .

$$\:\:\:\:\:\:\:\:{Is}\:{that}\:{right}\:! \\ $$$$\:\:\:\:{IF}\:\::\: \\ $$$$\underset{{k}\:=\:\mathrm{1}} {\overset{{n}} {\sum}}\:\left(\lfloor\frac{{n}}{{k}}\rfloor−\lfloor\frac{{n}−\mathrm{1}}{{k}}\rfloor\right)\:=\:\mathrm{2} \\ $$$$\:\:\:{so}\:{n}\:{is}\:{a}\:{prime}\:{number}\:. \\ $$

  Pg 14      Pg 15      Pg 16      Pg 17      Pg 18      Pg 19      Pg 20      Pg 21      Pg 22      Pg 23   

Terms of Service

Privacy Policy

Contact: info@tinkutara.com