Question and Answers Forum

OthersQuestion and Answers: Page 124

Question Number 26424 Answers: 0 Comments: 0

The front of a train 80m long passes a signal at a speed of 72km/hr. If the rear of the train passes the signal 5s later, Find (a) The magnitude of the acceleration of the train. (b) The speed at which the rear of the train passes the signal.

$$\mathrm{The}\:\mathrm{front}\:\mathrm{of}\:\mathrm{a}\:\mathrm{train}\:\mathrm{80m}\:\mathrm{long}\:\mathrm{passes}\:\mathrm{a}\:\mathrm{signal}\:\mathrm{at}\:\mathrm{a}\:\mathrm{speed}\:\mathrm{of}\:\mathrm{72km}/\mathrm{hr}.\:\mathrm{If}\:\mathrm{the} \\ $$$$\mathrm{rear}\:\mathrm{of}\:\mathrm{the}\:\mathrm{train}\:\mathrm{passes}\:\mathrm{the}\:\mathrm{signal}\:\mathrm{5s}\:\mathrm{later},\:\mathrm{Find} \\ $$$$\left(\mathrm{a}\right)\:\mathrm{The}\:\mathrm{magnitude}\:\mathrm{of}\:\mathrm{the}\:\mathrm{acceleration}\:\mathrm{of}\:\mathrm{the}\:\mathrm{train}. \\ $$$$\left(\mathrm{b}\right)\:\mathrm{The}\:\mathrm{speed}\:\mathrm{at}\:\mathrm{which}\:\mathrm{the}\:\mathrm{rear}\:\mathrm{of}\:\mathrm{the}\:\mathrm{train}\:\mathrm{passes}\:\mathrm{the}\:\mathrm{signal}. \\ $$

Question Number 26382 Answers: 0 Comments: 1

Question Number 26381 Answers: 0 Comments: 9

Question Number 26380 Answers: 0 Comments: 0

Question Number 26352 Answers: 1 Comments: 0

x(x+9)=(x+3)(x+7)−10

$${x}\left({x}+\mathrm{9}\right)=\left({x}+\mathrm{3}\right)\left({x}+\mathrm{7}\right)−\mathrm{10} \\ $$

Question Number 26329 Answers: 1 Comments: 0

A small particle moving with a uniform acceleration a covers distances X and Y in the first two equal and consecutive intervals of time t. Show that a = ((Y − X)/t^2 )

$$\mathrm{A}\:\mathrm{small}\:\mathrm{particle}\:\mathrm{moving}\:\mathrm{with}\:\mathrm{a}\:\mathrm{uniform}\:\mathrm{acceleration}\:\mathrm{a}\:\mathrm{covers}\:\mathrm{distances}\: \\ $$$$\mathrm{X}\:\mathrm{and}\:\mathrm{Y}\:\mathrm{in}\:\mathrm{the}\:\mathrm{first}\:\mathrm{two}\:\mathrm{equal}\:\mathrm{and}\:\mathrm{consecutive}\:\mathrm{intervals}\:\mathrm{of}\:\mathrm{time}\:\mathrm{t}.\:\mathrm{Show}\:\mathrm{that} \\ $$$$\mathrm{a}\:=\:\frac{\mathrm{Y}\:−\:\mathrm{X}}{\mathrm{t}^{\mathrm{2}} } \\ $$

Question Number 26328 Answers: 1 Comments: 1

Three towns X, Y and Z are on a straight road and Y is the mid−way between X and Z. A motor cyclist moving with uniform acceleration passes X, Y and Z. The speed with which the motocyclist passes X and Z are 20m/s and 40m/s respectively. Find the speed with which the motorcyclist passes Y.

$$\mathrm{Three}\:\mathrm{towns}\:\mathrm{X},\:\mathrm{Y}\:\mathrm{and}\:\mathrm{Z}\:\mathrm{are}\:\mathrm{on}\:\mathrm{a}\:\mathrm{straight}\:\mathrm{road}\:\mathrm{and}\:\mathrm{Y}\:\mathrm{is}\:\mathrm{the}\:\mathrm{mid}−\mathrm{way}\:\mathrm{between} \\ $$$$\mathrm{X}\:\mathrm{and}\:\mathrm{Z}.\:\mathrm{A}\:\mathrm{motor}\:\mathrm{cyclist}\:\mathrm{moving}\:\mathrm{with}\:\mathrm{uniform}\:\mathrm{acceleration}\:\mathrm{passes}\:\mathrm{X},\:\mathrm{Y}\:\mathrm{and}\:\mathrm{Z}.\: \\ $$$$\mathrm{The}\:\mathrm{speed}\:\mathrm{with}\:\mathrm{which}\:\mathrm{the}\:\mathrm{motocyclist}\:\mathrm{passes}\:\mathrm{X}\:\mathrm{and}\:\mathrm{Z}\:\mathrm{are}\:\mathrm{20m}/\mathrm{s}\:\mathrm{and}\:\mathrm{40m}/\mathrm{s}\: \\ $$$$\mathrm{respectively}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{speed}\:\mathrm{with}\:\mathrm{which}\:\mathrm{the}\:\mathrm{motorcyclist}\:\mathrm{passes}\:\mathrm{Y}. \\ $$

Question Number 26320 Answers: 2 Comments: 3

solve x^2 −1=2^x

$$\mathrm{solve}\:{x}^{\mathrm{2}} −\mathrm{1}=\mathrm{2}^{{x}} \\ $$

Question Number 26312 Answers: 0 Comments: 6

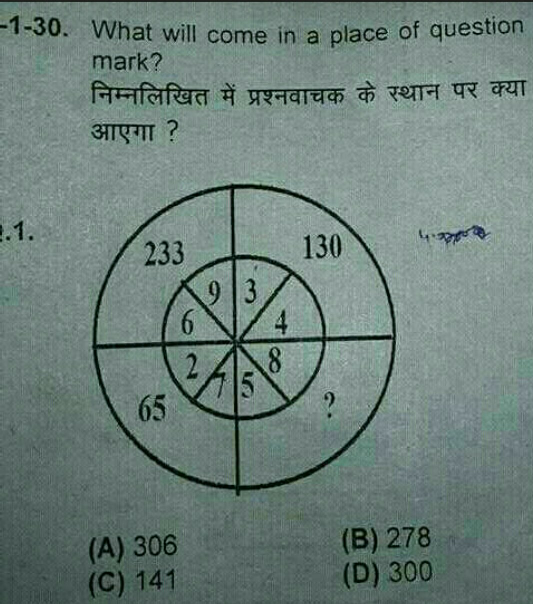
Question Number 26298 Answers: 0 Comments: 2

y=sin^4 x y′=? differential

$${y}=\mathrm{sin}\:^{\mathrm{4}} {x} \\ $$$${y}'=?\:{differential} \\ $$

Question Number 26354 Answers: 1 Comments: 0

Question Number 26262 Answers: 1 Comments: 0

(x+5) (x−2)=17−x find the value of x

$$\left(\mathrm{x}+\mathrm{5}\right)\:\left(\mathrm{x}−\mathrm{2}\right)=\mathrm{17}−\mathrm{x}\:\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{x} \\ $$

Question Number 26246 Answers: 0 Comments: 0

If normal plasma is 7.4 and normal CO_2 is 1.2mm, what is the normal (H_2 CO_3 ^− )

$$\mathrm{If}\:\:\mathrm{normal}\:\mathrm{plasma}\:\mathrm{is}\:\mathrm{7}.\mathrm{4}\:\mathrm{and}\:\mathrm{normal}\:\:\mathrm{CO}_{\mathrm{2}} \:\mathrm{is}\:\mathrm{1}.\mathrm{2mm},\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{normal}\:\left(\mathrm{H}_{\mathrm{2}} \mathrm{CO}_{\mathrm{3}} ^{−} \right) \\ $$

Question Number 26174 Answers: 0 Comments: 0

Question Number 26166 Answers: 0 Comments: 0

Question Number 26156 Answers: 1 Comments: 0

a two digit number is 3 more than 4 times the sum of its digits. if 18 is added to this number .the sum is equal to number obtained by interchanging the digits.find the number

$${a}\:{two}\:{digit}\:{number}\:{is}\:\mathrm{3}\:{more}\:{than} \\ $$$$\mathrm{4}\:{times}\:{the}\:{sum}\:{of}\:{its}\:{digits}.\:{if} \\ $$$$\mathrm{18}\:{is}\:{added}\:{to}\:{this}\:{number}\:.{the}\: \\ $$$${sum}\:{is}\:{equal}\:{to}\:{number}\:{obtained} \\ $$$${by}\:{interchanging}\:{the}\:{digits}.{find}\:{the}\:{number} \\ $$

Question Number 26113 Answers: 1 Comments: 0

Question Number 26087 Answers: 0 Comments: 2

Given f(x) = (1 − x + x^2 − x^3 + ... − x^(2015) + x^(2016) )^2 Find the sum of all odd coeffisiens! Ex. f(x) = (x^2 + x + 1)^2 = 1x^4 + 2x^3 + 3x^2 + 2x + 1 The sum of odd coeffisien is 1 + 3 = 4

$$\mathrm{Given} \\ $$$${f}\left({x}\right)\:=\:\left(\mathrm{1}\:−\:{x}\:+\:{x}^{\mathrm{2}} \:−\:{x}^{\mathrm{3}} \:+\:...\:−\:{x}^{\mathrm{2015}} \:+\:{x}^{\mathrm{2016}} \right)^{\mathrm{2}} \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{all}\:\mathrm{odd}\:\mathrm{coeffisiens}! \\ $$$$ \\ $$$$\mathrm{Ex}.\:{f}\left({x}\right)\:=\:\left({x}^{\mathrm{2}} \:+\:{x}\:+\:\mathrm{1}\right)^{\mathrm{2}} \:=\:\mathrm{1}{x}^{\mathrm{4}} \:+\:\mathrm{2}{x}^{\mathrm{3}} \:+\:\mathrm{3}{x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:+\:\mathrm{1} \\ $$$$\mathrm{The}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{odd}\:\mathrm{coeffisien}\:\mathrm{is}\:\mathrm{1}\:+\:\mathrm{3}\:=\:\mathrm{4} \\ $$

Question Number 26078 Answers: 1 Comments: 0

x^2 −x−42 factorise

$$\mathrm{x}^{\mathrm{2}} −\mathrm{x}−\mathrm{42}\:\mathrm{factorise} \\ $$

Question Number 26058 Answers: 0 Comments: 0