Question and Answers Forum

OthersQuestion and Answers: Page 121

Question Number 31340 Answers: 0 Comments: 0

Deduce the power series of sin^2 x. Hence show that if x is small then (sin^2 x − x^2 cosx)/x^4 =(1/6) − (x^2 /(360))

$${Deduce}\:{the}\:{power}\:{series}\:{of}\:{sin}^{\mathrm{2}} {x}. \\ $$$${Hence}\:{show}\:{that}\:{if}\:{x}\:{is}\:{small}\:{then} \\ $$$$\left({sin}^{\mathrm{2}} {x}\:−\:{x}^{\mathrm{2}} {cosx}\right)/{x}^{\mathrm{4}} =\frac{\mathrm{1}}{\mathrm{6}}\:−\:\frac{{x}^{\mathrm{2}} }{\mathrm{360}} \\ $$

Question Number 31314 Answers: 0 Comments: 0

let x={(1/n)}_(n=1) ^∞ and y={(1/(n+1))}_(n=1) ^∞ be a sequence of real numbers and l_(2 ) ={x=(x_1 ,x_2 ,x_3 ,...):Σ_(n=1) ^∞ ∣xi∣^2 <∞} a linear space. (1) verify that x and y are in l_2 . (2) compute the inner product of x and y on l_2 please help me solve this question.

$${let}\:{x}=\left\{\frac{\mathrm{1}}{{n}}\right\}_{{n}=\mathrm{1}} ^{\infty} {and}\:{y}=\left\{\frac{\mathrm{1}}{{n}+\mathrm{1}}\right\}_{{n}=\mathrm{1}} ^{\infty} {be}\: \\ $$$${a}\:{sequence}\:{of}\:{real}\:{numbers}\:{and} \\ $$$${l}_{\mathrm{2}\:} =\left\{{x}=\left({x}_{\mathrm{1}} ,{x}_{\mathrm{2}} ,{x}_{\mathrm{3}} ,...\right):\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\mid{xi}\mid^{\mathrm{2}} <\infty\right\} \\ $$$${a}\:{linear}\:{space}.\: \\ $$$$\left(\mathrm{1}\right)\:{verify}\:{that}\:{x}\:{and}\:{y}\:{are}\:{in}\:{l}_{\mathrm{2}} . \\ $$$$\left(\mathrm{2}\right)\:{compute}\:{the}\:{inner}\:{product}\:{of}\:{x}\: \\ $$$${and}\:{y}\:{on}\:{l}_{\mathrm{2}} \\ $$$$\boldsymbol{{please}}\:\boldsymbol{{help}}\:\boldsymbol{{me}}\:\boldsymbol{{solve}}\:\boldsymbol{{this}}\: \\ $$$$\boldsymbol{{que}}{s}\boldsymbol{{tion}}. \\ $$

Question Number 31306 Answers: 1 Comments: 0

Complete the square in y^2 +8y+9k and hence find the value of k that makes it a perfect square.

$$\mathrm{Complete}\:\mathrm{the}\:\mathrm{square}\:\mathrm{in}\:\mathrm{y}^{\mathrm{2}} \:+\mathrm{8y}+\mathrm{9k}\:\mathrm{and}\:\mathrm{hence}\:\mathrm{find}\:\mathrm{the} \\ $$$$\mathrm{value}\:\mathrm{of}\:\mathrm{k}\:\mathrm{that}\:\mathrm{makes}\:\mathrm{it}\:\mathrm{a}\:\mathrm{perfect}\:\mathrm{square}. \\ $$

Question Number 31281 Answers: 1 Comments: 0

find three nos in AP whose product is equal to the square of their sum.

$${find}\:{three}\:{nos}\:{in}\:{AP}\:{whose}\:{product} \\ $$$${is}\:{equal}\:{to}\:{the}\:{square}\:{of}\:{their}\:{sum}. \\ $$

Question Number 31085 Answers: 0 Comments: 1

calculate ∫∫_(x^2 +y^2 −2x≤0) xdxdy.

$${calculate}\:\int\int_{{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} \:−\mathrm{2}{x}\leqslant\mathrm{0}} {xdxdy}. \\ $$

Question Number 31017 Answers: 1 Comments: 0

solve (√(1+tan^2 x/1+cot^2 x= tanx))

$${solve}\:\sqrt{\mathrm{1}+{tan}^{\mathrm{2}} {x}/\mathrm{1}+{cot}^{\mathrm{2}} {x}=\:\:\:{tanx}} \\ $$

Question Number 30771 Answers: 0 Comments: 0

let I_n = ∫_0 ^(π/4) (dx/(cos^(2n+1) )) (n∈N) 1) find a and b fromR /∀x∈[0,(π/4)] (1/(cosx))=((acosx)/(1−sinx)) +((bcosx)/(1+sinx)) .find I_0 2) verify the relation (1/(cos^(2n+3) x))=(1/(cos^(2n+1) x)) +((sinx sinx)/(cos^(2n+3) )) .find the relation of recurrence between I_n and I_(n+1) .

$${let}\:\:{I}_{{n}} =\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{{dx}}{{cos}^{\mathrm{2}{n}+\mathrm{1}} }\:\:\:\:\left({n}\in{N}\right) \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{and}\:{b}\:{fromR}\:/\forall{x}\in\left[\mathrm{0},\frac{\pi}{\mathrm{4}}\right] \\ $$$$\frac{\mathrm{1}}{{cosx}}=\frac{{acosx}}{\mathrm{1}−{sinx}}\:+\frac{{bcosx}}{\mathrm{1}+{sinx}}\:\:.{find}\:\:{I}_{\mathrm{0}} \\ $$$$\left.\mathrm{2}\right)\:{verify}\:{the}\:{relation} \\ $$$$\frac{\mathrm{1}}{{cos}^{\mathrm{2}{n}+\mathrm{3}} {x}}=\frac{\mathrm{1}}{{cos}^{\mathrm{2}{n}+\mathrm{1}} {x}}\:+\frac{{sinx}\:{sinx}}{{cos}^{\mathrm{2}{n}+\mathrm{3}} }\:.{find}\:{the}\:{relation} \\ $$$${of}\:{recurrence}\:{between}\:{I}_{{n}} \:{and}\:{I}_{{n}+\mathrm{1}} \:\:. \\ $$

Question Number 30558 Answers: 0 Comments: 0

find I= ∫_(1/2) ^1 arctan((√(1−x^2 )) dx .

$${find}\:{I}=\:\int_{\frac{\mathrm{1}}{\mathrm{2}}} ^{\mathrm{1}} \:\:{arctan}\left(\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\:\:\:{dx}\:\:.\right. \\ $$

Question Number 30424 Answers: 0 Comments: 0

find Σ_(n=0) ^∞ (x^n /(3n+2)) for ∣x∣<1 then find Σ_(n=0) ^∞ (((−1)^n )/((3n+2)2^n )) .

$${find}\:\:\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\:\:\frac{{x}^{{n}} }{\mathrm{3}{n}+\mathrm{2}}\:\:\:{for}\:\:\mid{x}\mid<\mathrm{1}\:\:{then}\:{find}\: \\ $$$$\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left(\mathrm{3}{n}+\mathrm{2}\right)\mathrm{2}^{{n}} }\:. \\ $$

Question Number 30017 Answers: 1 Comments: 0

find the elements of the ellipse given the following equation 1.(x^2 /(25))+(y^2 /4)=1 2.x^2 +9y^2 =9

$$\mathrm{find}\:\mathrm{the}\:\mathrm{elements}\:\mathrm{of}\:\mathrm{the}\:\mathrm{ellipse}\:\mathrm{given}\:\mathrm{the}\:\mathrm{following}\:\mathrm{equation} \\ $$$$\mathrm{1}.\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{25}}+\frac{\mathrm{y}^{\mathrm{2}} }{\mathrm{4}}=\mathrm{1} \\ $$$$\mathrm{2}.\mathrm{x}^{\mathrm{2}} +\mathrm{9y}^{\mathrm{2}} =\mathrm{9} \\ $$

Question Number 29880 Answers: 0 Comments: 3

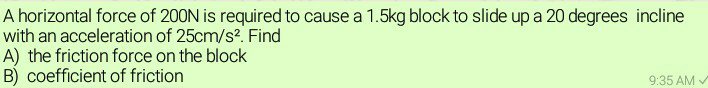
Question Number 29700 Answers: 1 Comments: 0

32^(32^(32) ) /7...find remainder

$$\mathrm{32}^{\mathrm{32}^{\mathrm{32}} } \:/\mathrm{7}...\mathrm{find}\:\mathrm{remainder} \\ $$

Question Number 29680 Answers: 1 Comments: 0

i^(i ) what is iota to power iota

$$\mathrm{i}^{\mathrm{i}\:} \:\:\mathrm{what}\:\mathrm{is}\:\mathrm{iota}\:\mathrm{to}\:\mathrm{power}\:\mathrm{iota} \\ $$

Question Number 29648 Answers: 1 Comments: 5

How many arrangements are there of 4 letters chosen from the word COMMUNICATION

$$\mathrm{How}\:\mathrm{many}\:\mathrm{arrangements}\:\mathrm{are} \\ $$$$\mathrm{there}\:\mathrm{of}\:\mathrm{4}\:\mathrm{letters}\:\mathrm{chosen}\:\mathrm{from}\: \\ $$$$\mathrm{the}\:\mathrm{word}\:{COMMUNICATION} \\ $$

Question Number 29607 Answers: 0 Comments: 0

Prove the convergence of each of the following sequence (i) {((n − 1)/n)}_(n = 1) ^∞ (ii) {(1/(2)^(1/n) )}_(n = 1) ^∞ (iii) {((n + 1)/n)}_(n = 1) ^∞

$$\mathrm{Prove}\:\mathrm{the}\:\mathrm{convergence}\:\mathrm{of}\:\mathrm{each}\:\mathrm{of}\:\mathrm{the}\:\mathrm{following}\:\mathrm{sequence} \\ $$$$\left(\mathrm{i}\right)\:\:\left\{\frac{\mathrm{n}\:−\:\mathrm{1}}{\mathrm{n}}\right\}_{\mathrm{n}\:=\:\mathrm{1}} ^{\infty} \:\:\:\:\:\:\:\:\left(\mathrm{ii}\right)\:\:\:\left\{\frac{\mathrm{1}}{\sqrt[{\mathrm{n}}]{\mathrm{2}}}\right\}_{\mathrm{n}\:=\:\mathrm{1}} ^{\infty} \:\:\:\:\:\:\left(\mathrm{iii}\right)\:\:\:\:\left\{\frac{\mathrm{n}\:+\:\mathrm{1}}{\mathrm{n}}\right\}_{\mathrm{n}\:=\:\mathrm{1}} ^{\infty} \\ $$

Question Number 29550 Answers: 1 Comments: 0

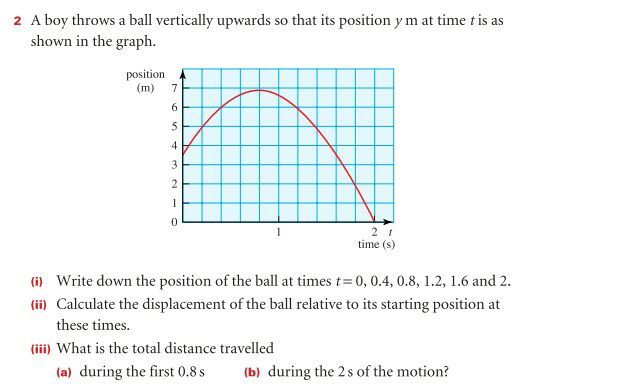
Question Number 29522 Answers: 1 Comments: 7

Question Number 29425 Answers: 1 Comments: 1

Question Number 29364 Answers: 0 Comments: 0

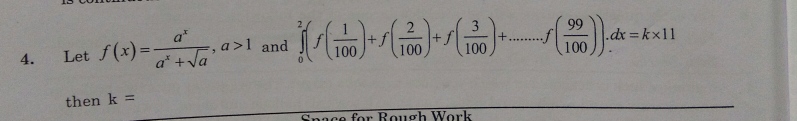
Question Number 29313 Answers: 0 Comments: 0

Question Number 29287 Answers: 0 Comments: 0

plz is there any app for medical forum just like this one

$${plz}\:{is}\:{there}\:{any}\:{app}\:{for}\:{medical} \\ $$$${forum}\:{just}\:{like}\:{this}\:{one} \\ $$

Question Number 29268 Answers: 0 Comments: 2

A man moves 20m North , then 12m East and finally 15m South.His displacement from the starting point is now (a) 13m (b) 27m (c) 47m (d) 23m

$$\mathrm{A}\:\mathrm{man}\:\mathrm{moves}\:\:\mathrm{20m}\:\:\mathrm{North}\:,\:\:\mathrm{then}\:\:\mathrm{12m}\:\mathrm{East}\:\:\mathrm{and}\:\:\mathrm{finally}\:\:\mathrm{15m}\:\:\mathrm{South}.\mathrm{His} \\ $$$$\mathrm{displacement}\:\mathrm{from}\:\mathrm{the}\:\mathrm{starting}\:\mathrm{point}\:\mathrm{is}\:\mathrm{now} \\ $$$$\left(\mathrm{a}\right)\:\mathrm{13m}\:\:\left(\mathrm{b}\right)\:\:\mathrm{27m}\:\:\left(\mathrm{c}\right)\:\:\mathrm{47m}\:\:\left(\mathrm{d}\right)\:\:\mathrm{23m} \\ $$

Question Number 29272 Answers: 0 Comments: 0

(2x−3x^3 )y^((2)) +4y^((1)) +6xy=0 this equation has an answer in the form of several sentences. get the public answer.

$$\left(\mathrm{2}{x}−\mathrm{3}{x}^{\mathrm{3}} \right){y}^{\left(\mathrm{2}\right)} +\mathrm{4}{y}^{\left(\mathrm{1}\right)} +\mathrm{6}{xy}=\mathrm{0} \\ $$$${this}\:{equation}\:{has}\:{an}\:{answer}\:{in}\:{the}\:{form}\:{of}\:{several}\:{sentences}. \\ $$$${get}\:{the}\:{public}\:{answer}. \\ $$

Question Number 29265 Answers: 0 Comments: 0

An electric pump with efficiency of 70% raises water to a height of 15m . If water is delivered at the rate of 350 dm^3 per minute. (i) what is the power rating of the pump ? (mass of 1 dm^3 = 1 kg) (ii) what is the energy lost by the pump ? (g = 10 m/s^2 ) (Answer: 1250W. 22.5 KJ)

$$\mathrm{An}\:\mathrm{electric}\:\mathrm{pump}\:\mathrm{with}\:\mathrm{efficiency}\:\mathrm{of}\:\:\:\mathrm{70\%}\:\:\mathrm{raises}\:\mathrm{water}\:\mathrm{to}\:\mathrm{a}\:\mathrm{height}\:\mathrm{of}\:\:\mathrm{15m} \\ $$$$.\:\mathrm{If}\:\mathrm{water}\:\mathrm{is}\:\mathrm{delivered}\:\mathrm{at}\:\mathrm{the}\:\mathrm{rate}\:\mathrm{of}\:\:\mathrm{350}\:\mathrm{dm}^{\mathrm{3}} \:\mathrm{per}\:\mathrm{minute}.\:\: \\ $$$$\left(\mathrm{i}\right)\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{power}\:\mathrm{rating}\:\mathrm{of}\:\mathrm{the}\:\mathrm{pump}\:?\:\:\:\left(\mathrm{mass}\:\mathrm{of}\:\mathrm{1}\:\mathrm{dm}^{\mathrm{3}} \:=\:\mathrm{1}\:\mathrm{kg}\right) \\ $$$$\left(\mathrm{ii}\right)\:\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{energy}\:\mathrm{lost}\:\mathrm{by}\:\mathrm{the}\:\mathrm{pump}\:?\:\:\:\left(\mathrm{g}\:=\:\mathrm{10}\:\mathrm{m}/\mathrm{s}^{\mathrm{2}} \right) \\ $$$$\left(\mathrm{Answer}:\:\:\:\mathrm{1250W}.\:\:\:\:\mathrm{22}.\mathrm{5}\:\mathrm{KJ}\right) \\ $$

Question Number 29222 Answers: 1 Comments: 0

3x−4y=12, xy=2

$$\mathrm{3}{x}−\mathrm{4}{y}=\mathrm{12},\:{xy}=\mathrm{2} \\ $$

Question Number 29209 Answers: 1 Comments: 4

Pg 116 Pg 117 Pg 118 Pg 119 Pg 120 Pg 121 Pg 122 Pg 123 Pg 124 Pg 125

Contact: info@tinkutara.com