
Question Number 2381 by Yozzi last updated on 18/Nov/15
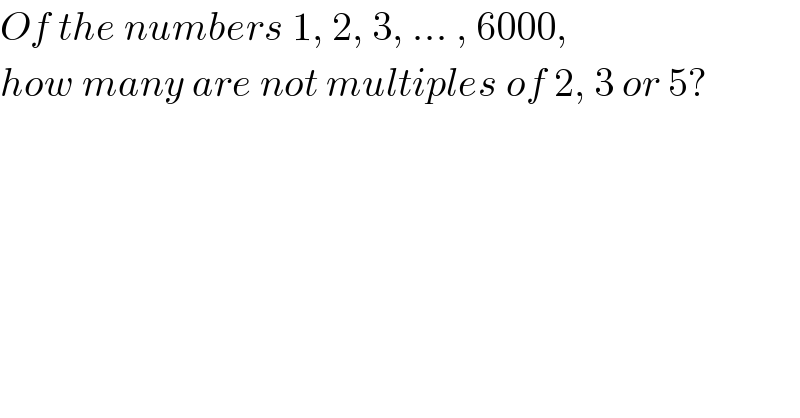
$${Of}\:{the}\:{numbers}\:\mathrm{1},\:\mathrm{2},\:\mathrm{3},\:...\:,\:\mathrm{6000}, \\ $$$${how}\:{many}\:{are}\:{not}\:{multiples}\:{of}\:\mathrm{2},\:\mathrm{3}\:{or}\:\mathrm{5}? \\ $$
Commented by 123456 last updated on 18/Nov/15
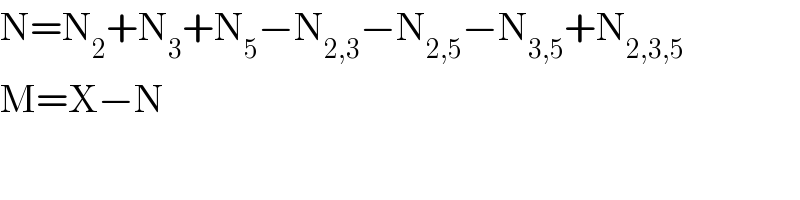
$$\mathrm{N}=\mathrm{N}_{\mathrm{2}} +\mathrm{N}_{\mathrm{3}} +\mathrm{N}_{\mathrm{5}} −\mathrm{N}_{\mathrm{2},\mathrm{3}} −\mathrm{N}_{\mathrm{2},\mathrm{5}} −\mathrm{N}_{\mathrm{3},\mathrm{5}} +\mathrm{N}_{\mathrm{2},\mathrm{3},\mathrm{5}} \\ $$$$\mathrm{M}=\mathrm{X}−\mathrm{N} \\ $$
Commented by prakash jain last updated on 18/Nov/15

$${n}\left(\mathrm{A}\cup\mathrm{B}\cup\mathrm{C}\right)={n}\left(\mathrm{A}\right)+{n}\left(\mathrm{B}\right)+{n}\left(\mathrm{C}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−{n}\left(\mathrm{A}\cap\mathrm{B}\right)−{n}\left(\mathrm{A}\cap\mathrm{C}\right)−{n}\left(\mathrm{B}\cap\mathrm{C}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+{n}\left(\mathrm{A}\cap\mathrm{B}\cap\mathrm{C}\right) \\ $$
Answered by 123456 last updated on 18/Nov/15
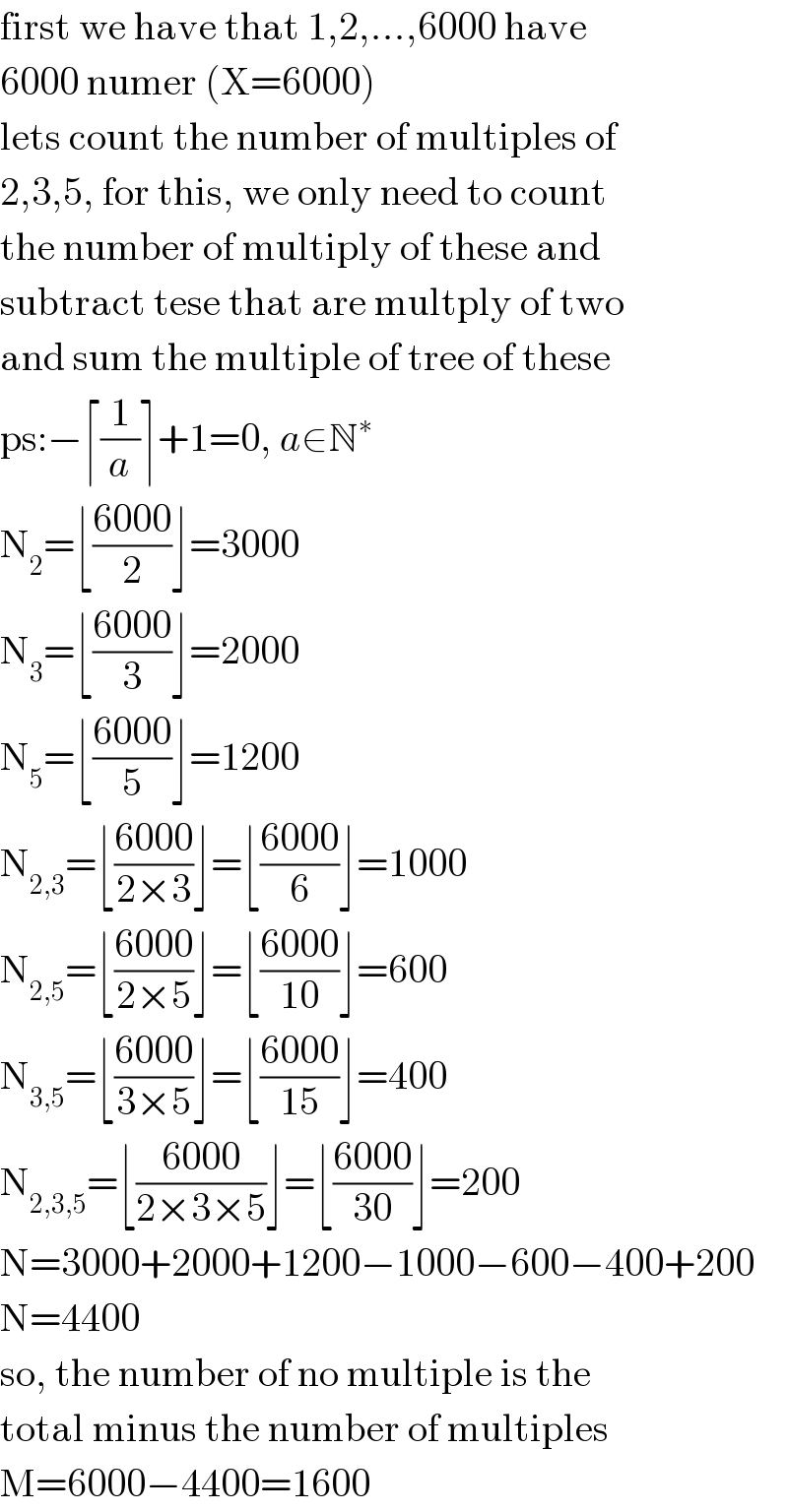
$$\mathrm{first}\:\mathrm{we}\:\mathrm{have}\:\mathrm{that}\:\mathrm{1},\mathrm{2},...,\mathrm{6000}\:\mathrm{have} \\ $$$$\mathrm{6000}\:\mathrm{numer}\:\left(\mathrm{X}=\mathrm{6000}\right) \\ $$$$\mathrm{lets}\:\mathrm{count}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{multiples}\:\mathrm{of} \\ $$$$\mathrm{2},\mathrm{3},\mathrm{5},\:\mathrm{for}\:\mathrm{this},\:\mathrm{we}\:\mathrm{only}\:\mathrm{need}\:\mathrm{to}\:\mathrm{count} \\ $$$$\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{multiply}\:\mathrm{of}\:\mathrm{these}\:\mathrm{and} \\ $$$$\mathrm{subtract}\:\mathrm{tese}\:\mathrm{that}\:\mathrm{are}\:\mathrm{multply}\:\mathrm{of}\:\mathrm{two} \\ $$$$\mathrm{and}\:\mathrm{sum}\:\mathrm{the}\:\mathrm{multiple}\:\mathrm{of}\:\mathrm{tree}\:\mathrm{of}\:\mathrm{these} \\ $$$$\mathrm{ps}:−\lceil\frac{\mathrm{1}}{{a}}\rceil+\mathrm{1}=\mathrm{0},\:{a}\in\mathbb{N}^{\ast} \\ $$$$\mathrm{N}_{\mathrm{2}} =\lfloor\frac{\mathrm{6000}}{\mathrm{2}}\rfloor=\mathrm{3000} \\ $$$$\mathrm{N}_{\mathrm{3}} =\lfloor\frac{\mathrm{6000}}{\mathrm{3}}\rfloor=\mathrm{2000} \\ $$$$\mathrm{N}_{\mathrm{5}} =\lfloor\frac{\mathrm{6000}}{\mathrm{5}}\rfloor=\mathrm{1200} \\ $$$$\mathrm{N}_{\mathrm{2},\mathrm{3}} =\lfloor\frac{\mathrm{6000}}{\mathrm{2}×\mathrm{3}}\rfloor=\lfloor\frac{\mathrm{6000}}{\mathrm{6}}\rfloor=\mathrm{1000} \\ $$$$\mathrm{N}_{\mathrm{2},\mathrm{5}} =\lfloor\frac{\mathrm{6000}}{\mathrm{2}×\mathrm{5}}\rfloor=\lfloor\frac{\mathrm{6000}}{\mathrm{10}}\rfloor=\mathrm{600} \\ $$$$\mathrm{N}_{\mathrm{3},\mathrm{5}} =\lfloor\frac{\mathrm{6000}}{\mathrm{3}×\mathrm{5}}\rfloor=\lfloor\frac{\mathrm{6000}}{\mathrm{15}}\rfloor=\mathrm{400} \\ $$$$\mathrm{N}_{\mathrm{2},\mathrm{3},\mathrm{5}} =\lfloor\frac{\mathrm{6000}}{\mathrm{2}×\mathrm{3}×\mathrm{5}}\rfloor=\lfloor\frac{\mathrm{6000}}{\mathrm{30}}\rfloor=\mathrm{200} \\ $$$$\mathrm{N}=\mathrm{3000}+\mathrm{2000}+\mathrm{1200}−\mathrm{1000}−\mathrm{600}−\mathrm{400}+\mathrm{200} \\ $$$$\mathrm{N}=\mathrm{4400} \\ $$$$\mathrm{so},\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{no}\:\mathrm{multiple}\:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{total}\:\mathrm{minus}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{multiples} \\ $$$$\mathrm{M}=\mathrm{6000}−\mathrm{4400}=\mathrm{1600} \\ $$
Commented by Yozzi last updated on 18/Nov/15
$${Thanks}.\:{I}\:{got}\:{that}. \\ $$
