Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 138823 by mnjuly1970 last updated on 18/Apr/21

......Nice ... Calculus...... Evaluate:: š›—=Ī£_(n=1) ^āˆž (1/)=?

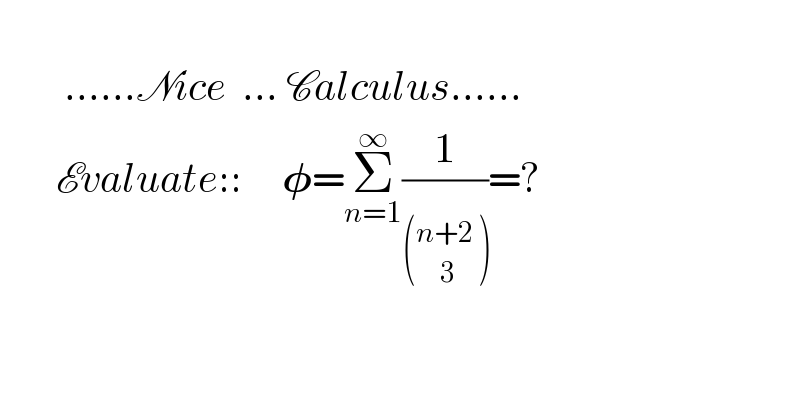
$$\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:......\mathscr{N}{ice}\:\:...\:\mathscr{C}{alculus}...... \\ $$$$\:\:\:\:\:\:\:\mathscr{E}{valuate}::\:\:\:\:\:\boldsymbol{\phi}=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{}=? \\ $$

Answered by qaz last updated on 18/Apr/21

Ļ†=Ī£_(n=1) ^āˆž (1/ (((n+2)),(3) ))=Ī£_(n=1) ^āˆž (6/((n+2)(n+1)n)) =6āˆ«_0 ^1 dxāˆ«_0 ^x dxāˆ«_0 ^x Ī£_(n=1) ^āˆž x^(nāˆ’1) dx =6āˆ«_0 ^1 dxāˆ«_0 ^x dxāˆ«_0 ^x (dx/(1āˆ’x)) =āˆ’6āˆ«_0 ^1 dxāˆ«_0 ^x ln(1āˆ’x)dx =6āˆ«_0 ^1 xlnx+1āˆ’xdx =(9/2)

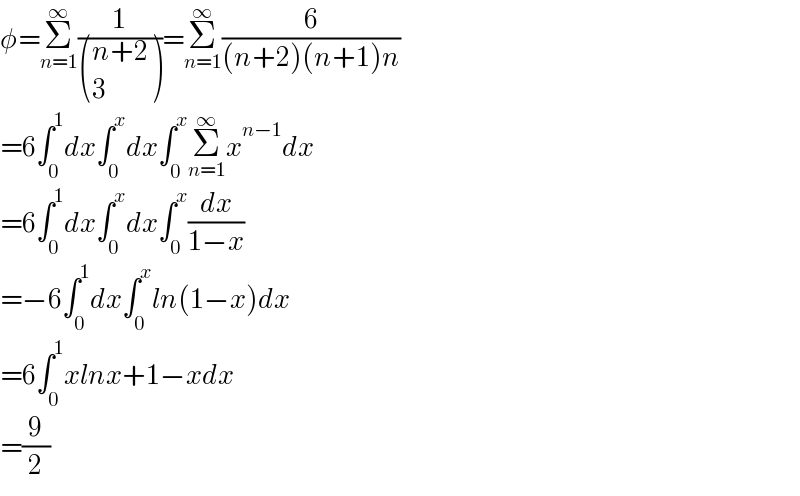
$$\phi=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\begin{pmatrix}{{n}+\mathrm{2}}\\{\mathrm{3}}\end{pmatrix}}=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{6}}{\left({n}+\mathrm{2}\right)\left({n}+\mathrm{1}\right){n}} \\ $$$$=\mathrm{6}\int_{\mathrm{0}} ^{\mathrm{1}} {dx}\int_{\mathrm{0}} ^{{x}} {dx}\int_{\mathrm{0}} ^{{x}} \underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{x}^{{n}āˆ’\mathrm{1}} {dx} \\ $$$$=\mathrm{6}\int_{\mathrm{0}} ^{\mathrm{1}} {dx}\int_{\mathrm{0}} ^{{x}} {dx}\int_{\mathrm{0}} ^{{x}} \frac{{dx}}{\mathrm{1}āˆ’{x}} \\ $$$$=āˆ’\mathrm{6}\int_{\mathrm{0}} ^{\mathrm{1}} {dx}\int_{\mathrm{0}} ^{{x}} {ln}\left(\mathrm{1}āˆ’{x}\right){dx} \\ $$$$=\mathrm{6}\int_{\mathrm{0}} ^{\mathrm{1}} {xlnx}+\mathrm{1}āˆ’{xdx} \\ $$$$=\frac{\mathrm{9}}{\mathrm{2}} \\ $$

Commented by mnjuly1970 last updated on 18/Apr/21

grateful...

$${grateful}... \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com