Question and Answers Forum

All Questions      Topic List

Vector Questions

Previous in All Question      Next in All Question      

Previous in Vector      Next in Vector      

Question Number 140176 by EDWIN88 last updated on 05/May/21

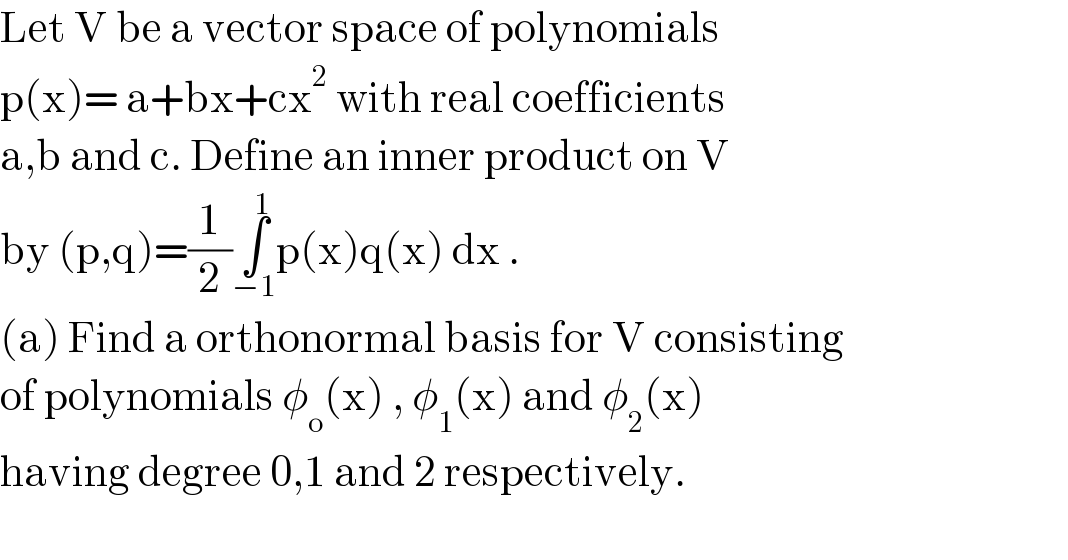
Let V be a vector space of polynomials p(x)= a+bx+cx^2 with real coefficients a,b and c. Define an inner product on V by (p,q)=(1/2)∫_(−1) ^1 p(x)q(x) dx . (a) Find a orthonormal basis for V consisting of polynomials φ_o (x) , φ_1 (x) and φ_2 (x) having degree 0,1 and 2 respectively.

$$\mathrm{Let}\:\mathrm{V}\:\mathrm{be}\:\mathrm{a}\:\mathrm{vector}\:\mathrm{space}\:\mathrm{of}\:\mathrm{polynomials} \\ $$$$\mathrm{p}\left(\mathrm{x}\right)=\:\mathrm{a}+\mathrm{bx}+\mathrm{cx}^{\mathrm{2}} \:\mathrm{with}\:\mathrm{real}\:\mathrm{coefficients} \\ $$$$\mathrm{a},\mathrm{b}\:\mathrm{and}\:\mathrm{c}.\:\mathrm{Define}\:\mathrm{an}\:\mathrm{inner}\:\mathrm{product}\:\mathrm{on}\:\mathrm{V} \\ $$$$\mathrm{by}\:\left(\mathrm{p},\mathrm{q}\right)=\frac{\mathrm{1}}{\mathrm{2}}\underset{−\mathrm{1}} {\overset{\mathrm{1}} {\int}}\mathrm{p}\left(\mathrm{x}\right)\mathrm{q}\left(\mathrm{x}\right)\:\mathrm{dx}\:. \\ $$$$\left(\mathrm{a}\right)\:\mathrm{Find}\:\mathrm{a}\:\mathrm{orthonormal}\:\mathrm{basis}\:\mathrm{for}\:\mathrm{V}\:\mathrm{consisting} \\ $$$$\mathrm{of}\:\mathrm{polynomials}\:\phi_{\mathrm{o}} \left(\mathrm{x}\right)\:,\:\phi_{\mathrm{1}} \left(\mathrm{x}\right)\:\mathrm{and}\:\phi_{\mathrm{2}} \left(\mathrm{x}\right) \\ $$$$\mathrm{having}\:\mathrm{degree}\:\mathrm{0},\mathrm{1}\:\mathrm{and}\:\mathrm{2}\:\mathrm{respectively}. \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com