
Question Number 44826 by Joel578 last updated on 05/Oct/18
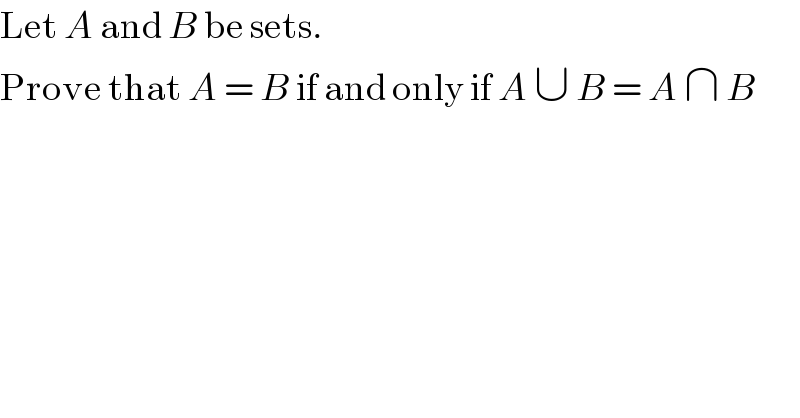
$$\mathrm{Let}\:{A}\:\mathrm{and}\:{B}\:\mathrm{be}\:\mathrm{sets}. \\ $$$$\mathrm{Prove}\:\mathrm{that}\:{A}\:=\:{B}\:\mathrm{if}\:\mathrm{and}\:\mathrm{only}\:\mathrm{if}\:{A}\:\cup\:{B}\:=\:{A}\:\cap\:{B} \\ $$
Answered by Kunal12588 last updated on 05/Oct/18

$${A}\:\cup{B}={A}\cap{B}\:......\left(\mathrm{1}\right) \\ $$$${let}\:\:{x}\in{A} \\ $$$$\Rightarrow\:{x}\in{A}\cup{B} \\ $$$$\Rightarrow{x}\in{A}\cap{B}\:\:\:{from}\:\left(\mathrm{1}\right) \\ $$$$\Rightarrow{x}\in{A}\:{and}\:{x}\in{B} \\ $$$${every}\:\:{element}\:{of}\:{A}\:{is}\:{also}\:{an}\:{element}\:{of}\:{B} \\ $$$$\Rightarrow{A}\subset{B} \\ $$$${let}\:{y}\in{B} \\ $$$$\Rightarrow{y}\in{A}\:{and}\:{y}\in{B} \\ $$$$\Rightarrow{B}\subset{A} \\ $$$${A}={B} \\ $$
Commented by Kunal12588 last updated on 05/Oct/18
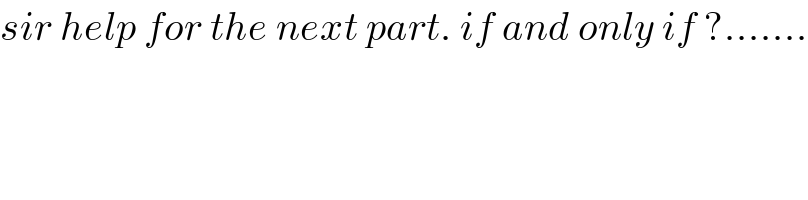
$${sir}\:{help}\:{for}\:{the}\:{next}\:{part}.\:{if}\:{and}\:{only}\:{if}\:?....... \\ $$
Answered by arcana last updated on 06/Oct/18

$$\mathrm{si}\:\mathrm{A}=\mathrm{B} \\ $$$$\mathrm{A}=\mathrm{A}\cup\mathrm{A}=\mathrm{A}\cup\mathrm{B}\:\vee\:\mathrm{B}=\mathrm{B}\cup\mathrm{B}=\mathrm{A}\cup\mathrm{B} \\ $$$$ \\ $$$$\Rightarrow\:\mathrm{A}\cap\mathrm{B}=\left(\mathrm{A}\cup\mathrm{B}\right)\cap\left(\mathrm{A}\cup\mathrm{B}\right)=\mathrm{A}\cup\mathrm{B} \\ $$
