Question and Answers Forum

All Questions      Topic List

Arithmetic Questions

Previous in All Question      Next in All Question      

Previous in Arithmetic      Next in Arithmetic      

Question Number 188970 by BaliramKumar last updated on 09/Mar/23

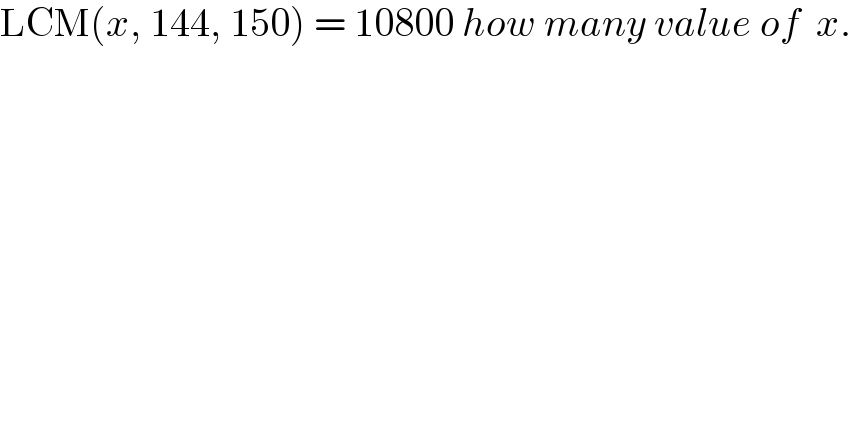
LCM(x, 144, 150) = 10800 how many value of x.

$$\mathrm{LCM}\left({x},\:\mathrm{144},\:\mathrm{150}\right)\:=\:\mathrm{10800}\:{how}\:{many}\:{value}\:{of}\:\:{x}. \\ $$$$ \\ $$

Answered by mr W last updated on 10/Mar/23

10800=2^4 ×3^3 ×5^2 144=2^4 ×3^2 150=2×3×5^2 x=2^(0−4) ×3^3 ×5^(0−2) ⇒there are 5×3=15 possible values for x.

$$\mathrm{10800}=\mathrm{2}^{\mathrm{4}} ×\mathrm{3}^{\mathrm{3}} ×\mathrm{5}^{\mathrm{2}} \\ $$$$\mathrm{144}=\mathrm{2}^{\mathrm{4}} ×\mathrm{3}^{\mathrm{2}} \\ $$$$\mathrm{150}=\mathrm{2}×\mathrm{3}×\mathrm{5}^{\mathrm{2}} \\ $$$${x}=\mathrm{2}^{\mathrm{0}−\mathrm{4}} ×\mathrm{3}^{\mathrm{3}} ×\mathrm{5}^{\mathrm{0}−\mathrm{2}} \\ $$$$\Rightarrow{there}\:{are}\:\mathrm{5}×\mathrm{3}=\mathrm{15}\:{possible}\:{values} \\ $$$${for}\:{x}. \\ $$

Commented by BaliramKumar last updated on 10/Mar/23

Thanks

$${Thanks} \\ $$

Commented by BaliramKumar last updated on 10/Mar/23

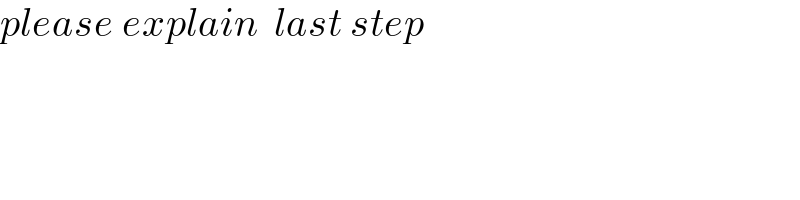
please explain last step

$${please}\:{explain}\:\:{last}\:{step} \\ $$

Commented by mr W last updated on 10/Mar/23

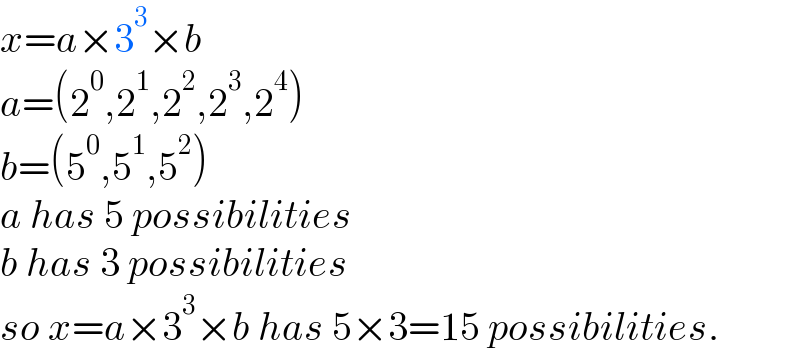
x=a×3^3 ×b a=(2^0 ,2^1 ,2^2 ,2^3 ,2^4 ) b=(5^0 ,5^1 ,5^2 ) a has 5 possibilities b has 3 possibilities so x=a×3^3 ×b has 5×3=15 possibilities.

$${x}={a}×\mathrm{3}^{\mathrm{3}} ×{b} \\ $$$${a}=\left(\mathrm{2}^{\mathrm{0}} ,\mathrm{2}^{\mathrm{1}} ,\mathrm{2}^{\mathrm{2}} ,\mathrm{2}^{\mathrm{3}} ,\mathrm{2}^{\mathrm{4}} \right) \\ $$$${b}=\left(\mathrm{5}^{\mathrm{0}} ,\mathrm{5}^{\mathrm{1}} ,\mathrm{5}^{\mathrm{2}} \right) \\ $$$${a}\:{has}\:\mathrm{5}\:{possibilities} \\ $$$${b}\:{has}\:\mathrm{3}\:{possibilities} \\ $$$${so}\:{x}={a}×\mathrm{3}^{\mathrm{3}} ×{b}\:{has}\:\mathrm{5}×\mathrm{3}=\mathrm{15}\:{possibilities}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com