Question and Answers Forum

IntegrationQuestion and Answers: Page 79

Question Number 147310 Answers: 1 Comments: 1

Question Number 147309 Answers: 2 Comments: 0

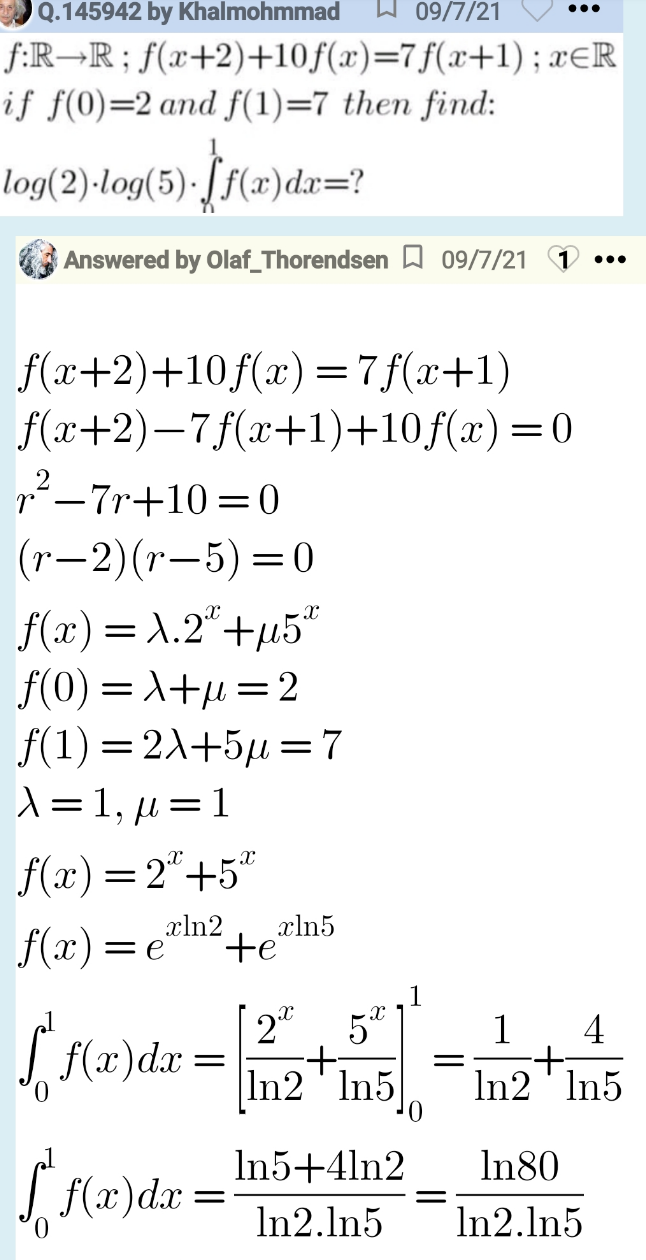
Question Number 147287 Answers: 2 Comments: 0

...Advanced Calculus... Calculate :: { (( i :: I := ∫_0 ^( 1) ln(x).ln(1+x) dx)),(( ii :: J := ∫_0 ^( 1) Li_( 2) ( 1− x^( 2) ) =?)) :} Note:: Li_2 (x) = Σ_(n=1) ^( ∞) (x^( n) /n^( 2) ) ........ ■ .... m.n....

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:...\mathrm{Advanced}\:\:\mathrm{Calculus}... \\ $$$$ \\ $$$$\:\:\:\:\:\:\mathrm{C}{alculate}\:::\:\:\:\:\begin{cases}{\:\:\mathrm{i}\:::\:\:\:\:\:\:\mathrm{I}\::=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \mathrm{ln}\left(\mathrm{x}\right).\mathrm{ln}\left(\mathrm{1}+\mathrm{x}\right)\:\mathrm{dx}}\\{\:\:\mathrm{ii}\:::\:\:\:\:\:\mathrm{J}\::=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \mathrm{Li}_{\:\mathrm{2}} \left(\:\mathrm{1}−\:\mathrm{x}^{\:\mathrm{2}} \right)\:=?}\end{cases} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{Note}::\:\:\:\mathrm{Li}_{\mathrm{2}} \:\left(\mathrm{x}\right)\:=\:\underset{{n}=\mathrm{1}} {\overset{\:\infty} {\sum}}\:\frac{\mathrm{x}^{\:{n}} }{{n}^{\:\mathrm{2}} }\:\:\:\:........\:\blacksquare\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:....\:\mathrm{m}.\mathrm{n}.... \\ $$$$ \\ $$

Question Number 147275 Answers: 1 Comments: 0

f(x)=∫_0 ^x e^(t−(t^2 /2)) dt show that ∫_0 ^1 f(t)dt=(√e)−1

$${f}\left({x}\right)=\int_{\mathrm{0}} ^{{x}} {e}^{{t}−\frac{{t}^{\mathrm{2}} }{\mathrm{2}}} {dt}\: \\ $$$${show}\:{that}\:\int_{\mathrm{0}} ^{\mathrm{1}} {f}\left({t}\right){dt}=\sqrt{{e}}−\mathrm{1} \\ $$

Question Number 147206 Answers: 0 Comments: 0

calculste ∫_0 ^1 (√(1+x^4 ))dx

$$\mathrm{calculste}\:\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\mathrm{dx} \\ $$

Question Number 147203 Answers: 1 Comments: 0

find U_n =∫_0 ^∞ (e^(−nx^2 ) /(x^2 +n^2 ))dx (n≥1) nature of ΣU_n and Σ nU_n

$$\mathrm{find}\:\mathrm{U}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{−\mathrm{nx}^{\mathrm{2}} } }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{n}^{\mathrm{2}} }\mathrm{dx}\:\:\:\:\:\left(\mathrm{n}\geqslant\mathrm{1}\right) \\ $$$$\mathrm{nature}\:\mathrm{of}\:\Sigma\mathrm{U}_{\mathrm{n}} \:\mathrm{and}\:\Sigma\:\mathrm{nU}_{\mathrm{n}} \\ $$

Question Number 147202 Answers: 0 Comments: 0

find ∫_0 ^1 ((√x)/( (√(x^2 +3))+(√(2x^2 +1))))dx

$$\mathrm{find}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\sqrt{\mathrm{x}}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{3}}+\sqrt{\mathrm{2x}^{\mathrm{2}} +\mathrm{1}}}\mathrm{dx} \\ $$

Question Number 147166 Answers: 2 Comments: 0

∫_R e^(ixt) e^(−t^2 ) dt..

$$\int_{\mathbb{R}} \mathrm{e}^{\mathrm{ixt}} \mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{dt}.. \\ $$

Question Number 147163 Answers: 0 Comments: 0

Question Number 147101 Answers: 1 Comments: 0

find U_n =∫_0 ^1 (1+x^2 )(1+x^4 )....(1+x^2^n )dx

$$\mathrm{find}\:\mathrm{U}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{x}^{\mathrm{4}} \right)....\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}^{\mathrm{n}} } \right)\mathrm{dx} \\ $$

Question Number 147061 Answers: 2 Comments: 0

∫_( 0 ) ^( ∞) (x^a /((1+x^3 ))) (dx/x) =? 0<a<3

$$\:\:\:\:\:\int_{\:\mathrm{0}\:} ^{\:\infty} \:\frac{{x}^{{a}} }{\left(\mathrm{1}+{x}^{\mathrm{3}} \right)}\:\frac{{dx}}{{x}}\:=?\: \\ $$$$\:\:\mathrm{0}<{a}<\mathrm{3}\:\: \\ $$

Question Number 147060 Answers: 1 Comments: 0

∫_0 ^(π/2) e^(2x) (√(tanx))dx

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {e}^{\mathrm{2}{x}} \sqrt{{tanx}}{dx} \\ $$

Question Number 147035 Answers: 2 Comments: 0

using residue theorem evaluate ∫_(∣z∣=3) ((zsecz)/((z−1)^2 ))dz

$${using}\:{residue}\:{theorem} \\ $$$${evaluate}\:\:\int_{\mid{z}\mid=\mathrm{3}} \frac{{zsecz}}{\left({z}−\mathrm{1}\right)^{\mathrm{2}} }{dz} \\ $$

Question Number 146996 Answers: 1 Comments: 0

∫ln(cht)dt

$$\int{ln}\left({cht}\right){dt} \\ $$

Question Number 146962 Answers: 1 Comments: 0

Question Number 146933 Answers: 0 Comments: 0

.....# advanced calculu#...... I := ∫_(−∞) ^( +∞) sin(cosh(x).cos(sinhx))dx=? ....solution .... I:=(1/2) ∫_(−∞) ^( +∞) {sin (cosh(x)+sinh(x))+sin(cosh(x)−sinh (x))} :=_(sinh(x)=((e^( x) −e^( −x) )/2)) ^(cosh(x)=((e^( x) +e^( −x) )/2)) (1/2) ∫_(−∞) ^( +∞) {sin(e^x )+sin (e^( −x) )}dx := (1/2) ∫_(−∞) ^( ∞) sin(e^( x) )dx +[(1/2)∫_(−∞) ^( +∞) sin(e^( x) )dx :: x=^(sub) −x] := ∫_(−∞) ^( +∞) sin(e^( x) ) dx =^(e^( x) =y) ∫_0 ^( ∞) ((sin(t))/t) dt ...... I:= (π/2) ..... ...m.n.1970...

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:.....#\:{advanced}\:\:{calculu}#...... \\ $$$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{I}\::=\:\int_{−\infty} ^{\:+\infty} {sin}\left({cosh}\left({x}\right).{cos}\left({sinhx}\right)\right){dx}=? \\ $$$$\:\:\:\:\:....{solution}\:.... \\ $$$$\:\:\:\:\:\:\:\mathrm{I}:=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{−\infty} ^{\:+\infty} \left\{{sin}\:\left({cosh}\left({x}\right)+{sinh}\left({x}\right)\right)+{sin}\left({cosh}\left({x}\right)−{sinh}\:\left({x}\right)\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\::\underset{{sinh}\left({x}\right)=\frac{{e}^{\:{x}} −{e}^{\:−{x}} }{\mathrm{2}}} {\overset{{cosh}\left({x}\right)=\frac{{e}^{\:{x}} +{e}^{\:−{x}} }{\mathrm{2}}} {=}}\:\:\frac{\mathrm{1}}{\mathrm{2}}\:\int_{−\infty} ^{\:+\infty} \left\{{sin}\left({e}^{{x}} \right)+{sin}\:\left({e}^{\:−{x}} \right)\right\}{dx} \\ $$$$\:\:\:\:\:\:\:\:\::=\:\:\frac{\mathrm{1}}{\mathrm{2}}\:\int_{−\infty} ^{\:\infty} {sin}\left({e}^{\:{x}} \right){dx}\:+\left[\frac{\mathrm{1}}{\mathrm{2}}\int_{−\infty} ^{\:+\infty} {sin}\left({e}^{\:{x}} \right){dx}\:::\:\:{x}\overset{{sub}} {=}−{x}\right] \\ $$$$\:\:\:\:\:\:\:\::=\:\int_{−\infty} ^{\:+\infty} {sin}\left({e}^{\:{x}} \:\right)\:{dx}\:\overset{{e}^{\:{x}} ={y}} {=}\:\int_{\mathrm{0}} ^{\:\infty} \frac{{sin}\left({t}\right)}{{t}}\:{dt}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:......\:\mathrm{I}:=\:\frac{\pi}{\mathrm{2}}\:..... \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...{m}.{n}.\mathrm{1970}... \\ $$$$\: \\ $$$$ \\ $$

Question Number 146836 Answers: 0 Comments: 0

Σ_(n=1) ^∞ (1/(ϕ^( n) F_n )) =?

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\varphi^{\:{n}} \:\mathrm{F}_{{n}} }\:=? \\ $$

Question Number 146835 Answers: 2 Comments: 0

calculate ∫_0 ^∞ (dx/((x^2 +3)^2 (x^2 +4)^2 ))

$$\mathrm{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{dx}}{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{3}\right)^{\mathrm{2}} \left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{4}\right)^{\mathrm{2}} } \\ $$

Question Number 146791 Answers: 1 Comments: 0

∫(x/(1+cos^2 (x)))dx

$$\int\frac{\mathrm{x}}{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \left(\mathrm{x}\right)}\mathrm{dx} \\ $$

Question Number 146790 Answers: 1 Comments: 0

∫(1/x) e^(−(1/x^2 )) dx