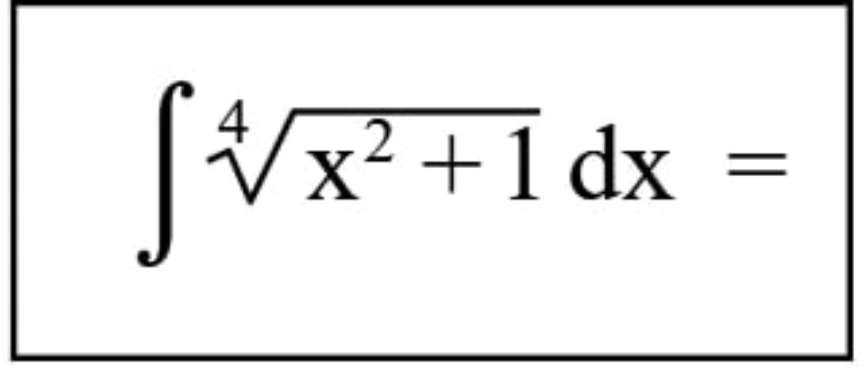Question and Answers Forum

IntegrationQuestion and Answers: Page 77

Question Number 147799 Answers: 1 Comments: 0

∫(((√(2−x^2 ))+(√(2+x^2 )))/( (√(4−x^2 ))))dx

$$\int\frac{\sqrt{\mathrm{2}−{x}^{\mathrm{2}} }+\sqrt{\mathrm{2}+{x}^{\mathrm{2}} }}{\:\sqrt{\mathrm{4}−{x}^{\mathrm{2}} }}{dx} \\ $$

Question Number 147749 Answers: 1 Comments: 0

∫(((√(2−x^2 ))+(√(2+x^2 )))/( (√(4−x^4 ))))dx

$$\int\frac{\sqrt{\mathrm{2}−{x}^{\mathrm{2}} }+\sqrt{\mathrm{2}+{x}^{\mathrm{2}} }}{\:\sqrt{\mathrm{4}−{x}^{\mathrm{4}} }}{dx} \\ $$

Question Number 147747 Answers: 0 Comments: 0

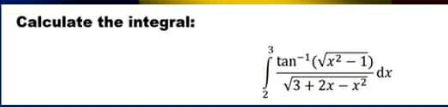
Question Number 147746 Answers: 1 Comments: 0

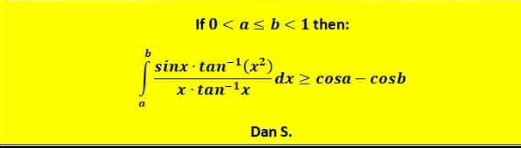
Question Number 147683 Answers: 1 Comments: 0

let F(x)=(1/((x+1)^5 (2x−3)^4 )) 1) find ∫ F(x)dx 2)en deduire la decomposition de F en element simples

$$\mathrm{let}\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\left(\mathrm{x}+\mathrm{1}\right)^{\mathrm{5}} \left(\mathrm{2x}−\mathrm{3}\right)^{\mathrm{4}} } \\ $$$$\left.\mathrm{1}\right)\:\mathrm{find}\:\int\:\mathrm{F}\left(\mathrm{x}\right)\mathrm{dx} \\ $$$$\left.\mathrm{2}\right)\mathrm{en}\:\mathrm{deduire}\:\mathrm{la}\:\mathrm{decomposition}\:\mathrm{de}\:\mathrm{F}\:\mathrm{en}\:\mathrm{element}\:\mathrm{simples} \\ $$

Question Number 147682 Answers: 0 Comments: 0

decompose F(x)=(1/((x^n −1)(x^2 +x+1))) dans C(x) puis dans R(x)

$$\mathrm{decompose}\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\left(\mathrm{x}^{\mathrm{n}} −\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{x}+\mathrm{1}\right)}\:\mathrm{dans}\:\mathrm{C}\left(\mathrm{x}\right)\:\mathrm{puis}\:\mathrm{dans}\:\mathrm{R}\left(\mathrm{x}\right) \\ $$

Question Number 147680 Answers: 0 Comments: 2

find by residus ∫_0 ^∞ ((cos(2x))/((x^2 −x+1)^3 ))dx

$$\mathrm{find}\:\mathrm{by}\:\mathrm{residus}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}} }\mathrm{dx} \\ $$

Question Number 147670 Answers: 1 Comments: 0

Question Number 147576 Answers: 1 Comments: 0

(1):: Σ_(i=1) ^n Σ_(j=1) ^n ∣i−j∣=? (2):: Σ_(i=1) ^n Σ_(j=i) ^n (1/j)=? (3):: Σ_(i=1) ^n^2 [(√i)]=?

$$\left(\mathrm{1}\right)::\:\:\:\:\:\underset{\mathrm{i}=\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\underset{\mathrm{j}=\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\mid\mathrm{i}−\mathrm{j}\mid=? \\ $$$$\left(\mathrm{2}\right)::\:\:\:\:\:\underset{\mathrm{i}=\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\underset{\mathrm{j}=\mathrm{i}} {\overset{\mathrm{n}} {\sum}}\frac{\mathrm{1}}{\mathrm{j}}=? \\ $$$$\left(\mathrm{3}\right)::\:\:\:\:\:\:\underset{\mathrm{i}=\mathrm{1}} {\overset{\mathrm{n}^{\mathrm{2}} } {\sum}}\left[\sqrt{\mathrm{i}}\right]=? \\ $$

Question Number 147487 Answers: 1 Comments: 0

(a , 2a +1 ]∩[ a^( 2) −a , a^( 2) + 4a +1 )≠ ∅ a ∈ ?

$$ \\ $$$$ \\ $$$$\left({a}\:,\:\mathrm{2}{a}\:+\mathrm{1}\:\right]\cap\left[\:{a}^{\:\mathrm{2}} \:−{a}\:,\:{a}^{\:\mathrm{2}} +\:\mathrm{4}{a}\:+\mathrm{1}\:\right)\neq\:\varnothing \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{a}\:\in\:? \\ $$$$ \\ $$

Question Number 147477 Answers: 1 Comments: 1

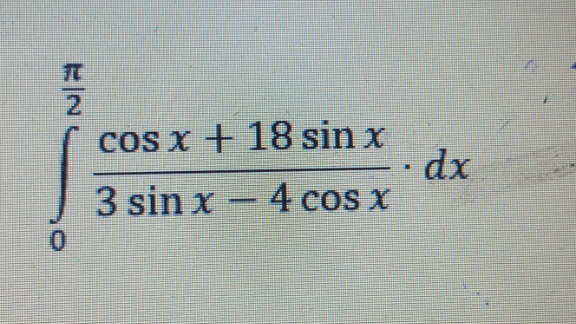
Question Number 147399 Answers: 0 Comments: 0

∫_0 ^(π/2) (e^(2arctg(u)) /( (√u)))

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{e}^{\mathrm{2}{arctg}\left({u}\right)} }{\:\sqrt{{u}}} \\ $$

Question Number 147310 Answers: 1 Comments: 1

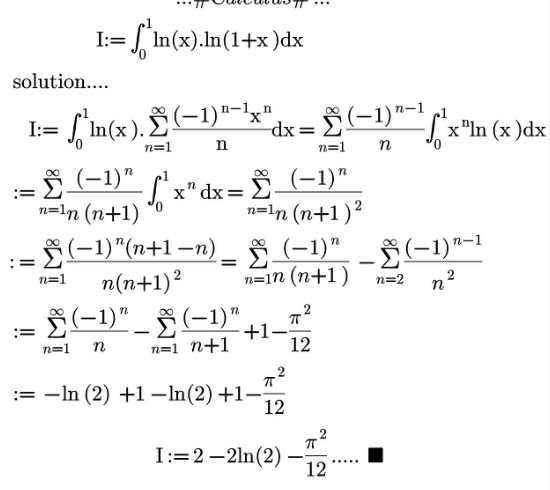
Question Number 147309 Answers: 2 Comments: 0

Question Number 147287 Answers: 2 Comments: 0

...Advanced Calculus... Calculate :: { (( i :: I := ∫_0 ^( 1) ln(x).ln(1+x) dx)),(( ii :: J := ∫_0 ^( 1) Li_( 2) ( 1− x^( 2) ) =?)) :} Note:: Li_2 (x) = Σ_(n=1) ^( ∞) (x^( n) /n^( 2) ) ........ ■ .... m.n....

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:...\mathrm{Advanced}\:\:\mathrm{Calculus}... \\ $$$$ \\ $$$$\:\:\:\:\:\:\mathrm{C}{alculate}\:::\:\:\:\:\begin{cases}{\:\:\mathrm{i}\:::\:\:\:\:\:\:\mathrm{I}\::=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \mathrm{ln}\left(\mathrm{x}\right).\mathrm{ln}\left(\mathrm{1}+\mathrm{x}\right)\:\mathrm{dx}}\\{\:\:\mathrm{ii}\:::\:\:\:\:\:\mathrm{J}\::=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \mathrm{Li}_{\:\mathrm{2}} \left(\:\mathrm{1}−\:\mathrm{x}^{\:\mathrm{2}} \right)\:=?}\end{cases} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{Note}::\:\:\:\mathrm{Li}_{\mathrm{2}} \:\left(\mathrm{x}\right)\:=\:\underset{{n}=\mathrm{1}} {\overset{\:\infty} {\sum}}\:\frac{\mathrm{x}^{\:{n}} }{{n}^{\:\mathrm{2}} }\:\:\:\:........\:\blacksquare\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:....\:\mathrm{m}.\mathrm{n}.... \\ $$$$ \\ $$

Question Number 147275 Answers: 1 Comments: 0

f(x)=∫_0 ^x e^(t−(t^2 /2)) dt show that ∫_0 ^1 f(t)dt=(√e)−1

$${f}\left({x}\right)=\int_{\mathrm{0}} ^{{x}} {e}^{{t}−\frac{{t}^{\mathrm{2}} }{\mathrm{2}}} {dt}\: \\ $$$${show}\:{that}\:\int_{\mathrm{0}} ^{\mathrm{1}} {f}\left({t}\right){dt}=\sqrt{{e}}−\mathrm{1} \\ $$

Question Number 147206 Answers: 0 Comments: 0

calculste ∫_0 ^1 (√(1+x^4 ))dx

$$\mathrm{calculste}\:\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\mathrm{dx} \\ $$

Question Number 147203 Answers: 1 Comments: 0

find U_n =∫_0 ^∞ (e^(−nx^2 ) /(x^2 +n^2 ))dx (n≥1) nature of ΣU_n and Σ nU_n

$$\mathrm{find}\:\mathrm{U}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{−\mathrm{nx}^{\mathrm{2}} } }{\mathrm{x}^{\mathrm{2}} \:+\mathrm{n}^{\mathrm{2}} }\mathrm{dx}\:\:\:\:\:\left(\mathrm{n}\geqslant\mathrm{1}\right) \\ $$$$\mathrm{nature}\:\mathrm{of}\:\Sigma\mathrm{U}_{\mathrm{n}} \:\mathrm{and}\:\Sigma\:\mathrm{nU}_{\mathrm{n}} \\ $$

Question Number 147202 Answers: 0 Comments: 0

find ∫_0 ^1 ((√x)/( (√(x^2 +3))+(√(2x^2 +1))))dx

$$\mathrm{find}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\sqrt{\mathrm{x}}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{3}}+\sqrt{\mathrm{2x}^{\mathrm{2}} +\mathrm{1}}}\mathrm{dx} \\ $$

Question Number 147166 Answers: 2 Comments: 0

∫_R e^(ixt) e^(−t^2 ) dt..

$$\int_{\mathbb{R}} \mathrm{e}^{\mathrm{ixt}} \mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{dt}.. \\ $$

Question Number 147163 Answers: 0 Comments: 0

Question Number 147101 Answers: 1 Comments: 0

find U_n =∫_0 ^1 (1+x^2 )(1+x^4 )....(1+x^2^n )dx

$$\mathrm{find}\:\mathrm{U}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{x}^{\mathrm{4}} \right)....\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}^{\mathrm{n}} } \right)\mathrm{dx} \\ $$

Question Number 147061 Answers: 2 Comments: 0

∫_( 0 ) ^( ∞) (x^a /((1+x^3 ))) (dx/x) =? 0<a<3

$$\:\:\:\:\:\int_{\:\mathrm{0}\:} ^{\:\infty} \:\frac{{x}^{{a}} }{\left(\mathrm{1}+{x}^{\mathrm{3}} \right)}\:\frac{{dx}}{{x}}\:=?\: \\ $$$$\:\:\mathrm{0}<{a}<\mathrm{3}\:\: \\ $$

Question Number 147060 Answers: 1 Comments: 0

∫_0 ^(π/2) e^(2x) (√(tanx))dx

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {e}^{\mathrm{2}{x}} \sqrt{{tanx}}{dx} \\ $$

Question Number 147035 Answers: 2 Comments: 0

using residue theorem evaluate ∫_(∣z∣=3) ((zsecz)/((z−1)^2 ))dz

$${using}\:{residue}\:{theorem} \\ $$$${evaluate}\:\:\int_{\mid{z}\mid=\mathrm{3}} \frac{{zsecz}}{\left({z}−\mathrm{1}\right)^{\mathrm{2}} }{dz} \\ $$

Question Number 146996 Answers: 1 Comments: 0

∫ln(cht)dt

$$\int{ln}\left({cht}\right){dt} \\ $$

Pg 72 Pg 73 Pg 74 Pg 75 Pg 76 Pg 77 Pg 78 Pg 79 Pg 80 Pg 81

Contact: info@tinkutara.com