Question and Answers Forum

IntegrationQuestion and Answers: Page 51

Question Number 168368 Answers: 1 Comments: 0

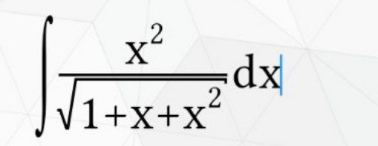
Question Number 168366 Answers: 1 Comments: 0

Question Number 168355 Answers: 1 Comments: 0

let U_n =∫_0 ^1 (x^n )(√(1−x^(2n+1) )))dx 1) find a equivalent of U_n (n∼∞) 2) study the comvergence of Σ U_n

$$\left.{let}\:{U}_{{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \left({x}^{{n}} \right)\sqrt{\mathrm{1}−{x}^{\mathrm{2}{n}+\mathrm{1}} }\right){dx} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{equivalent}\:{of}\:{U}_{{n}} \left({n}\sim\infty\right) \\ $$$$\left.\mathrm{2}\right)\:{study}\:{the}\:{comvergence}\:{of}\:\Sigma\:{U}_{{n}} \\ $$

Question Number 168341 Answers: 1 Comments: 0

∫(1+x^2 )^3 dx=?

$$\int\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{3}} {dx}=? \\ $$

Question Number 168338 Answers: 1 Comments: 0

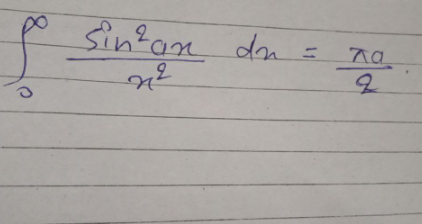
Question Number 168320 Answers: 0 Comments: 0

∫(((arcsin(x))^2 )/(1+x^2 ))dx=???

$$\:\:\:\int\frac{\left({arcsin}\left({x}\right)\right)^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=??? \\ $$

Question Number 168311 Answers: 0 Comments: 5

Calculate∫(((arcsin(x))^2 )/(1+x^2 ))dx

$${Calculate}\int\frac{\left({arcsin}\left({x}\right)\right)^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$

Question Number 168303 Answers: 2 Comments: 0

calculate ∫_0 ^1 x(√(1−x^6 ))dx

$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} {x}\sqrt{\mathrm{1}−{x}^{\mathrm{6}} }{dx} \\ $$

Question Number 168296 Answers: 2 Comments: 0

∫(5x+2)cos(2x)dx=?

$$\int\left(\mathrm{5}{x}+\mathrm{2}\right){cos}\left(\mathrm{2}{x}\right){dx}=? \\ $$

Question Number 168188 Answers: 4 Comments: 1

∫ ((1−sin 2x)/((1+sin 2x)^2 )) dx =?

$$\:\:\:\:\:\int\:\frac{\mathrm{1}−\mathrm{sin}\:\mathrm{2}{x}}{\left(\mathrm{1}+\mathrm{sin}\:\mathrm{2}{x}\right)^{\mathrm{2}} }\:{dx}\:=? \\ $$

Question Number 167989 Answers: 2 Comments: 0

∫ ((3−cos x)/(3+cos x)) dx =?

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\int\:\frac{\mathrm{3}−\mathrm{cos}\:{x}}{\mathrm{3}+\mathrm{cos}\:{x}}\:{dx}\:=? \\ $$

Question Number 167965 Answers: 1 Comments: 0

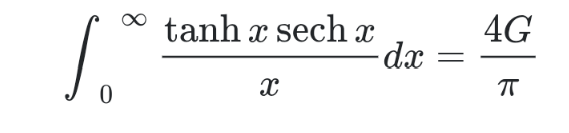
Question Number 167922 Answers: 4 Comments: 2

Find the value of Q if ∫_0 ^Q (√(cosec θ−1)) dθ=ln (((3+2(√2))/2))

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\:\mathrm{Q}\:\:\mathrm{if} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{Q}} \sqrt{\mathrm{cosec}\:\theta−\mathrm{1}}\:\mathrm{d}\theta=\mathrm{ln}\:\left(\frac{\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{2}}\right) \\ $$$$ \\ $$

Question Number 167912 Answers: 1 Comments: 0

∫^(π/4) _0 ((cos(12x))/(cos^(14) (x)))dx=?

$$\underset{\mathrm{0}} {\int}^{\frac{\pi}{\mathrm{4}}} \frac{{cos}\left(\mathrm{12}{x}\right)}{{cos}^{\mathrm{14}} \left({x}\right)}{dx}=? \\ $$

Question Number 167885 Answers: 0 Comments: 0

Question Number 167862 Answers: 1 Comments: 0

■Nice integral■ 𝛀=∫_0 ^1 ((ln^3 (1−x^2 ))/( (√(1−x^2 ))))dx ? by MATH.AMIN

$$\blacksquare\boldsymbol{\mathrm{Nice}}\:\boldsymbol{\mathrm{integral}}\blacksquare \\ $$$$\boldsymbol{\Omega}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\boldsymbol{\mathrm{ln}}^{\mathrm{3}} \left(\mathrm{1}−\boldsymbol{\mathrm{x}}^{\mathrm{2}} \right)}{\:\sqrt{\mathrm{1}−\boldsymbol{\mathrm{x}}^{\mathrm{2}} }}\boldsymbol{\mathrm{dx}}\:\:\:? \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{by}}\:\boldsymbol{\mathrm{MATH}}.\boldsymbol{\mathrm{AMIN}} \\ $$

Question Number 167823 Answers: 1 Comments: 2

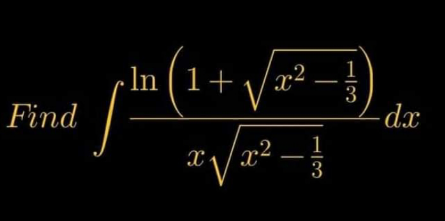
Question Number 167716 Answers: 1 Comments: 0

Question Number 167713 Answers: 1 Comments: 0

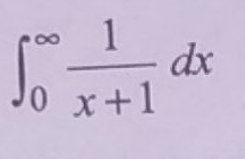
Question Number 167647 Answers: 2 Comments: 0

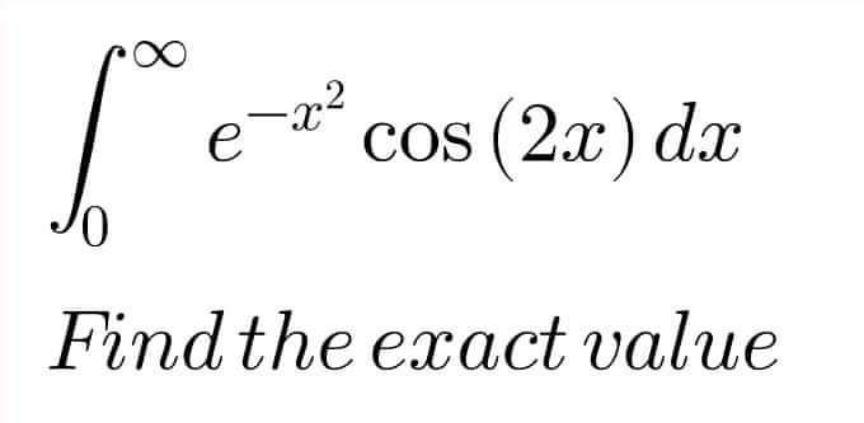
Question Number 167626 Answers: 0 Comments: 0

∫_0 ^∞ (e^(−t) /( (√t))) e^(−(1/(4t))) dt

$$\int_{\mathrm{0}} ^{\infty} \frac{{e}^{−{t}} }{\:\sqrt{{t}}}\:{e}^{−\frac{\mathrm{1}}{\mathrm{4}{t}}} \:{dt} \\ $$

Question Number 167598 Answers: 1 Comments: 0

∫ (x/(5x^4 +x^3 −5x−1)) dx=?

$$\:\:\:\:\:\:\int\:\frac{\mathrm{x}}{\mathrm{5x}^{\mathrm{4}} +\mathrm{x}^{\mathrm{3}} −\mathrm{5x}−\mathrm{1}}\:\mathrm{dx}=? \\ $$

Question Number 167596 Answers: 1 Comments: 0

∫ ((cos 7x−cos 8x)/(1+2cos 5x)) dx =?

$$\:\:\int\:\frac{\mathrm{cos}\:\mathrm{7x}−\mathrm{cos}\:\mathrm{8x}}{\mathrm{1}+\mathrm{2cos}\:\mathrm{5x}}\:\mathrm{dx}\:=? \\ $$

Question Number 167586 Answers: 2 Comments: 1

λ=∫ (dx/( (√(1+cos x))+(√(1+sin x)))) =?

$$\:\:\:\:\:\:\:\:\lambda=\int\:\frac{\mathrm{dx}}{\:\sqrt{\mathrm{1}+\mathrm{cos}\:\mathrm{x}}+\sqrt{\mathrm{1}+\mathrm{sin}\:\mathrm{x}}}\:=? \\ $$

Question Number 167562 Answers: 1 Comments: 0

∫ (e^(−x) /(1+e^x )) dx=?

$$\:\:\:\:\:\:\:\:\int\:\frac{{e}^{−{x}} }{\mathrm{1}+{e}^{{x}} }\:{dx}=? \\ $$

Question Number 167510 Answers: 1 Comments: 0

explicite f(a)=∫_0 ^(π/2) ln(a+tan^2 x)dx a≥2

$${explicite}\:{f}\left({a}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left({a}+{tan}^{\mathrm{2}} {x}\right){dx} \\ $$$${a}\geqslant\mathrm{2} \\ $$

Pg 46 Pg 47 Pg 48 Pg 49 Pg 50 Pg 51 Pg 52 Pg 53 Pg 54 Pg 55

Contact: info@tinkutara.com