
IntegrationQuestion and Answers: Page 301
Question Number 34662 Answers: 0 Comments: 0
Question Number 34661 Answers: 0 Comments: 0
Question Number 34635 Answers: 2 Comments: 4
Question Number 34633 Answers: 0 Comments: 0
Question Number 34593 Answers: 0 Comments: 0
Question Number 34562 Answers: 1 Comments: 1
Question Number 34561 Answers: 0 Comments: 1
Question Number 34985 Answers: 1 Comments: 0
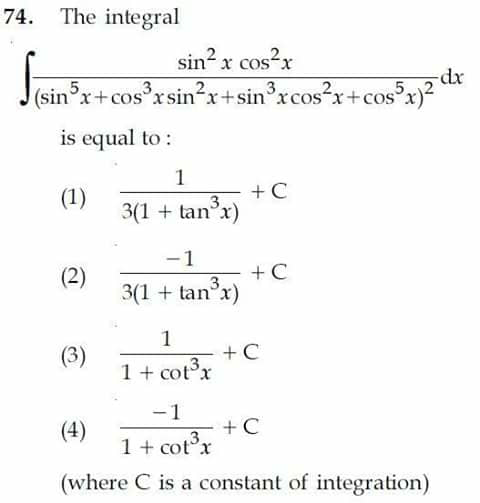
Question Number 34421 Answers: 0 Comments: 1
Question Number 34320 Answers: 0 Comments: 2
Question Number 34316 Answers: 0 Comments: 0
Question Number 34315 Answers: 0 Comments: 2
Question Number 34314 Answers: 0 Comments: 1
Question Number 34312 Answers: 0 Comments: 1
Question Number 34308 Answers: 0 Comments: 0
Question Number 34298 Answers: 0 Comments: 2
Question Number 34297 Answers: 1 Comments: 1
Question Number 34296 Answers: 0 Comments: 3
Question Number 34295 Answers: 0 Comments: 0
Question Number 34294 Answers: 0 Comments: 0
Question Number 34293 Answers: 0 Comments: 0
Question Number 34292 Answers: 0 Comments: 1
Question Number 34291 Answers: 0 Comments: 0
Question Number 34290 Answers: 0 Comments: 0
Question Number 34289 Answers: 0 Comments: 1
Question Number 34288 Answers: 0 Comments: 0
Pg 296 Pg 297 Pg 298 Pg 299 Pg 300 Pg 301 Pg 302 Pg 303 Pg 304 Pg 305
