Question and Answers Forum

IntegrationQuestion and Answers: Page 298

Question Number 35583 Answers: 0 Comments: 0

find ∫_0 ^(π/6) (√(x−sinx)) dx

$${find}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} \:\sqrt{{x}−{sinx}}\:{dx} \\ $$

Question Number 35582 Answers: 0 Comments: 0

let g(x)= (1/(2+sinx)) , 2π periodic odd developp f at fourier serie .

$${let}\:{g}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{2}+{sinx}}\:\:\:\:,\:\mathrm{2}\pi\:{periodic}\:{odd} \\ $$$${developp}\:{f}\:{at}\:{fourier}\:{serie}\:. \\ $$

Question Number 35581 Answers: 0 Comments: 0

let f(x) = (3/(1+2cosx)) , 2π periodic even developp f at fourier serie.

$${let}\:{f}\left({x}\right)\:=\:\:\frac{\mathrm{3}}{\mathrm{1}+\mathrm{2}{cosx}}\:\:,\:\mathrm{2}\pi\:{periodic}\:{even} \\ $$$${developp}\:{f}\:\:{at}\:{fourier}\:{serie}. \\ $$$$ \\ $$

Question Number 35580 Answers: 0 Comments: 0

if (1/(1+cosx)) = (a_0 /2) +Σ_(n=1) ^∞ a_n cos(nx) calculate a_0 and a_n

$${if}\:\:\:\frac{\mathrm{1}}{\mathrm{1}+{cosx}}\:=\:\frac{{a}_{\mathrm{0}} }{\mathrm{2}}\:+\sum_{{n}=\mathrm{1}} ^{\infty} \:{a}_{{n}} \:{cos}\left({nx}\right)\:\:{calculate}\:{a}_{\mathrm{0}} \\ $$$${and}\:{a}_{{n}} \\ $$

Question Number 35551 Answers: 0 Comments: 1

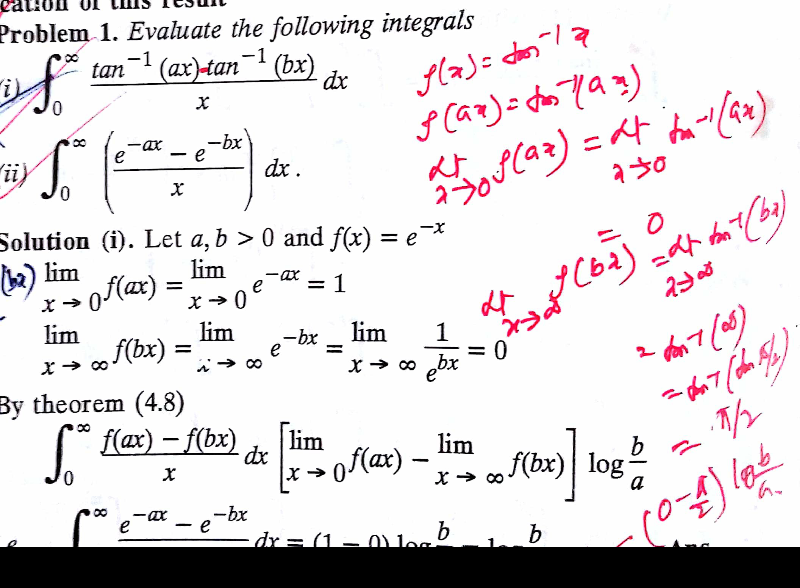
Question Number 35550 Answers: 0 Comments: 0

Question Number 35549 Answers: 0 Comments: 1

Question Number 35548 Answers: 0 Comments: 0

Question Number 35547 Answers: 0 Comments: 0

Question Number 35546 Answers: 0 Comments: 0

Question Number 35545 Answers: 0 Comments: 0

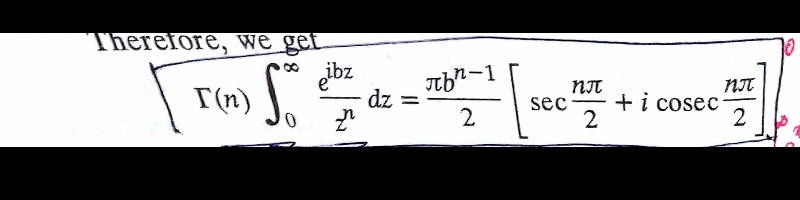
Question Number 35544 Answers: 0 Comments: 1

Question Number 35543 Answers: 0 Comments: 0

Question Number 35541 Answers: 0 Comments: 3

Question Number 35471 Answers: 0 Comments: 0

∫_a ^b f(x)dx=area under the curve but say why what is the meaning of ∫ ←this sign

$$\int_{{a}} ^{{b}} {f}\left({x}\right){dx}={area}\:{under}\:{the}\:{curve}\:{but}\:{say}\:{why} \\ $$$${what}\:{is}\:{the}\:{meaning}\:{of}\:\int\:\leftarrow{this}\:{sign} \\ $$

Question Number 35456 Answers: 2 Comments: 0

∫(dx/(x(x^(2018) +1)))

$$\int\frac{{dx}}{{x}\left({x}^{\mathrm{2018}} +\mathrm{1}\right)} \\ $$

Question Number 35440 Answers: 1 Comments: 2

find the value of ∫_0 ^∞ (dx/((2x^2 +1)^2 ))

$${find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\frac{{dx}}{\left(\mathrm{2}{x}^{\mathrm{2}} \:\:+\mathrm{1}\right)^{\mathrm{2}} } \\ $$

Question Number 35438 Answers: 0 Comments: 1

let m>0 and 0<a<b 1) calculate ∫_0 ^∞ ((cos(mx))/((x^2 +a^2 )(x^2 +b^2 )))dx 2)find the value of ∫_0 ^∞ ((cos(2x))/((x^2 +1)(x^2 +3)))dx

$${let}\:{m}>\mathrm{0}\:{and}\:\mathrm{0}<{a}<{b}\: \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\:\:\frac{{cos}\left({mx}\right)}{\left({x}^{\mathrm{2}} \:+{a}^{\mathrm{2}} \right)\left({x}^{\mathrm{2}} \:+{b}^{\mathrm{2}} \right)}{dx} \\ $$$$\left.\mathrm{2}\right){find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\:\frac{{cos}\left(\mathrm{2}{x}\right)}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}^{\mathrm{2}} \:\:+\mathrm{3}\right)}{dx} \\ $$

Question Number 35429 Answers: 1 Comments: 1

find ∫_0 ^1 ((2x−1)/(√(x^2 +6))) dx

$${find}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{\mathrm{2}{x}−\mathrm{1}}{\sqrt{{x}^{\mathrm{2}} \:\:+\mathrm{6}}}\:{dx} \\ $$

Question Number 35428 Answers: 1 Comments: 1

find ∫ ((x+3)/(√(x^2 +x −1)))dx

$${find}\:\:\:\:\:\int\:\:\:\:\frac{{x}+\mathrm{3}}{\sqrt{{x}^{\mathrm{2}} \:+{x}\:−\mathrm{1}}}{dx} \\ $$

Question Number 35427 Answers: 1 Comments: 1

calculate ∫_2 ^5 (e^(√(x+1)) /(√(x+1)))dx

$${calculate}\:\:\:\int_{\mathrm{2}} ^{\mathrm{5}} \:\:\:\frac{{e}^{\sqrt{{x}+\mathrm{1}}} }{\sqrt{{x}+\mathrm{1}}}{dx} \\ $$

Question Number 35379 Answers: 2 Comments: 3

∫_0 ^( 1) t^2 (√(1+t^2 )) dt = ?

$$\int_{\mathrm{0}} ^{\:\:\mathrm{1}} {t}^{\mathrm{2}} \sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt}\:=\:? \\ $$

Question Number 35325 Answers: 1 Comments: 0

Sketch the region enclosed by the curves of y=1/x and y=1/x^2 and find the area of the region. plzz help me

$${Sketch}\:{the}\:{region}\:{enclosed}\:{by}\:{the} \\ $$$${curves}\:{of}\:{y}=\mathrm{1}/{x}\:{and}\:{y}=\mathrm{1}/{x}^{\mathrm{2}} \:{and} \\ $$$${find}\:{the}\:{area}\:{of}\:{the}\:{region}. \\ $$$${plzz}\:{help}\:{me} \\ $$

Question Number 35294 Answers: 1 Comments: 1

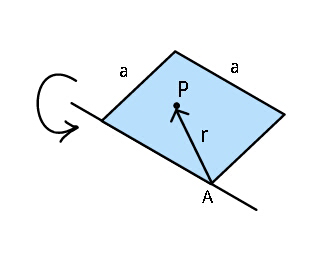
Question Number 35290 Answers: 0 Comments: 1

∫((x+1)/x^3 )dx

$$\int\frac{{x}+\mathrm{1}}{{x}^{\mathrm{3}} }{dx} \\ $$

Question Number 35242 Answers: 1 Comments: 1

find ∫_0 ^π ((xdx)/(1+sinx))

$${find}\:\:\int_{\mathrm{0}} ^{\pi} \:\:\:\:\frac{{xdx}}{\mathrm{1}+{sinx}} \\ $$

Pg 293 Pg 294 Pg 295 Pg 296 Pg 297 Pg 298 Pg 299 Pg 300 Pg 301 Pg 302

Contact: info@tinkutara.com