Question and Answers Forum

IntegrationQuestion and Answers: Page 296

Question Number 35982 Answers: 0 Comments: 0

let f(t) =∫_0 ^∞ e^(−arctsn( 1+tx^2 )) dx with t from R 1) calculate f^′ (t) 2) find a simple form of f(t) .

$${let}\:{f}\left({t}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:{e}^{−{arctsn}\left(\:\mathrm{1}+{tx}^{\mathrm{2}} \right)} {dx}\:\:{with}\:{t}\:{from}\:{R} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}^{'} \left({t}\right) \\ $$$$\left.\mathrm{2}\right)\:{find}\:{a}\:{simple}\:{form}\:{of}\:{f}\left({t}\right)\:. \\ $$

Question Number 35949 Answers: 1 Comments: 1

∫_0 ^( α) ((tan θ)/(√(a^2 cos^2 θ−b^2 sin^2 θ))) dθ = ?

$$\int_{\mathrm{0}} ^{\:\:\alpha} \frac{\mathrm{tan}\:\theta}{\sqrt{{a}^{\mathrm{2}} \mathrm{cos}\:^{\mathrm{2}} \theta−{b}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \theta}}\:{d}\theta\:=\:? \\ $$

Question Number 35920 Answers: 1 Comments: 1

∫ ((e^(2x) +1)/(2e^x −1)) dx = ?

$$\int\:\frac{{e}^{\mathrm{2}{x}} +\mathrm{1}}{\mathrm{2}{e}^{{x}} −\mathrm{1}}\:{dx}\:=\:? \\ $$

Question Number 35909 Answers: 1 Comments: 4

∫((7x−6)/((x^2 +25)(√((x−3)^2 +4)))) dx = ?

$$\int\frac{\mathrm{7}{x}−\mathrm{6}}{\left({x}^{\mathrm{2}} +\mathrm{25}\right)\sqrt{\left({x}−\mathrm{3}\right)^{\mathrm{2}} +\mathrm{4}}}\:{dx}\:=\:? \\ $$

Question Number 35832 Answers: 1 Comments: 3

find the value of f(λ) = ∫_(−a) ^a (dx/((λ +_ x^2 )^(3/2) )) λ∈R .

$${find}\:{the}\:{value}\:{of}\:\:{f}\left(\lambda\right)\:=\:\int_{−{a}} ^{{a}} \:\:\:\frac{{dx}}{\left(\lambda\:+_{} {x}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} } \\ $$$$\lambda\in{R}\:. \\ $$

Question Number 35821 Answers: 0 Comments: 4

let f(t) = ∫_0 ^∞ ((e^(−ax) −e^(−bx) )/x^2 ) e^(−tx^2 ) dx with t>0 1) calculate f^′ (t) 2)find a simple form of f(t) 3) find the value of ∫_0 ^∞ ((e^(−2x) −e^(−x) )/x^2 ) e^(−3x^2 ) dx

$${let}\:{f}\left({t}\right)\:=\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−{ax}} \:−{e}^{−{bx}} }{{x}^{\mathrm{2}} }\:{e}^{−{tx}^{\mathrm{2}} } {dx}\:\:\:{with}\:{t}>\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}^{'} \left({t}\right) \\ $$$$\left.\mathrm{2}\right){find}\:{a}\:{simple}\:{form}\:{of}\:{f}\left({t}\right) \\ $$$$\left.\mathrm{3}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−\mathrm{2}{x}} \:\:−{e}^{−{x}} }{{x}^{\mathrm{2}} }\:{e}^{−\mathrm{3}{x}^{\mathrm{2}} } {dx} \\ $$

Question Number 35732 Answers: 1 Comments: 1

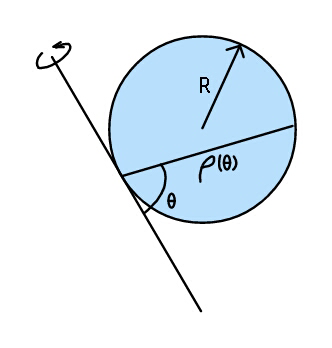
Question Number 35729 Answers: 1 Comments: 1

find the value of I =∫_0 ^π (dx/(2−cosx))

$${find}\:{the}\:{value}\:{of}\:\:\:{I}\:=\int_{\mathrm{0}} ^{\pi} \:\:\:\:\frac{{dx}}{\mathrm{2}−{cosx}} \\ $$

Question Number 35691 Answers: 0 Comments: 0

calculate lim_(a→0^+ ) ∫_(−a) ^a (√((1+x^2 )/(a^2 −x^2 ))) dx .

$${calculate}\:{lim}_{{a}\rightarrow\mathrm{0}^{+} \:\:\:\:} \:\:\:\int_{−{a}} ^{{a}} \:\:\sqrt{\frac{\mathrm{1}+{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} \:−{x}^{\mathrm{2}} }}\:\:{dx}\:. \\ $$

Question Number 35687 Answers: 1 Comments: 2

calculate f(a)=∫_0 ^π (dx/(1−a cosx)) a from R . 2) application calculate ∫_0 ^π (dx/(1−2cosx))

$${calculate}\:{f}\left({a}\right)=\int_{\mathrm{0}} ^{\pi} \:\:\:\:\:\frac{{dx}}{\mathrm{1}−{a}\:{cosx}}\:\:{a}\:{from}\:{R}\:. \\ $$$$\left.\mathrm{2}\right)\:{application}\:\:{calculate}\:\:\int_{\mathrm{0}} ^{\pi} \:\:\:\:\frac{{dx}}{\mathrm{1}−\mathrm{2}{cosx}} \\ $$

Question Number 35686 Answers: 1 Comments: 1

calculate ∫_(√3) ^(+∞) (dx/(x(√( 2+x^2 )))) .

$${calculate}\:\:\int_{\sqrt{\mathrm{3}}} ^{+\infty} \:\:\:\:\:\frac{{dx}}{{x}\sqrt{\:\mathrm{2}+{x}^{\mathrm{2}} }}\:. \\ $$

Question Number 35685 Answers: 1 Comments: 1

calculate ∫_0 ^(π/4) x artan(2x+1)dx

$${calculate}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:{x}\:{artan}\left(\mathrm{2}{x}+\mathrm{1}\right){dx} \\ $$

Question Number 35684 Answers: 1 Comments: 1

calculate I = ∫_0 ^1 e^(2t) ln(1+e^t )dt

$${calculate}\:{I}\:\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:{e}^{\mathrm{2}{t}} \:{ln}\left(\mathrm{1}+{e}^{{t}} \right){dt} \\ $$

Question Number 35683 Answers: 1 Comments: 1

find ∫ x^2 ln(x^6 −1)dx

$${find}\:\int\:\:{x}^{\mathrm{2}} {ln}\left({x}^{\mathrm{6}} −\mathrm{1}\right){dx} \\ $$

Question Number 35682 Answers: 1 Comments: 2

let F(x) = ∫_(x +1) ^(x^2 +1) arctan(1+t)dt 1) calculate (∂F/∂x)(x) 2) find lim_(x→0) F(x) .

$${let}\:{F}\left({x}\right)\:=\:\int_{{x}\:+\mathrm{1}} ^{{x}^{\mathrm{2}} \:+\mathrm{1}} \:\:\:{arctan}\left(\mathrm{1}+{t}\right){dt} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:\frac{\partial{F}}{\partial{x}}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:\:{find}\:{lim}_{{x}\rightarrow\mathrm{0}} \:{F}\left({x}\right)\:. \\ $$

Question Number 35681 Answers: 1 Comments: 1

find ∫ arctan(x)dx

$${find}\:\:\int\:{arctan}\left({x}\right){dx} \\ $$

Question Number 35680 Answers: 0 Comments: 0

by using residus theorem calculate W_n =∫_0 ^(π/2) cos^(2n) t dt ( wallis integal) n integr natural .

$${by}\:{using}\:{residus}\:{theorem}\:{calculate} \\ $$$${W}_{{n}} \:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:{cos}^{\mathrm{2}{n}} {t}\:{dt}\:\:\left(\:\:{wallis}\:{integal}\right)\:{n}\:{integr} \\ $$$${natural}\:. \\ $$

Question Number 35678 Answers: 0 Comments: 1

let f(t) =∫_0 ^∞ ((e^(−tx^2 ) arctan(x^2 ))/x^2 )dx with t>0 1) study the existencte of f(t) 2)calculate f^′ (t) 3)find a simple form of f(t).

$${let}\:{f}\left({t}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{e}^{−{tx}^{\mathrm{2}} } \:{arctan}\left({x}^{\mathrm{2}} \right)}{{x}^{\mathrm{2}} }{dx}\:{with}\:{t}>\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:{study}\:{the}\:{existencte}\:{of}\:{f}\left({t}\right) \\ $$$$\left.\mathrm{2}\right){calculate}\:{f}^{'} \left({t}\right) \\ $$$$\left.\mathrm{3}\right){find}\:{a}\:{simple}\:{form}\:{of}\:{f}\left({t}\right). \\ $$

Question Number 35677 Answers: 0 Comments: 2

find F(x)=∫_0 ^x e^(−2t) cos(t+(π/4))dx.

$${find}\:{F}\left({x}\right)=\int_{\mathrm{0}} ^{{x}} \:{e}^{−\mathrm{2}{t}} {cos}\left({t}+\frac{\pi}{\mathrm{4}}\right){dx}. \\ $$

Question Number 35676 Answers: 0 Comments: 1

find f(x)=∫_0 ^x ch^4 t dt

$${find}\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{{x}} \:{ch}^{\mathrm{4}} {t}\:{dt} \\ $$

Question Number 35675 Answers: 0 Comments: 1

calculate ∫_1 ^3 (x/(e^x −1))dx ..

$${calculate}\:\:\int_{\mathrm{1}} ^{\mathrm{3}} \:\:\:\frac{{x}}{{e}^{{x}} \:−\mathrm{1}}{dx}\:.. \\ $$

Question Number 35635 Answers: 1 Comments: 1

Question Number 35992 Answers: 0 Comments: 1

let f(x)= ((sin(2x))/x) χ_(]−a,a[) (x) with a>0 calculate the fourier trsnsform of f .

$${let}\:{f}\left({x}\right)=\:\frac{{sin}\left(\mathrm{2}{x}\right)}{{x}}\:\chi_{\left.\right]−{a},{a}\left[\right.} \left({x}\right)\:\:{with}\:{a}>\mathrm{0} \\ $$$${calculate}\:{the}\:{fourier}\:{trsnsform}\:{of}\:{f}\:. \\ $$

Question Number 35632 Answers: 0 Comments: 2

let ϕ(x)= (1/(√(a^2 −x^2 ))) if ∣x∣<a and ϕ(x)=0 if ∣x∣≥a find the fourier transform of ϕ .

$${let}\:\varphi\left({x}\right)=\:\frac{\mathrm{1}}{\sqrt{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }}\:\:{if}\:\mid{x}\mid<{a}\:\:{and}\:\varphi\left({x}\right)=\mathrm{0}\:{if}\:\mid{x}\mid\geqslant{a} \\ $$$${find}\:{the}\:{fourier}\:{transform}\:{of}\:\varphi\:. \\ $$

Question Number 35631 Answers: 0 Comments: 0

let U_n = ∫_0 ^∞ e^(−(t/n)) arctan(t)dt find a equivalent of U_n (n→+∞)

$${let}\:{U}_{{n}} =\:\int_{\mathrm{0}} ^{\infty} \:\:\:{e}^{−\frac{{t}}{{n}}} \:\:{arctan}\left({t}\right){dt} \\ $$$${find}\:{a}\:{equivalent}\:{of}\:{U}_{{n}} \:\:\left({n}\rightarrow+\infty\right) \\ $$

Question Number 35630 Answers: 0 Comments: 5

1) find the value of f(x)=∫_0 ^∞ ((1−cos(xt))/t^2 ) e^(−t) dt 2) calculate ∫_0 ^∞ ((1−cos(t))/t^2 ) e^(−t) dt .

$$\left.\mathrm{1}\right)\:{find}\:{the}\:{value}\:{of}\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}−{cos}\left({xt}\right)}{{t}^{\mathrm{2}} }\:{e}^{−{t}} {dt} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{1}−{cos}\left({t}\right)}{{t}^{\mathrm{2}} }\:{e}^{−{t}} \:{dt}\:. \\ $$

Pg 291 Pg 292 Pg 293 Pg 294 Pg 295 Pg 296 Pg 297 Pg 298 Pg 299 Pg 300

Contact: info@tinkutara.com